1, 4, 9, 16, 36, 49, 64 find the pattern
Answers
Answer:
0, 1, 4, 9, 16, 25, 36, 49, 64, 81, 100, 121, 144. Its a square number
Step-by-step explanation:
Hope this helps! Please mark brainliest if it did, it means a lot of proogression to me and thanks! <3
Answer:
Informally: When you multiply an integer (a “whole” number, positive, negative or zero) times itself, the resulting product is called a square number, or a perfect square or simply “a square.” So, 0, 1, 4, 9, 16, 25, 36, 49, 64, 81, 100, 121, 144, and so on, are all square numbers.
Step-by-step explanation:
Related Questions
what is the probability that after a fair coin is tossed 5 times that the result will contain 3 'heads' given that the first toss was a 'head' ?
Answers
Using Conditional probability,
The probability that after a fair coin is tossed 5 times that the result will contain 3 'heads' given that the first toss was a 'head' is 3/5.
A fair coin is tossed five times .
so, total possible outcomes = 2⁵ = 32
Let A: head observed on first toss
B: 3 heads observed.
Probability that the first toss was head (p) = 1/2
we have to find out probability that on 5 tosses result contain 3 heads given that first toss wss head .
Using Binomial Probability distribution
P (X= x) = ⁿCₓ (p)ˣ (q)⁽ⁿ⁻ˣ⁾
x = 3 , n = 5
we get , P(B) = P(X= 3 ) = ⁵C₃(1/2)³(1/2)²
=> P(B) = 10 ×(1/2)⁵= 5/16
since the first toss is fixed as a head.
P(A ∩ B) =P( X₁ = 1 and X₂+ X₃ + X₄ + X₅) = P(X₁= 1)P(X₂+X₃+X₄+X₅)
= 1/2 × ⁴C₂ (1/2)⁵ = 3 (1/2)⁴
The required probability is , Conditional probability P(B/A) = P(A ∩ B)/P(A)
= 3(1/2)⁴/ 5/16 = 3/5
Hence , the required probability is 3/5.
To learn more about Conditional Probability, refer:
https://brainly.com/question/10567654
#SPJ4
What are the 5 properties of division?
Answers
According to the division property of equality, if both sides of an equation are divided by a common real integer that is not zero, the quotients remain equal.
Commutative property, associative property, distributive property, and identity property are the four number properties. Only the algebraic operations addition, subtraction, multiplication, and division are linked with number characteristics.
The four key terminology used in the division process are dividend, divisor, quotient and remainder. Dividend Divisor = Quotient + Remainder is the formula for dividing two numbers.
Commutative property, associative property, distributive property, and identity property are the four number properties. Only the algebraic operations addition, subtraction, multiplication, and division are linked with number characteristics.
For more questions on Division
https://brainly.com/question/28119824
#SPJ4
What is sum and difference identities?
Answers
Sum and difference identities are formulas in trigonometry that allow us to express the trigonometric functions of the sum or difference of two angles in terms of the trigonometric functions of the individual angles.
The sum identities are:
sin(a + b) = sin(a)cos(b) + cos(a)sin(b)
cos(a + b) = cos(a)cos(b) - sin(a)sin(b)
tan(a + b) = (tan(a) + tan(b)) / (1 - tan(a)tan(b))
The difference identities are:
sin(a - b) = sin(a)cos(b) - cos(a)sin(b)
cos(a - b) = cos(a)cos(b) + sin(a)sin(b)
tan(a - b) = (tan(a) - tan(b)) / (1 + tan(a)tan(b))
For example, if we want to find the value of sin(45 + 30), we can use the sum identity for sine:
sin(45 + 30) = sin(45)cos(30) + cos(45)sin(30)
We know that sin(45) and cos(30) are both equal to √2/2, and that cos(45) and sin(30) are both equal to 1/2, so we can substitute these values into the formula and simplify:
sin(45 + 30) = (√2/2) x (1/2) + (√2/2) x (1/2)
= (√2 + 1)/2
Therefore, sin(45 + 30) = (√2 + 1)/2.
To learn more about Sum and difference please click on below link
https://brainly.com/question/29774937
#SPJ4
PLEASE HELP
a swimming pool is the shape of a rectangular prism. It holds 1440 cubic feet of water. If the base measures 15ft by 12ft, then how deep is the pool
Answers
Mr. Schaum used 10 buckets to collect rainfall in various locations on his property. Create a line plot using the number line provided to show the amount of rain collected in each bucket in gallons. Write an expression that includes multiplication to show how to find the total amount of water collected in gallons. Then, solve your expression.
Answers
Answer:
Step-by-step explanation:
the solution can be found in the attachment.

What is the surface area?
5 yd
6 yd
5 yd
5 yd
4 yd
Answers
Triangle ABC is shown.
b
Move expressions into the blanks to complete the proof of the Law of Cosines.
For A ABD, c² =
For A CBD, a²=
b2
c(cos A)
c²
and
Using substitution, this yields a² = b²-
Similarly, these steps can be used to solve for similar equations for b² and c², which yield the Law of Cosines.
h²
(x-b)²
c(cos B)
= (b² − 2bx+x²)+h².
-
=, which yields x =_
+
1²
h(cos A)
(b-x)²
h(cos B)
2bc(cos A)
cos A
cos B
2bc(cos B)

Answers
The triangles are used to prove the cosine rule which gives a²=b²-c²+2bcCosA
What is the cosine rule?In trigonometry, the Cosine Rule says that the square of the length of any side of a given triangle is equal to the sum of the squares of the length of the other sides minus twice the product of the other two sides multiplied by the cosine of angle included between them.
From the given parameters
For ΔABD, c²=x²+b² and SinB = x/c
Which yields that x=cCosb
For ΔCBD, a²= h² + (b -x )² = b²-2bx +x²) +h²
Using substitution this yields
a² = b² -c² + 2bc CosA
This gives the cosine rule from the two triangles
Learn more about the cosine rule on https://brainly.com/question/26952873
#SPJ1
how much does the 400-troy-ounce gold ingot weigh?
Answers
The answer is that a 400-troy-ounce gold ingot weighs approximately 12.4 kilograms or 27.34 pounds. This weight is equivalent to 3,110 grams or 3.11 kilograms. In summary, a 400-troy-ounce gold ingot weighs around 12.4 kilograms or 27.34 pounds and is equivalent to 3,110 grams or 3.11 kilograms.
The 400-troy-ounce gold ingot weighs, as the name suggests, 400 troy ounces. To provide some context, one troy ounce is equivalent to 31.1035 grams. Therefore, to determine the weight of the gold ingot in grams, you can perform the following calculation: 400 troy ounces x 31.1035 grams/troy ounce = 12,441.4 grams. In summary, the 400-troy-ounce gold ingot weighs 12,441.4 grams.
The weight of a 400-troy-ounce gold ingot can be calculated as follows: One troy ounce is equal to approximately 31.1 grams. The troy ounce is commonly used as a unit of weight for precious metals like gold. To find the weight of a 400-troy-ounce gold ingot, we multiply 400 by the weight of one troy ounce: 400 troy ounces * 31.1 grams per troy ounce = 12,440 grams. Since there are 1,000 grams in a kilogram, we can convert the weight from grams to kilograms:
12,440 grams / 1,000 grams per kilogram = 12.44 kilograms.
To know more about weight visit :-
https://brainly.com/question/12576008
#SPJ11
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP

Answers
Answer:
You can narrow it down to A or D because it is clear that football gets more attendance. There is typically a large distance between the 2, for example the first 1 has a distance of 80 and second a distance of 44. This means the answer is likely D, that football averages 50 more people in attendance
Step-by-step explanation:
In a half hour, Sarah is meeting her friends at the lake, 6 miles from her house. At what average speed must
she ride her bike to get there on time?
Answers
Answer:
Divide 6 miles by 0.5 hour: 6/0.5 = 12 miles per hour.
Step-by-step explanation:
The average speed must she rides her bike to get there on time will be 12 miles per hour.
What is speed?The distance covered by the particle or the body in an hour is called speed. It is a scalar quantity. It is the ratio of distance to time.
We know that the speed formula
Speed = Distance/Time
In a half-hour, Sarah is meeting her friends at the lake, 6 miles from her house.
The average speed must she rides her bike to get there on time will be
S = 6 / (1/2)
S = 6 x 2
S = 12 miles per hour
The average speed must she rides her bike to get there on time will be 12 miles per hour.
More about the speed link is given below.
https://brainly.com/question/7359669
#SPJ2
(laws of exponents with integer exponents lc) simplify (24)−1. one over two raised to the power of negative four one over two raised to the fourth power −24 23
Answers
By using the law of exponent the value of (24)⁻¹ is equal to 1/24.
To simplify (24)⁻¹, we can apply the rule for negative exponents.
The rule states that any non-zero number raised to the power of -n is equal to the reciprocal of that number raised to the power of n.
Applying this rule to (24)⁻¹, we have,
(24)⁻¹
= 1 / (24)¹
= 1 / 24
Therefore, applying the law of exponent (24)⁻¹ simplifies to 1/24.
Learn more about laws of exponent here
brainly.com/question/28834295
#SPJ4
The above question is incomplete, the complete question is:
Using laws of exponents with integer exponents ,simplify (24)⁻¹.
one over two raised to the power of negative four one over two raised to the fourth power .
What is the value of X?

Answers
Answer:
I think the answer for this question is 2.24cm
I think the bigger number would be 203 but I don't knowwww

Answers
Step-by-step explanation:
Let the number of psychology books be 'x'.
and that of sociology books be 'y'.
x + y = 317 (given)
Number of sociology books sold was 89 less than that of psychology books.
y = x - 89
Which means,
x + x - 89 = 317
2x = 317 + 89
2x = 406
x = 406/2 = 203
y = 203 - 89 = 114
Hence, 203 psychology books and 114 sociology books were sold.
diagram shows the position of a ship (s) and a marine base (b) the scale of the diagram is 1cm represents 20km
a) work out the real distance between the ship and the base
b)measuring the bearing of B from S
c) a fishing boat (f) is 20km from s on a bearing of 95 degrees

Answers
The real distance between the ship and the base is 35 km and the measure of the bearing of B from S would be; 70 degrees.
How to find the scale factor?Scale factor is the factor which is used to enlarge or smaller the original figure. Scale factor is the ratio of representation measurement of the figure to the original figures.
The figure represent the position of the ship(S) and the marine base(B).
a) Real distance between the ship and the base is;
When the distance from ship and the base is measured by rules. It comes out 3.5 cm.
The scale of the diagram is 1 cm, represents 10 km. Thus,
BS = 10/ 1 x 3.5
BS = 35 km
b) Measure the bearing of B from S
With the help of compass, the bearing angle of B from S is measured as the given;
Angle BSN = 70 degree
Thus, the real distance between the ship and the base is 35 km and the measure of the bearing of B from S would be; 70 degrees.
Learn more about the scale factor here;
brainly.com/question/10253650
#SPJ1
Which of the following inequalities represents all values of x for which the
quotient below is defined?

Answers
Answer: I think your answer is B!!
Step-by-step explanation: i might not be right but im pretty sure
is 25 3/8 bigger than 25 2/5
best answer = brainliest
Answers
Answer:
2/5 is greater than 3/8, so 25 2/5 is greater
Step-by-step explanation:
Find the least common denominator or LCM of the two denominators:
LCM of 5 and 8 is 40
Next, find the equivalent fraction of both fractional numbers with denominator 40
For the 1st fraction, since 5 × 8 = 40,
2/5
=
2 × 8
5 × 8
=
16/40
Likewise, for the 2nd fraction, since 8 × 5 = 40,
3/8
=
3 × 5
8 × 5
=
15/40
Since the denominators are now the same, the fraction with the bigger numerator is the greater fraction
16/40 > 15/ 40 or 2/5 > 3/8
the graph of the derivative of a function f crosses the x-axis 3 times. what does this tell you about the graph of f
Answers
Answer:
the graph of f has 3 turning points
Step-by-step explanation:
The graph of a function has a turning point (local extreme) where the derivative is zero and changes sign.
DerivativeThe derivative of a function tells you the slope of that function's graph. When the derivative is positive, the function is increasing. When the derivative is negative, the function is decreasing.
Turning PointWhere the derivative changes sign from positive to negative, the graph of the function changes direction from increasing to decreasing. At the point where the derivative is zero (between positive and negative), the graph is neither increasing nor decreasing. A tangent to the function at that point is a horizontal line, and the function itself is at a local maximum, a turning point.
The reverse is also true. When the derivative changes sign from negative to positive, the function changes from decreasing to increasing. The turning point where that occurs is a local minimum.
3 CrossingsIf the derivative crosses the x-axis (changes sign) 3 times, then there are three local extremes in the graph of f. The graph of f has 3 turning points.
__
Additional comment
In the attached graph, we have constructed a derivative function (red) that crosses the x-axis 3 times. It is the derivative of f(x), which is shown in blue. The purpose is to show the local extremes of f(x) match the zero crossings of the derivative.

The state of Colorado covers about 1.04 x 10^5 square miles. The Indian Ocean covers about 2.808 x 10^7 square miles. How many times bigger is the Indian Ocean than Colorado?
Answers
Answer:
27976000
Step-by-step explanation:
1.04 x 10^5=104000, and 2.808 x 10^7=28080000
28080000-104000=27976000
that's what I think, did this help
if a = b and b = c then a = c. true or false
Answers
Answer: true
Step-by-step explanation:
Answer: True
Step-by-step explanation: Has to be true if a b and c are all numbers
Graph the equation Y = X -1
Answers
Answer:sorry for the delate
Step-by-step explanation:
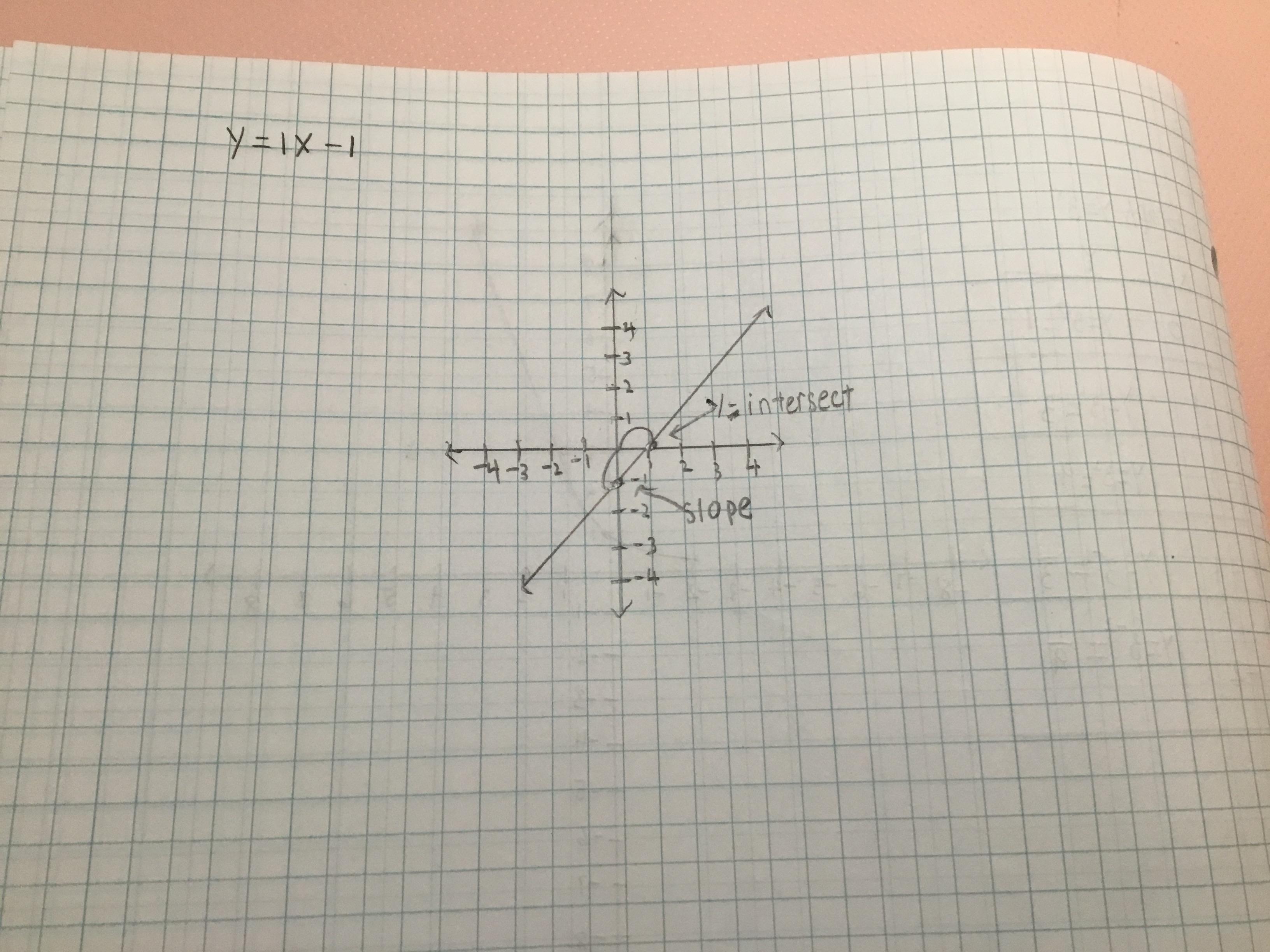
Simplifying
Y = x + -1
Reorder the terms:
Y = -1 + x
Solving
Y = -1 + x
Solving for variable 'Y'.
Move all terms containing Y to the left, all other terms to the right.
Simplifying
Y = -1 + x
3. The vertices of ABC are A(2, -1), B(0, -3), and C(4, -2). Find the vertices of the triangle after a translation 2 units right and 3 units down.
A. O A'YO, 2), B'(-2, 0), C'(2, 1)
B.O A-1, 1), B'(-3,-1), C(1,0)
C. O A'(4, -4), B (2, -6), C(6,-5)
D. O A'(4, 2), B (2,0), C'(6, 1)

Answers
Answer:
C
Step-by-step explanation:
|x+1|<4 please and thank youuuu
Answers
Answer:
-5,3
Step-by-step explanation:
Jeremiah is taking two of his friends out for pancakes. If he spends $17.55 on their meals and has $26 to spend in total, Jeremiah’s pancakes must cost no more than what amount?
Inequality Question
Answers
Answer:
$8.45
Step-by-step explanation:
if he has $26 to start and spends 17.55 on his friends, he has 8.45 leftover to spend on himself, but since he bought his friends pancakes for 17.55 their pancakes would have cost 8.775 each which suggests that Jeremiah likely cannot afford his own pancakes
In a geometric series, the sum of the third term and the fifth term is 295181. Three
consecutive terms of the same series are 179x, 21027x and 31381x. If x is equal to
the sixth term in the series, and the sum of the terms in the series is 419093072x,
find the number of terms in the series.
Answers
Therefore, the value of n, the number of terms within the geometric series, is around 104.804.n = 1 + log base 1.0241 (2341106.65 * 0.0241)
Geometric series calculation.Given:
Sum of the third term and the fifth term of the geometric series = 295181
Three consecutive terms: 179x, 21027x, and 31381x
Sum of all terms in the series = 419093072x
To find the number of terms in the series, we need to determine the common ratio (r) of the geometric series and then use it to calculate the number of terms.
Step 1: Find the common ratio (r)
The common ratio (r) can be found by dividing the second term by the first term or the third term by the second term. Let's use the first and second terms:
21027x / 179x = r
Simplifying:
r = 21027 / 179
Step 2: Find the value of x
From the given information, we know that x is equal to the sixth term in the series. Using the formula for the nth term of a geometric series, we can express the sixth term in terms of the first term and the common ratio:
sixth term = first term * (r(n-1))
Plugging in the values:
31381x = 179x * (r⁵)
Simplifying:
(r⁵)= 31381 / 179
Step 3: Find the number of terms
To find the number of terms, we need to determine the value of n in the sixth term formula. We can use the sum of all terms in the series and the formula for the sum of a geometric series:
Sum of all terms = first term * ((rn - 1) / (r - 1))
Plugging in the values:
419093072x = 179x * ((rn - 1) / (r - 1))
We can simplify this equation to:
((r(n - 1) / (r - 1)) = 419093072 / 179
Now, we have two equations:
r⁵ = 31381 / 179
((rn - 1) / (r - 1)) = 419093072 / 179
To solve for n, able to multiply both sides of the equation by 0.0241:
1.0241(n - 1 = 2341106.65 * 0.0241
Presently, we are able solve for n by taking the logarithm of both sides of the condition with base 1.0241:
log base 1.0241 (1.0241(n - 1) = log base 1.0241 (2341106.65 * 0.0241)
n - 1 = log base 1.0241 (2341106.65 * 0.0241)
To confine n, we include 1 to both sides of the equation:
n = 1 + log base 1.0241 (2341106.65 * 0.0241
n ≈ 104.804
Therefore, the value of n, the number of terms within the geometric series, is around 104.804.n = 1 + log base 1.0241 (2341106.65 * 0.0241)
Learn more about geometric series below.
https://brainly.com/question/24643676
#SPJ4
Therefore, the value of n, the number of terms within the geometric series, is around 104.804.n = 1 + log base 1.0241 (2341106.65 * 0.0241)
Given:
Sum of the third term and the fifth term of the geometric series = 295181
Three consecutive terms: 179x, 21027x, and 31381x
Sum of all terms in the series = 419093072x
To find the number of terms in the series, we need to determine the common ratio (r) of the geometric series and then use it to calculate the number of terms.
Step 1: Find the common ratio (r)
The common ratio (r) can be found by dividing the second term by the first term or the third term by the second term. Let's use the first and second terms:
21027x / 179x = r
Simplifying:
r = 21027 / 179
Step 2: Find the value of x
From the given information, we know that x is equal to the sixth term in the series. Using the formula for the nth term of a geometric series, we can express the sixth term in terms of the first term and the common ratio:
sixth term = first term * (r(n-1))
Plugging in the values:
31381x = 179x * (r⁵)
Simplifying:
(r⁵)= 31381 / 179
Step 3: Find the number of terms
To find the number of terms, we need to determine the value of n in the sixth term formula. We can use the sum of all terms in the series and the formula for the sum of a geometric series:
Sum of all terms = first term * ((rn - 1) / (r - 1))
Plugging in the values:
419093072x = 179x * ((rn - 1) / (r - 1))
We can simplify this equation to:
((r(n - 1) / (r - 1)) = 419093072 / 179
Now, we have two equations:
r⁵ = 31381 / 179
((rn - 1) / (r - 1)) = 419093072 / 179
To solve for n, able to multiply both sides of the equation by 0.0241:
1.0241(n - 1 = 2341106.65 * 0.0241
Presently, we are able solve for n by taking the logarithm of both sides of the condition with base 1.0241:
log base 1.0241 (1.0241(n - 1) = log base 1.0241 (2341106.65 * 0.0241)
n - 1 = log base 1.0241 (2341106.65 * 0.0241)
To confine n, we include 1 to both sides of the equation:
n = 1 + log base 1.0241 (2341106.65 * 0.0241
n ≈ 104.804
Therefore, the value of n, the number of terms within the geometric series, is around 104.804.n = 1 + log base 1.0241 (2341106.65 * 0.0241)
Learn more about geometric series from the given link:
brainly.com/question/24643676
#SPJ11
PLS HELP ME ASAP ILL GIVER BRAINliest

Answers
Answer:
Step-by-step explanation:
True or False? Justify your answer. Answers without correct justification will receive no credit. (I) A square matrix with the characteristic polynomial λ 4
−4λ 3
+2λ 2
−λ+3 is invertible. Justification: True □ False (II) Matrix [ 1
3
3
4
] in Z 5
is digonalizable. Justification: True False (III) Two matrices A and B are similar if and only if they similar to a same diagonal matrix. Justification: True □ False (IV) There exists a matrix A with eigenvalue 5 whose algebraic multiplicity is 2 and geometric multiplicity is 3 . Justification: True □ False (V) Two diagonal matrices D 1
and D 2
are similar if and only if D 1
=D 2
. Justification:
Previous question
Answers
The statements are marked as;
I. False
II. False
III. True
IV. False
V. True
How to determine the statementsTo prove the statements, we need to know the following;
The characteristic polynomial has a root = 0 in order to be an invertible matrix If and only if two matrices A and B share the same Jordan canonical form, they are comparable. The number of times an eigenvalue appears as a root of the characteristic polynomial is indicated by its algebraic multiplicity. If and only if two diagonal matrices D1 and D2 contain the same diagonal entries, they are comparable.Learn more about matrix at: https://brainly.com/question/94574
#SPJ4
Help me please I need the help right now plss

Answers
The fraction \($\frac{9}{12}$\) can be expressed as the sum of the smaller fraction \($\frac{3}{4}$\).
Ryan grew \($\frac{1}{2}$\) feet in a year.
1. To express the fraction \($\frac{9}{12}$\) as a sum of smaller fractions, we can simplify it by dividing both the numerator and denominator by their greatest common divisor. In this case, the greatest common divisor of \(9\) and \(12\) is \(3\).
So, we can rewrite \($\frac{9}{12}$\) as:
\($\frac{9}{12} = \frac{3 \times 3}{3 \times 4} = \frac{3}{4}$\)
Therefore, the fraction \($\frac{9}{12}$\) can be expressed as the sum of the smaller fraction \($\frac{3}{4}$\).
2. To find how much Ryan grew in a year, we need to calculate the difference between his height this year and his height last year.
Let's convert the mixed fractions to improper fractions for easier computation:
Last year's height: \($4 \frac{3}{4} = \frac{4 \times 4 + 3}{4} = \frac{19}{4}feet\)
This year's height: \($5 \frac{1}{4} = \frac{5 \times 4 + 1}{4} = \frac{21}{4} feet\)
To determine the growth, we subtract last year's height from this year's height:
\($\frac{21}{4} - \frac{19}{4} = \frac{21 - 19}{4} = \frac{2}{4} = \frac{1}{2}feet\)
Therefore, Ryan grew \($\frac{1}{2}$\) feet in a year.
For more such questions on fraction: https://brainly.com/question/17220365
#SPJ11
Neal has a $15 gift card for music downloads. Each song costs $1 to download. The amount of money left on the card, in dollars, can be represented by the function f(x)=15−x, where x is the number of downloaded songs. Which of the following statements are true for the given function? (Choose all that apply).
a. f(1) = 14 means Neal downloaded 1 song and has $14 left on his gift card.
b. f(2) = 15 means Neal downloaded 2 songs and has $15 left on his gift card.
c. You could represent Neal downloaded 12 songs and has $3 left on his gift card with f(12)=3.
d. f(15)=0 represents that Neal has $15 on his gift card before he downloads any songs.
Y’all i’m really struggling with this, and it’s due tonight
Answers
Answer: a. b. and c.
Step-by-step explanation:
a.- (f)1=14 is correct in this explanation because 15-
1=14
b.- f(2)=15 is incorrect. 15-2 does not equal 15. It would be 13.
=0
c.- f(12)=3 is correct because 15-12=3
d.- f(15)=0 is correct because 15-5=
i'll give brainliest

Answers
Answer:
its d
Step-by-step explanation:
write the equation of the line in slope-int form that is perpendicular to y=-x - 18 and passes through (-6,11)
Answers
Answer:
y = x + 17
Step-by-step explanation:
the equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
y = - x - 18 ← is in slope- intercept form
with slope m = - 1
given a line with slope m then the slope of a line perpendicular to it is
\(m_{perpendicular}\) = - \(\frac{1}{m}\) = - \(\frac{1}{-1}\) = 1 , then
y = x + c ← is the partial equation of the perpendicular line
to find c substitute (- 6, 11 ) into the partial equation
11 = - 6 + c ⇒ c = 11 + 6 = 17
y = x + 17 ← equation of perpendicular line