A rectangular prism with a volume of 5x^3 +14x^2+8x cubic units has a base area of x^2 c2x square units. Find the height of the rectangular prism
Answers
The height of the rectangular prism is given by the expression \(5x + 14 + 8/x\).
To find the height of the rectangular prismIts volume must be divided by the size of its base.
Given:
Volume of the rectangular prism \(= 5x^3 + 14x^2 + 8x cubic units\)
Base area of the rectangular prism =\(x^2 square units\)
Height of the rectangular prism is determined by its volume divided by its base area.
Plugging in the given values, we have:
Height =\((5x^3 + 14x^2 + 8x) / (x^2)\)
To simplify the expression, we can divide each term in the numerator by \(x^2\):
Height =\((5x^3/x^2 + 14x^2/x^2 + 8x/x^2)\)
= \((5x + 14 + 8/x)\)
Therefore, the height of the rectangular prism is given by the expression \(5x + 14 + 8/x.\)
Learn more about rectangular prism here : brainly.com/question/17929725
#SPJ1
Related Questions
what is 36.12 divided by 0.42 show your work
Answers
Answer: 86
Step-by-step explanation:
calculator but..
\(\frac{36.12}{0.42}\) just convert them into whole numbers and after finding the value put the same number of spaces in the decimal behind it. Did that make sense?
Write a simplified expression for the perimeter of the rectangle

Answers
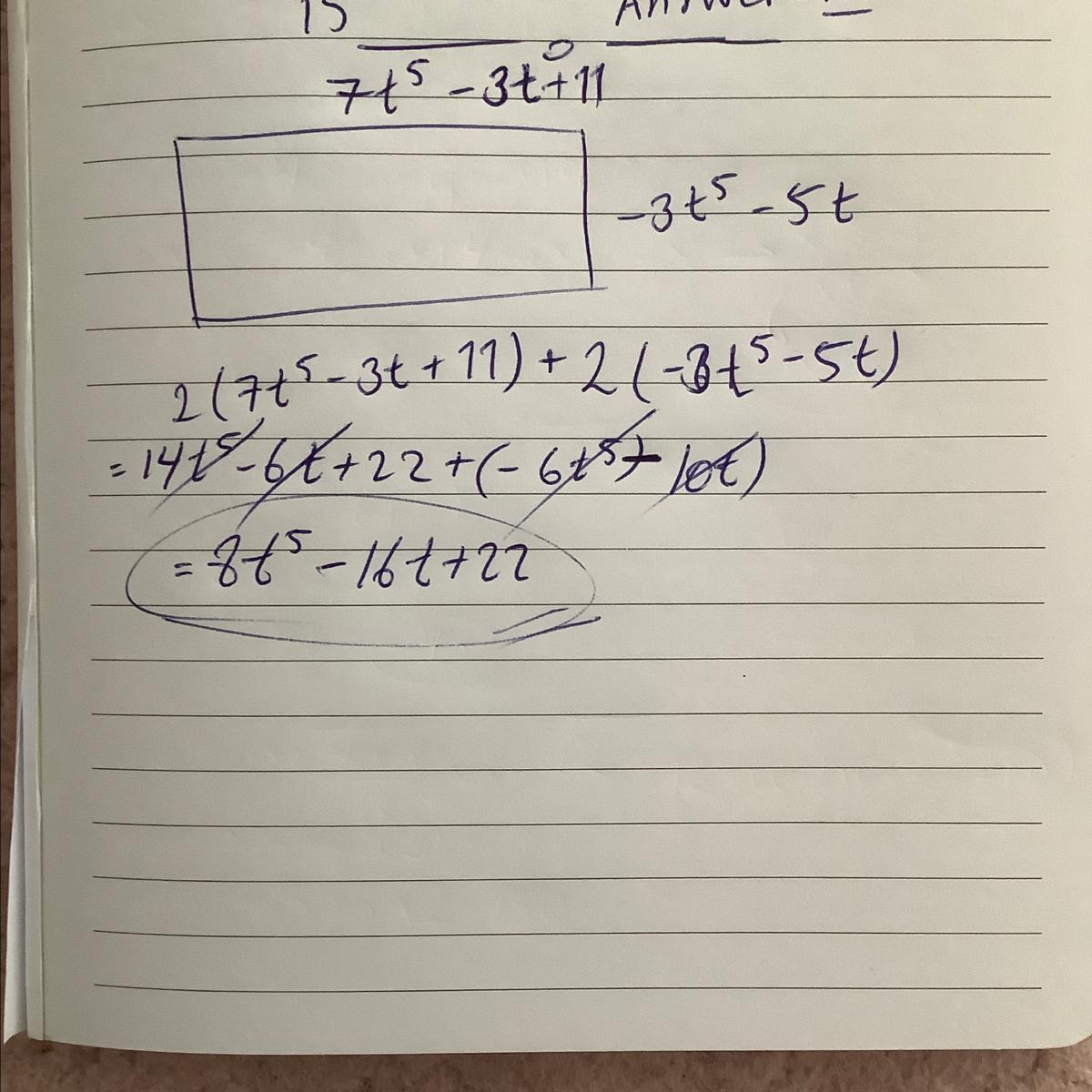
what’s the answer help
asap & make sure the answers right !

Answers
Answer: 169.56 or 170 units^3
Step-by-step explanation:
Volume of a cylinder
3.14(3)^2=28.26*6=169.56
Answer:
54π
Step-by-step explanation:
V = πr^2 h
V = π 3*3*6
V = 54π or 169.56
Consider the parabola y = 4x - x2. Find the slope of the tangent line to the parabola at the point (1, 3). Find an equation of the tangent line in part (a).
Answers
The given parabolic equation is y = 4x - x² and the point is (1, 3). We are to determine the slope of the tangent line at (1, 3) and then obtain an equation of the tangent line. we must first calculate the derivative of the given equation.
We can do this by using the power rule of differentiation. The derivative of x² is 2x. So the derivative of y = 4x - x² is dy/dx = 4 - 2x.Since we want to find the slope of the tangent line at (1, 3), we need to substitute x = 1 into the equation we just obtained. dy/dx = 4 - 2x = 4 - 2(1) = 2. Therefore, the slope of the tangent line at (1, 3) is 2.We can now write the equation of the tangent line. We know the slope of the tangent line, m = 2, and we know the point (1, 3).
We can use the point-slope form of the equation of a line to obtain the equation of the tangent line. The point-slope form of the equation of a line is given as: y - y₁ = m(x - x₁)where m is the slope, (x₁, y₁) is a point on the line.Substituting in the values we have, we get:y - 3 = 2(x - 1)We can expand this equation to obtain the slope-intercept form of the equation of the tangent line:y = 2x + 1Therefore, the equation of the tangent line to the parabola y = 4x - x² at the point (1, 3) is y = 2x + 1.
To Know about intercept visit:
brainly.com/question/14180189
#SPJ11
-2x - 6y = -24
X-8y = -10
Answers
Answer:
x = -26 and y = -2
I hope this is the answer you are looking for
Step-by-step explanation:
x-8y = -10 => add 8y to both sides
+8y = +8y
x = -10+8y
-2(-10+8y) -6y = -24 => intersect what you got into the first equation
20-16y-6y = -24 => Distribute the -2
20-22y = -24 => subtract 20 in both sides
-20 = -20
-22y = -44 => divided by -22 in both sides
/-22 = /-22
y = -2
x-8(-2) =-10 => intersect the y into the other equation
x+16 = -10 => multiply -8*-2
-16 = -16
x = -26 => subtract 16 in both sides.
Last week salazar played 13 more tennis games than perry. if they played a combined total of 53 games. How many games did salazar play?
Answers
If last week Salazar played 13 more tennis games than Perry and they played a combined total of 53 games, then Salazar played a total of 33 games.
Let the total number of games played by Perry be x.
It is given that, Salazar played 13 more tennis games than Perry.
⇒ Total games played by Salazar = x + 13
Also, Salazar and Perry played a combined of 53 games.
Hence, total number of tennis games played by Salazar and Perry = 53
⇒ Games played by Salazar + Games played by Perry = 53
⇒ x + (x + 13) = 53
2x + 13 = 53
2x = 53 - 13
2x = 40
x = 40 / 2
x = 20
Therefore, total number of games played by Salazar = x+13
= 20 + 13
= 33
Thus, Salazar played total 33 games.
Learn more on total here:
https://brainly.com/question/14306809
#SPJ1
Divide 8x³+x²-32x-4 by x2-4.
OA. 8x² +33x+100+
OB. 8x²-31x+156-.
O C. 8x-1
OD. 8x+1
396
x² - 4
620
-4
Answers
The correct answer is OD. 8x + 1.
To divide 8x³ + x² - 32x - 4 by x² - 4, we can use polynomial long division.
The dividend is 8x³ + x² - 32x - 4, and the divisor is x² - 4.
We start by dividing the highest degree term, which is 8x³, by x². This gives us 8x.
Next, we multiply the divisor x² - 4 by the quotient 8x. The result is 8x³ - 32x.
Subtracting 8x³ - 32x from the dividend, we get x² - 32x.
Now, we divide x² - 32x by x² - 4. This gives us 1.
Multiplying the divisor x² - 4 by the quotient 1, we get x² - 4.
Subtracting x² - 4 from the remaining dividend, which is -32x, we get -32x + 4.
Since we can no longer divide, the final result is the quotient we obtained: 8x + 1.
Therefore, the correct answer is:
OD. 8x + 1.
for such more question on polynomial
https://brainly.com/question/15702527
#SPJ8
A rectangular price tag has a perimeter of 24 centimeters. Its area is 27 square centimeters. What are the dimensions of the price tag?
Answers
Perimeter is the sides added up
3*2+9*2=24
And area is b*h
9*3=27
Determine the x- and y-intercepts of the graph of x + 3y = 9.
Then plot the intercepts to graph the equation.
Line
Answers
x intercept (9, 0) and y intercept (0, 3)
Equation in y intercept form is
y = -1/3x + 3
Step by step
x + 3y = 9
Change this into y intercept form
3y = -1x + 9
Divide both sides by 3 to isolate y
3/3 y = -1/3x + 9/3
y = -1/3x + 3 is your equation
Your points to graph
Using your original equation
x + 3y = 9
Substitute 0 for x and solve for y intercept
0 +3y = 9
Divide both sides by 3
Y = 3
Substitute 0 for y and solve for x intercept
X + (3)(0) = 9
X = 9
You now have points to graph
(9, 0) and (0, 3)
Graph is attached

Water is rushing down the slide at a rate of 1 half gallon every 3 seconds .At this rate , how many gallons of water rush down the slide each seconds ?
Answers
1/2 gallons of water rush down the slide each seconds.
Explain the method of unitary?The unitary approach involves calculating the value of a specific unit, from which we can calculate the values of the necessary number of units.The unitary method's formula is to identify the value of a specific unit, then multiply that value by the number of units to obtain the required value.For the given question-
Rate of running water = 1 half gallon every 3 seconds.
Rate of running water = 1 1/2 = 3/2 gallon every 3 seconds.
It means-
In every 3 seconds ----> 3/2 gallons water flows.
Thus for 1 sec, divide both side by 3.
1 seconds ----> 1/2 gallons water flows.
Therefore, 1/2 gallons of water rush down the slide each seconds.
To know more about the method of unitary, here
https://brainly.com/question/24587372
#SPJ1
Quadrilateral STUV is a rectangle, SU=z+53, and TV=2z. What is the value of z?TSVURz=Submit

Answers
Recall that the diagonals of a rectangle are congruent. Therefore, we can set the following equation:
\(z+53=2z.\)Solving the above equation for z, we get:
\(\begin{gathered} 2z-z=53, \\ z=53. \end{gathered}\)Answer:\(z=53.\)in a between-subjects 2x4 factorial design with 30 participants randomly assigned to each factorial combination, how many total participants are required?
Answers
A total of 240 participants are required for the study.
In a between-subjects factorial design, researchers manipulate two or more independent variables to examine their effects on the dependent variable. In this design, participants are randomly assigned to one of the treatment groups formed by the combination of the levels of the independent variables.
In a 2x4 factorial design, there are two independent variables, each with two levels. The first independent variable has two levels, and the second independent variable has four levels. Therefore, there are eight possible combinations of the independent variables, andbare assigned to each of these combinations.
To calculate the total number of participants required for the study, we simply multiply the number of participants per combination by the total number of combinations. Number of levels for the first independent variable (2) multiplied by the number of levels for the second independent variable (4) multiplied by the number of participants per cell (30).
In this case, we have:
30 participants per combination x 8 combinations = 240 total participants
So the total number of participants required is:
2 x 4 x 30 = 240 participants.
To learn more about factorial combination refer below:
https://brainly.com/question/15325293
#SPJ11
Solve: -8 = -7 + X x =
Answers
-8 = -7 + X
- 8 + 7 = X (Adding 7 to both sides of the equation)
-1 =x (Subtracting)
The answer is x=-1.
the answer of -8 = -7 +Xx is x= -1
Javier is comparing costs of two cars. Car A costs $130 per month for insurance and $0.10 per mile to run. Car B costs $108 per month for insurance and $0.15 per mile to run. He defines x as the number of miles driven each month and y as the total cost to run the car. Which set of equations can be used to find the number of miles Javier would need to drive to make the costs equal?
0.10 x = 130. 0.15 y = 108
y = 0.10 x + 130. y = 0.15 x + 108
0.10 x = 108. 0.15 y = 130
y = 0.15 x + 130. y = 0.10 x + 108
Answers
Answer:
y = 0.10 x + 130. y = 0.15 x + 108
Step-by-step explanation:
0.10 and 0.15 are the slope in the equation y = mx + b since it requires an input (number of miles) to spit out an output (number of miles*0.10 = price) and the x intercept is 130 and 108 creating the equation y = 0.10 x + 130. y = 0.15 x + 108.
What is the inverse of the function f(x) = 2x + 1?
h(x) = one-halfx – one-half
h(x) = one-halfx + one-half
h(x) = one-halfx – 2
h(x) = one-halfx + 2
f(–2) = g(–2)
f(0) = g(–2)
f(–2) = g(0)
Answers
The inverse of the function f(x) = 2x+1 is (x-1)/2.
What is inverse of function?The output of a function is returned by the inverse function, which also returns the initial value. Consider the inverse relationship between the functions f and g: f(g(x)) = g(f(x)) = x. The initial value is fetched via a function that is its inverse.
Given;
Function, f(x) = 2x + 1.
To find the inverse of the function:
First, let y = f(x)
That is,
y = 2x +1
Then, make x the subject of the equation
y = 2x + 1
To make x the subject of this equation:
First, subtract 1 from both sides
We get
y -1 = 2x
Now, divide both sides by 2.
x = (y-1)/2
Now, rewrite as f⁻¹(x) by replacing y by x.
That is,
f⁻¹(x) = (x-1)/2
Therefore, the inverse of the function f(x) is (x-1)/2.
To learn more about the inverse of function;
https://brainly.com/question/17872426
#SPJ1
what percent of 56.25$ is 11.25$
Answers
Answer:
3%percent of $375 is $11.25 and 20% of $56.25 is $11.25 .
Step-by-step explanation:
A sample of 40 provided a sample mean of 26.2. the population standard deviation is 6. Find the value of the test statistic. (round your answer to two decimal places.) (b) find the p-value. (round your answer to four decimal places.) p-value
Answers
Answer:
a. 1.26
b. 0.1038
Step-by-step explanation:
The p-value is 1.0000 (rounded to four decimal places). This means that we fail to reject the null hypothesis, which in this case would be that the population mean is equal to the sample mean of 26.2.
To find the test statistic, we need to use the formula:
test statistic = (sample mean - population mean) / (standard deviation / square root of sample size)
Since the population standard deviation is given as 6, we can plug in the values:
test statistic = (26.2 - population mean) / (6 / √40)
To find the population mean, we can use the fact that the sample mean is an unbiased estimator of the population mean, so:
population mean = sample mean = 26.2
Plugging in the values, we get:
test statistic = (26.2 - 26.2) / (6 / √40) = 0
The test statistic is 0.
To find the p-value, we need to use a t-distribution table or calculator. Since we have a sample size of 40, we can use the t-distribution with 39 degrees of freedom.
Using a calculator or table, we can find that the area to the right of 0 (since the test statistic is 0) is 0.5. Since this is a two-tailed test, we need to double this value to get the p-value:
p-value = 2 * 0.5 = 1.0000
Learn more about test statistic here: brainly.com/question/14128303
#SPJ11
whats the value of 4/5 x 3/4 in simplest form
Answers
The value of 4/5 x 3/4 in simplest form is 3/5. To find the value of 4/5 x 3/4, we need to multiply the numerators and denominators separately and simplify the resulting fraction to its simplest form. Multiplying the numerators 4 and 3 gives us 12, while multiplying the denominators 5 and 4 gives us 20. So, 4/5 x 3/4 can be written as 12/20.
To simplify 12/20 to its simplest form, we need to find the greatest common factor (GCF) of the numerator and denominator. In this case, both 12 and 20 are divisible by 4, so the GCF is 4. Dividing both the numerator and denominator by 4, we get 3/5. Therefore, the value of 4/5 x 3/4 in simplest form is 3/5.
In conclusion, the value of 4/5 x 3/4 in simplest form is 3/5, which can be obtained by multiplying the numerators and denominators separately and simplifying the resulting fraction by finding its greatest common factor.
To know more about simplest form visit :
https://brainly.com/question/290068
#SPJ11
When light bounces off a rough surface, in different directions, what is this called? (2 points)
a
Interaction
b
Movement
c
Property
d
Reflection
Answers
assume x and y are functions of t. evaluate for the following. y^3=2x^4 + 81 dx/dt = 4, x = 5, y = 11
dy/dt = ____
Answers
To find dy/dt, we can differentiate the given equation y^3 = 2x^4 + 81 with respect to t using implicit differentiation.
Differentiating both sides of the equation with respect to t, we get:
3y^2 * dy/dt = 8x^3 * dx/dt
Substituting the given values dx/dt = 4, x = 5, and y = 11 into the equation, we can solve for dy/dt:
3(11)^2 * dy/dt = 8(5)^3 * 4
363 * dy/dt = 8 * 125 * 4
363 * dy/dt = 4000
Dividing both sides by 363, we find:
dy/dt = 4000 / 363
Simplifying this expression, we get:
dy/dt = 400/33
Therefore, the value of dy/dt is 400/33.
Learn more about equation from
https://brainly.com/question/17145398
#SPJ11
I have to find an equation for a table

Answers
Let's say imput is i and that output is o.
The formula is 2 ^ i = o
2^0 = 1
2^1 = 2
2^2 = 4
2^3 = 8
2^4 = 16
2^5 = 32
Notice how the numbers match?
Hope this helped :)
Today the population of a city is 250,000 and is growing at a rate
of 4% per year. When or how many years will the population reach
850,000
Answers
Step-by-step explanation:
Principal = 250,000
Rate = 4%
Simple interest = 850,000
Time = ?
\(t = \frac{100 \times interest}{principal \times rate} \\ t = \frac{100 \times 850000}{250000 \times 4} \\ t = \frac{100 \times 85}{25 \times 4} \\ t = \frac{8500}{100} \\ t = 85years\)
Two friends, Annabelle and Austin, took summer jobs. The equation y=16.9xy=16.9x represents Austin's earnings in dollars and cents, yy, for working xx hours. The table below represents Annabelle's earnings in dollars and cents, yy, for working xx hours.
Answers
The proportional relationship is given by y = 16.9x
Two variables are said to be proportion if an increase/decrease in one variable causes an increase/decrease in the other variable.
Let x represent the hours and y represent the earnings in dollars.
Since x and y are proportional, hence:
y ∝ x
y = kx
Where k is the constant of proportionality.
Since from the equation, k = 16.9m hence, the relationship is given by:
y = 16.9x
The proportional relationship is given by y = 16.9x
Find out more at: https://brainly.com/question/17178834
HELPPP PLEASEEEEE ON NUMBER 7!!!!!!

Answers
Answer:
D is the answer since you added all the numbers together ten divide by five
Answer:
D
Step-by-step explanation:
We need to find the missing value to make sure it averages out to 250.
So they will be 5 rounds of different scores.
Since we dealing with averages, we need to set up a
equalivent equation to 250. We would add up all our previous scores and try to find the new x score and divide it by 5 and set it equal to 250. So our equation will look like.
Let x represent of our next game.
\( \frac{236 + 274 + 246 + 236 + x}{5} = 250\)
Multiply by 5 both sides
\(236 + 274 + 246 +236 + x = 1250\)
\(992 + x = 1250\)
\(x = 258\)
d is the answer
PLS HELP!!!!!!Which of the following tables represents the relationship between the circumference and diameter of a
circle?

Answers
Answer:
itanong mo kay kapatid na arlene
It is D. If you multiply D by 3.14 and it matches the C, it is the right one. So it is D
In circle Q with the measure of minor arc ⌢ = 6 0 ∘ , PR ⌢ =60 ∘ , find m ∠ P S R . m∠PSR
Answers
Answer:n circle Q with the measure of minor arc ⌢ = 6 0 ∘ , PR ⌢ =60 ∘ , find m ∠ P S R . m∠PSR - 16846452.
Step-by-step explanation:mark me as brainest please
PLEASE HELP NOW!!!!!!!!!!!!!!!!!

Answers
The total number of ounces of other ingredients in her soup will be 16 ounces.
What is an expression?Expression in maths is defined as the collection of the numbers variables and functions by using signs like addition, subtraction, multiplication, and division.
Given that:-
9 people are served with 6 ounces of soup.Water is 4.75 ounces and there are 8 ounces in each cup.The total quantity of soup = 9 x 6 = 54 ounces
The total quantity of water = 4.75 x 8 = 38
The other ingredients are = 54 - 38 = 16 ounces
Therefore total number of ounces of other ingredients in her soup will be 16 ounces.
To know more about expression follow
https://brainly.com/question/723406
#SPJ1
The following cone has a slant height of 17
cm and a radius of 8
cm.
What is the volume of the cone?
Responses
480π
320π
544π
Answers
The formula for the volume of a cone is:
V = (1/3)πr²h
where r is the radius of the base, h is the height of the cone, and π is pi.
In this case, the slant height is given as 17 cm, which we can use with the radius to find the height of the cone using the Pythagorean theorem:
h² = s² - r²
h² = 17² - 8²
h² = 225
h = 15
Now that we have the height, we can plug in the values for r and h into the formula for the volume:
V = (1/3)π(8²)(15)
V = (1/3)π(64)(15)
V = (1/3)(960π)
V = 320π
Therefore, the volume of the cone is 320π cubic cm. Answer: 320π.
Find the equation of this line (No need to show work, be fast please.)

Answers
We are given the graph of a line and asked to find its equation. Recall that we can write the equation of a line as
\(y\text{ - y\_0=m\lparen x - x\_0\rparen}\)where (x0, y0) is point on the line and m is the slope of the line.
From the graph, we can identify that points (-3, -2) and (5,4) are on the line. Now let us calculate the slope of the line.
Recall that given points (a,b) and (c,d), the slope of the line that passes through them is given by the formula
\(m=\frac{d\text{ -b}}{c\text{ -a}}=\frac{b\text{ -d}}{a\text{ - c}}\)In our case we got a=-3, b=-2, c=5 and d=4. So we have
\(m\text{ = }\frac{4\text{ - \lparen-2\rparen}}{5\text{ - \lparen-3\rparen}}=\frac{4+2}{5+3}=\frac{6}{8}=\frac{3}{4}\)Now, we can use the fact that the point (5,4) is on the line. So we use it as our (x0,y0). So our equation would be
\(y\text{ - 4=}\frac{3}{4}(x\text{ - 5\rparen}\)which is equivalent to
\(y\text{ - 4=}\frac{3}{4}x\text{ - }\frac{15}{4}\)Now, we add 4 on both sides, so we get
\(\begin{gathered} y=\frac{3}{4}x\text{ -}\frac{15}{4}+4=\frac{3}{4}x\text{ -}\frac{15}{4}+\frac{16}{4} \\ =\frac{3}{4}x+\frac{1}{4} \end{gathered}\)so the equation of the line is
\(y=\frac{3}{4}x+\frac{1}{4}\)Given that segment AB is parallel to segment DE,
AB = 38.5, DE = 11, AC = 3x + 8, and CE = x + 2, write and proportion and solve for x, then find AC.
Answers
The value of x is -1, and AC is 5, given AB=38.5, DE=11, AC=3x+8, and CE=x+2 where AB is parallel to DE.
Since AB is parallel to DE, then triangles ABC and CDE are similar, so we can set up a proportion of their corresponding sides:
AC/CE = AB/DE
Substituting the given values, we get:
(3x + 8)/(x + 2) = 38.5/11
Cross-multiplying, we get:
11(3x + 8) = 38.5(x + 2)
Expanding the multiplication, we get:
33x + 88 = 38.5x + 77
Subtracting 33x and 77 from both sides, we get:
11x = -11
Dividing by 11, we get:
x = -1
Substituting this value of x back into the expression for AC, we get:
AC = 3x + 8 = 3(-1) + 8 = 5
Therefore, AC = 5.
we used the fact that if two triangles are similar, then the corresponding sides are proportional. This means that the ratio of the lengths of the corresponding sides is the same for both triangles.
In the second step, we substituted the given values into the proportion, which allowed us to create an equation with one variable, x.
In the third step, we solved for x by simplifying the equation and isolating x. Once we found the value of x, we substituted it back into the given equation to find the length of AC.
Learn more about corresponding sides here:
https://brainly.com/question/16086091
#SPJ1