Can someone please solve this before my mom takes away my phone?

Answer:

Chart:

x           y

-6         11

3           5

15         -3

-12        15

Step-by-step explanation:

The only things you can plug in are the domain {-12, -6, 3, 15}

Plug in the domain into equation to find y.

-6 :

y = -2/3 (-6) +7

y = +47

y=11

(-6,11)

3:

y = -2/3 (3) +7

y = -2 +7

y = 5

(3, 5)

15:

y = -2/3 (15) +7

y =  -10 +7

y = -3

(15 , -3)

-12:

y = -2/3 (-12) +7

y = 8 + 7

y= 15

(-12,15)

Answer:

1) 11

2) 3

3) -3

4) -12

Step-by-step explanation:

eq(1):

\(y = \frac{-2}{3} x + 7\\\\y - 7 = \frac{-2}{3} x\\\\x = (y - 7)\frac{-3}{2} \\\\x = (7-y)\frac{3}{2} ---eq(2)\)

1) x = -6

sub in eq(1)

\(y = \frac{-2}{3} (-6) + 7\\\\y = \frac{12}{3} + 7\\\\y = 4+7\\\\y = 11\)

2) y = 5

sub in eq(2)

\(x = (7-5)\frac{3}{2} \\\\x = 3\)

3) x = 15

sub in eq(1)

\(y = \frac{-2}{3} 15 + 7\\\\y = \frac{-30}{3} +7\\\\y = -10 + 7\\\\y = -3\)

4)

sub in eq(2)

\(x = (7-15)\frac{3}{2} \\\\x = -8\frac{3}{2}\\ \\x = -12\)

Answer:

|−5| < |−6|

Step-by-step explanation:

The symbols around the numbers signify that you must switch the negative to a positive or vice versa. So, in this case, this equation is actually 5 < 6.

The time that the first train would get to Oakengats is 08:15.

The time of arrival of the first train can be gotten from the table.

The first entry has a blank space. This would have been the time of arrival for the train.

After the blank space, the next time is when the fits train got to this destination. That is at 08:15

Read more on train arrivals here:

https://brainly.com/question/21654749?referrer=searchResults

#SPJ1

Answer:

a. 44 cm b. 126 cm

Step-by-step explanation:

a. it is semi circle

perimeter is πd

22/7*14

44 cm

b. it is equilateral triangle

so perimeter is side* 3

42 *3 = 126cm

Answer:

Step-by-step explanation:

(a)

The figure perimeter it is half of a circle with a diameter d₁=14 cm and two halfs of circle with a diameter d₂=14 cm÷2=7 cm.

The length of a circle is  L₀ = πd, where d is the diameter.

Therefore:

\(\bold{L=\frac12d_1+2\cdot\frac12d_2 = \frac12\cdot14\pi+\frac12\cdot2\cdot7\pi=14\pi\,cm\approx44\,cm}\)

(b)

A full circle it is 360°, so the circle sector of 60° is  \(\frac{60^o}{360^o}=\frac16\)  of the circle. So the arc of 60° is ¹/₆ of the full circle length.

The figure is an equilateral triangle and two 60°-sectors of circles with radiuses of length of the triangle's side (r=42 cm).

Its perimetr it's two radiuses, two arcs of 60° and one side of the triangle.

The length of a circle is  L₀ = 2πr, where r is a radius.

Therefore:

\(\bold{L=2r+2\cdot\frac16\cdot 2\pi r+r=3r+\frac23\pi r}\\\\\bold{L=3\cdot42+\frac23\pi\cdot42=(126+28\pi)\,cm\approx214\,cm}\)

Answer:

18, the answer to 8+10 is 18, like you learn in 1st grade

Answer:

18 the answer

hope it helps

f(x) and g(x) are not closed under subtraction because when subtracted, the result will be a polynomial, the correct option is B.

A polynomial is a mathematical equation that solely uses the operations addition, subtraction, multiplication, and non-negative integer exponentiation of variables. Variables are sometimes known as indeterminate in mathematics. Majorly used polynomials are binomial and trinomial.

Given f(x) and  g(x) two polynomial functions in the standard form of the polynomial,

According to Closure Property, when something is closed, the output will be the same as the input.

The polynomials f(x) and g(x) can be seen in the image.

On subtracting the two polynomials, the output will be a polynomial and so it is closed under subtraction.

Therefore, The reason why f(x) and g(x) are not closed under subtraction is that the outcome of subtraction will be a polynomial, making option B the best choice.

Learn more about Polynomials here:

https://brainly.com/question/11536910

#SPJ1

Complete question:

Answer: 6 inches.

Step-by-step explanation:

In probability plotting, a graph of the cumulative distribution function (CDF) is plotted against a transformed version of the data to determine if the data come from a particular distribution.

The distance between the two lines is equal to the product of the critical value of the t-distribution and the standard error estimate.

This can be used to analyze the times to failure (i.e., loss of acceptable sharpness) for a random selection of ten drill bits from a process that follows a Weibull distribution.

To estimate the shape and scale parameters

follow these steps:

Step 1: Rank the data from smallest to largest

Step 2: Calculate the failure probability of each data point using the formula: i/(n+1), where i is the rank of the data point and n is the number of data points.

Step 3: Transform the failure probabilities using the inverse Weibull distribution function: F^(-1)(p) = (−ln(1−p))^β for a given shape parameter β and scale parameter η.

Step 4: Plot the transformed data against the theoretical distribution, which is a straight line for the Weibull distribution.

Step 5: Find the best-fitting line by eye, or by using regression analysis.

The slope of the line is equal to the shape parameter β, and the intercept is equal to the logarithm of the scale parameter ln(η).

The Weibull distribution can be represented as:\(F(x) = 1 − e^(-(x/η)^β)\), where β is the shape parameter and η is the scale parameter. The CDF model corresponding to your parameter estimates can be plotted by using the formula . This can be done by calculating the standard deviation of the residuals, and then calculating the upper and lower limits of the confidence interval using the t-distribution.

The confidence interval can be calculated as:β ± t(n−2,α/2) x SE(β)ln(η) ± t(n−2,α/2) x SE(ln(η))where SE(β) is the standard error estimate for the shape parameter, SE(ln(η)) is the standard error estimate for the logarithm of the scale parameter, and t(n−2,α/2) is the critical value of the t-distribution with n−2 degrees of freedom and a significance level of α/2.The confidence limits can be plotted as two parallel lines around the best-fitting line. The distance between the two lines is equal to the product of the critical value of the t-distribution and the standard error estimate.

for such more questions on probability

https://brainly.com/question/24756209

#SPJ11

To summarize, if the equation Hx = c is inconsistent for some c in ℝⁿ, the equation Hx = 0 is consistent because it always has at least one solution, x = 0.

If the equation Hx=c is inconsistent for some c in Rn, then it means that there is no solution for that particular value of c. However, this does not necessarily mean that the equation Hx=0 is also inconsistent. In fact, it is possible for Hx=0 to have a solution, even if Hx=c does not. This is because the equation Hx=0 represents a homogeneous system of linear equations, while Hx=c represents a non-homogeneous system. Homogeneous systems always have at least one solution (x=0), while non-homogeneous systems may or may not have a solution depending on the specific values of the constants involved.
If the equation Hx = c is inconsistent for some c in ℝⁿ, it means that there is no solution for that specific c value.

Now let's analyze the equation Hx = 0.
An equation is inconsistent if it has no solution. On the other hand, if an equation has at least one solution, it is considered consistent.
Since Hx = c is inconsistent for some c in ℝⁿ, it indicates that the rows of the matrix H do not span the entire space of ℝⁿ. In this case, the equation Hx = 0 will always have at least one solution (x = 0), making it a consistent equation. This is because when you substitute x = 0 into the equation Hx = 0, you get 0 = 0, which is always true.

Therefore, we cannot make any conclusions about the consistency of the equation Hx=0 based on the inconsistency of the equation Hx=c.

Learn more about inconsistent here: brainly.com/question/20512428

#SPJ11

Answer:

1. Mean : 85.6

2. Median : 90

3. Mode : 95

4. Range : 35

Step-by-step explanation:

1. For Mean, you add up all the terms and divide the resulting sum by the number of terms. In this case there were 9 terms that added up to 770, and when you divided that result by 9, the answer was 85.555 repeating. Since the question asks to round to the nearest tenth 85.6 represents the correct mean or average of the class.

2. To find the median, line up all the numbers in order from least to greatest. Like so :

60,75,75,90,90,95,95,95,95

In order to find the median, find the middle term. Since there are 9 terms, the middle term will be the fifth one. In this case, the answer was 90.

3. Mode represents the number that appears the most in a data set. Look at the 9 terms and find the one that appears the most. In this case it is 90.

4. Lastly, to find the range, subtract the lowest value in the data set from the highest one. 95 is the highest and 60 is the lowest. Do \(95-60\) and you will get an answer of 35.

Hope this helps!

Answer:

Mean: 85.6

Median: 90

Mode: 95

Range: 35

Step-by-step explanation:

Mean: Add up all the value and divide by the number of values you have.

75 + 95 + 90 + 95 + 60 + 95 + 75 + 95 + 90 = 770

770 / 9 = 85.6

Median: Rearrange in increasing order + look at middle number

60, 75, 75, 90, 90, 95, 95, 95, 95

Mode: Number that shows up the most.

95 shows up 4 times.

Range: Maximum - Minimum.

95 - 60 = 35.

Answer:

Step-by-step explanation:

Answer: A) between 11 and 12

Step-by-step explanation:

Because 125 squared = 11.18033988 …

Answer:

subtract 3 from both sides

6m+3=51

6m+3-3=51-3

simplify

6m=48

divide both sides

6m/6 = 48/6

simplify

divide the numbers

m=8

solution.

M = 8

Answer:

Yes because it is 90 degrees and the side with 30 is the hypotenuse and the hypotenuse is always the longest side on a right triangle hope this helps :)

Step-by-step explanation:

Answer:

96 cm^2

Step-by-step explanation:

Volume of a cube =(side)^3=64 cm^3.

(side)^3= (4 cm.)^3

or side =4 cm.

Surface area =6×(side)^2..

=6×4×4 cm^2=

96 cm^2. , Answer

Answer:

Step-by-step explanation:

Volume of cube = 64 m³

Side³ = 64

Side = ∛64 = ∛2*2*2*2*2*2

Side = 2*2 = 4 m

Surface area = 6side² = 6* 4² = 6*4*4

                     = 96 m²

The final payment on a $3000 loan with an interest rate of 4.25% made on March 1, repaid with payments of $500 on March 31, $1000 on June 15, and a final payment on August 31, can be calculated.

Step 1: Calculate the interest accrued from March 1 to August 31. The interest can be calculated using the formula: Interest = Principal × Rate × Time. In this case, Principal = $3000, Rate = 4.25% (or 0.0425 as a decimal), and Time = 6 months.

Step 2: Subtract the interest accrued from the total amount repaid. The total amount repaid is the sum of the three payments: $500 + $1000 + Final Payment.

Step 3: Set up an equation using the remaining balance and the interest accrued. The remaining balance is the difference between the total amount repaid and the interest accrued.

Step 4: Solve the equation for the final payment. Rearrange the equation to isolate the final payment variable.

Step 5: Substitute the values of the principal, rate, and time into the interest formula and calculate the interest accrued.

Step 6: Substitute the calculated interest accrued and the total amount repaid into the equation from Step 3 and solve for the final payment variable. The resulting value will be the final payment on the loan.

Learn more about decimal : brainly.com/question/30958821

#SPJ11

For the given triangle and the measures of the given angle the value of x is equal to -11° .

As given in the question,

For the given triangle,

Let us consider the triangle name ABC,

Where,  m ∠A = x + 51° ,

m ∠ABC = 80° ( vertically opposite angle of given 80° angle.)

m ∠ACB = 60° ( vertically opposite angle of given 60° angle.)

To get the value of x we have,

Sum of all the interior angles of a triangle is equal to 180°

m ∠A + m ∠B + m ∠C = 180°

⇒ x + 51° + 80° + 60° = 180°

⇒ x + 191° =  180°

⇒ x = 180° - 191°

⇒ x = - 11°

Therefore, for the given triangle and the measures of the given angle the value of x is equal to -11° .

Learn more about value here

brainly.com/question/14316282

#SPJ1

The output of the accumulator for the input sequence x(n) = nu(n), with the initial condition y(0) = 0, is given by:

y(n) = 1/2n(n+1) for n >= 0

The accumulator is a simple digital signal processing (DSP) system that takes an input sequence and accumulates it over time. The formula for the accumulator described by (2.2.30) is:

y(n) = y(n-1) + x(n)

where y(n) is the output of the accumulator at time n, y(n-1) is the output of the accumulator at the previous time step, and x(n) is the input sequence at time n.

In this case, the input sequence x(n) is given by x(n) = nu(n), where n is an integer and u(n) is the unit step function defined as:

u(n) = {

1, n >= 0

0, n < 0

}

Substituting x(n) into the accumulator formula, we get:

y(n) = y(n-1) + x(n)

y(n) = y(n-1) + n*u(n)

We can calculate the output of the accumulator for different values of n using this formula. However, the output will depend on the initial value of the accumulator, y(0). Without knowing this initial value, we cannot determine the output for all values of n. Therefore, we will assume that the initial value of the accumulator is zero, y(0) = 0.

Using this assumption, we can calculate the output of the accumulator for the first few values of n:

For n = 0:

y(0) = 0

For n = 1:

y(1) = y(0) + 1u(1) = 0 + 11 = 1

For n = 2:

y(2) = y(1) + 2u(2) = 1 + 21 = 3

For n = 3:

y(3) = y(2) + 3u(3) = 3 + 31 = 6

For n = 4:

y(4) = y(3) + 4u(4) = 6 + 41 = 10

And so on.

Therefore, the output of the accumulator for the input sequence x(n) = nu(n), with the initial condition y(0) = 0, is given by:

y(n) = 1/2n(n+1) for n >= 0.

Learn more about accumulator here:

https://brainly.com/question/31813770

#SPJ11

Answer:

The line AB, with A(Ax, Ay), B(Bx, By) and midpoint M(Mx, My) satisfying:

Ax + Bx = 2Mx

Ay + By = 2My

=>

2 + Bx = 2*1

5 + By = 2*2

=> Bx = 0

=> By = -1

=> B(0, -1)

Hope this helps!

:)

Answer:

B(3, 8 )

Step-by-step explanation:

Using the midpoint formula

Given endpoints (x₁, y₁ ) and (x₂, y₂ ) then midpoint is

[ \(\frac{1}{2}\)(x₁ + x₂ ), \(\frac{1}{2}\)(y₁ + y₂ ) ]

let the coordinates of B = (x, y ), then

\(\frac{1}{2}\)(1 + x) = 2 ( multiply both sides by 2 )

1 + x = 4 ( subtract 1 from both sides )

x = 3

and

\(\frac{1}{2}\) (2 + y) = 5 ( multiply both sides by 2 )

2 + y = 10 ( subtract 2 from both sides )

y = 8

Thus

coordinates of B = (3, 8 )

The area of the smallest circle with radius 3 is 28.27 unit². The area of the yellow ring and white ring is 28.27 unit² and 56.55 unit².

The area of the circle is the space occupied by it. It can be given as,

\(A=\pi r^2\)

Here (r) is the radius of the circle.

In this diagram, three concentric circles' rectangle.

The radius of the smallest circle is 3.

\(r_1=3\rm\unit\)

Thus, the area of it is,

\(A=\pi (3)^2\\A=28.27\rm\; unit^2\)

Let suppose the mid-point of the rectangle is D. Here, OB is the diagonal of the rectangle in which,

\(OD=BD\)  

The radius of the yellow ring's outer face from point O is,

\(r_3=OD+BD\\r_3=OD+OD\\r_3=2OD\\\)

OD is the radius of the small circle, which is 3 units long. Thus,

\(r_3=2\times3\\r_3=6\rm\; units\)

The line segment OC is the radius of the white circle from point O. In the triangle COB from the Pythagoras theorem, the radius r₂ of the middle circle is,

\(r_2=\sqrt{r_3^2-r_1^2}\\r_2=\sqrt{6^2-3^2}\\r_2=3\sqrt3\)

Thus, the area of the yellow ring is,

\(A_y=\pi r_3^2-\pi r_2^2\\A_y=\pi (6)^2-\pi (3\sqrt{3})^2\\A_y=28.27\rm\; unit^2\)

The area of white ring is,

\(A_w=\pi r_2^2-\pi r_1^2\\A_w=\pi (3\sqrt{3})^2-\pi (3)^2\\A_w=56.55\rm\; unit^2\)

Hence, the area of the smallest circle with radius 3 is 28.27 unit². The area of the yellow ring and white ring is 28.27 unit² and 56.55 unit².

Learn more about the area of the circle here;

https://brainly.com/question/402655

#SPJ1

Answer:

first step is to add the both angles that are given =78+46

=124

second step is to minus 124 from 180 =180-124

=56 . I used 180 because that is the sum of all the angles in a triangle . so x =56

The statement is false.

The width of a confidence interval depends on several factors, including the sample size, the variability of the data, and the desired level of confidence.

Doubling the population size will increase the total number of possible observations and may increase the sample size, which can lead to a narrower confidence interval.

However, if the variability of the data remains the same or increases, the confidence interval may become wider. Additionally, if the desired level of confidence is increased, the confidence interval will also become wider.

Therefore, the impact of doubling the population size on the width of a confidence interval cannot be determined without considering these other factors.

Learn more about confidence interval

brainly.com/question/24131141

#SPJ11

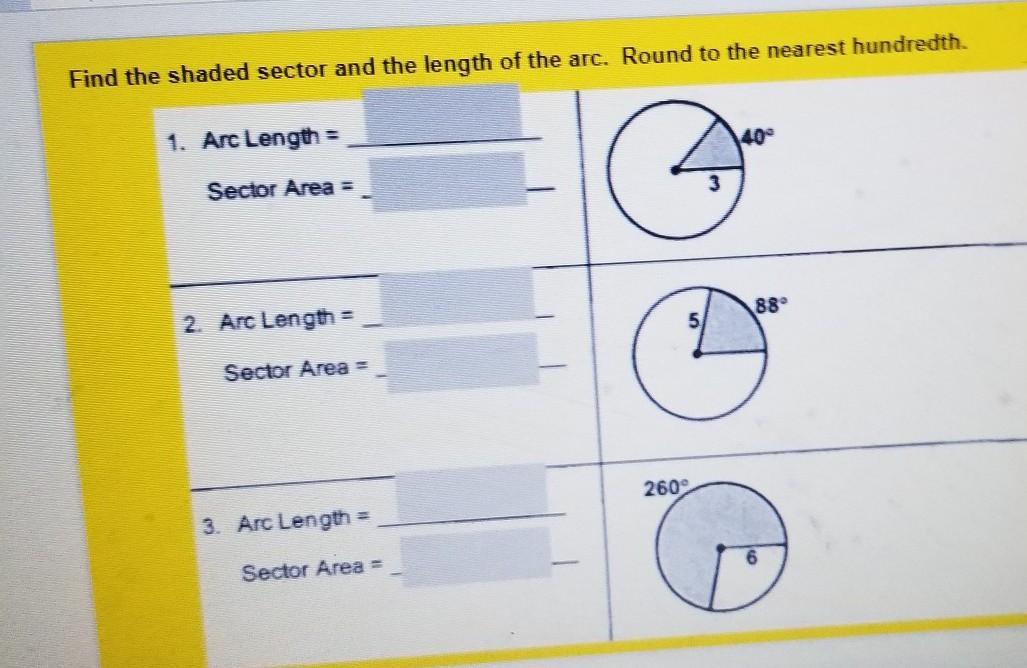
Answer:

Step-by-step explanation:

I'll show you how to do the first one; the other are exactly the same, so pay attention.

The formula for arc length is

\(AL=\frac{\theta}{360}*2\pi r\) where θ is the central angle's measure. It just so happens that the measure of the central angle is the same as the measure of the arc it intercepts. Our arc shows a measure of 40°; this measure is NOT the same as the length. Measures are in degrees while length is in inches, or cm, or meters, etc. Going off that info, our central angle measures 40°. Filling in the formula and using 3.1415 for π:

\(AL=\frac{40}{360}*2(3.1415)(3)\). I'm going to reduce that fraction a bit (and I'll use the same reduction in the Area of a sector coming up next):

\(AL=\frac{1}{9}*2(3.1415)(3)\) which makes

AL = 2.09 units. Now for Area of the Sector. The formula is almost identical, but instead uses the idea that the area of a circle is πr²:

\(A_s=\frac{\theta}{360}*\pi r^2\) where θ is, again, the measure of the central angle (which is the same as the measure of the arc it intercepts). Filling in:

\(A_s=\frac{1}{9}*(3.1415)(3)^2\) which simplifies a bit to

\(A_s=\frac{1}{9}*(3.1415)(9)\). As you can see, the 9's cancel each other out, leaving you with

\(A_s=3.14\) units²

Answer:

Step-by-step explanation:

\(Arc \ Length = 2 \pi r \frac{ \theta}{360}\)

\(Sector \ Area = \pi r^2 \frac{ \theta }{360}\)

\(1) Arc \ Length = 2 \pi \times 3 \times \frac{40}{360} = 2\pi \times 3 \times \frac{1}{9} = \frac{2}{3} \pi = 2.09\)

  \(Sector \ Area = \pi \times 9 \times \frac{40}{360} = 9\pi \times \frac{1}{9} = \pi = 3.14\)

\(2) Arc \ Length = 2 \pi \times 5 \times \frac{88}{360}= 7.68\)

  \(Sector \ Area = \pi \times 25 \times \frac{88}{360} = 19.2\)

\(3) Arc \ Length = 2 \pi \times 6 \times \frac{260}{360} = 27.22\)

  \(Sector \ Area = \pi \times 36 \times \frac{260}{360} = 81.68\)

A) c = 7/2 is the value that makes fX(x) a PDF.

B)  The CDF of X is given by:

C)  P(1/4 ≤ X ≤ 3/4) = 1023/4096.

(a) To show that fX(x) is a PDF, we need to verify two conditions:

Non-negativity: fX(x) is non-negative for all x in the support [0,1].

Total area under the curve equals 1: ∫fX(x)dx over the support [0,1] equals 1.

Let's check these conditions:

For 0 ≤ x ≤ 1, we have:

cx^3 + x/4 > 0

since c > 0, and x^3 + x/4 > 0 for all x in the support [0,1]. Therefore, fX(x) is non-negative for all x in the support.

To find c, we can use the fact that the total area under the curve equals 1:

∫fX(x)dx over the support [0,1] = 1

Integrate fX(x) with respect to x:

∫(cx^3 + x/4)dx over the support [0,1] = 1

[c/4 x^4 + 1/8 x^2] from 0 to 1 = 1

(c/4 + 1/8) - 0 = 1

c/4 = 3/8

c = 3/2 * 8/3 = 4/3 * 7/2 = 7/2

Therefore, c = 7/2 is the value that makes fX(x) a PDF.

(b) The cumulative distribution function (CDF) FX(x) is defined as:

FX(x) = ∫fX(t)dt over the interval [0,x]

For 0 ≤ x ≤ 1, we have:

FX(x) = ∫fX(t)dt over the interval [0,x]

 = ∫(7/2)t^3 + t/4 dt over the interval [0,x]

 = (7/2) * (1/4) * x^4 + (1/4) * (1/2) * x^2

 = (7/8) * x^4 + (1/8) * x^2

For x < 0 or x > 1, FX(x) = 0.

Therefore, the CDF of X is given by:

FX(x) = (7/8) * x^4 + (1/8) * x^2, for 0 ≤ x ≤ 1

FX(x) = 0, otherwise

(c) To find P(1/4 ≤ X ≤ 3/4), we can use the CDF:

P(1/4 ≤ X ≤ 3/4) = FX(3/4) - FX(1/4)

             = [(7/8) * (3/4)^4 + (1/8) * (3/4)^2] - [(7/8) * (1/4)^4 + (1/8) * (1/4)^2]

             = (81/256 + 9/64) - (7/2048 + 1/64)

             = 81/256 + 9/64 - 57/2048

             = 1023/4096

Therefore, P(1/4 ≤ X ≤ 3/4) = 1023/4096.

Learn more about value here:

https://brainly.com/question/17512333

#SPJ11

Answer:

The y-intercept is 3

Gradient: 4

11-3= 8

2-0= 2

8/2= 4

Hope this helped!

Answer:

One of the answers is HL, and the other answer is ΔBCA ≅ ΔDEC, or ΔCAB ≅ ΔCDE.

Step-by-step explanation:

Only right angles use HL as a postulate theorem.

Answer: 1/7

Step-by-step explanation:

From the question, we are informed that it takes Deanna seven hours to paint a fence. The fraction of the fence does she paint in one hour will be one divided by seven hours which can mathematically be written as:

= 1/7

This means that she'll paint 1/7 of the fence in 1 hour.