How do you tell if a graph is exponential or logarithmic?.
Answers
exponential function in the form y=ab^x by identifying the common ratio (b) and y-intercept (a) in the graph and if the log term involved in the given function then it is a logarithmic
How do you identify an exponential function from a graph?
Given a graph of a line, we can write a linear function in the form y=mx+b by identifying the slope (m) and y-intercept (b) in the graph. GIven a graph of an exponential curve, we can write an exponential function in the form y=ab^x by identifying the common ratio (b) and y-intercept (a) in the graph
and if the log term involved in the given function then it is a logarithmic
learn more about of exponential here
https://brainly.com/question/28596571
#SPJ4
Related Questions
Find the area of the trapezoid

Answers
Answer:
112
Step-by-step explanation:
add both bases the multiply by the height
Answer:
112
13 + 15
= 28 ÷ 2
= 14 × 8
= 112
What is the y-value of the solution to the system of equations? 3x 5y = 1 7x 4y = −13
Answers
The solution to the system of equations is x = -3 and y = 2. The y-value of the solution is 2
To find the y-value of the solution to the system of equations, we can solve the system using any suitable method such as substitution or elimination.
Given system of equations:
3x + 5y = 1
7x + 4y = -13
Let's use the method of elimination to solve the system:
Multiply equation 1 by 4 and equation 2 by 5 to make the coefficients of y in both equations equal:
4(3x + 5y) = 4(1) --> 12x + 20y = 4
5(7x + 4y) = 5(-13) --> 35x + 20y = -65
Now, subtract equation 1 from equation 2 to eliminate the y term:
(35x + 20y) - (12x + 20y) = -65 - 4
35x - 12x = -69
23x = -69
x = -69/23
x = -3
Substitute the value of x into equation 1 to find y:
3(-3) + 5y = 1
-9 + 5y = 1
5y = 1 + 9
5y = 10
y = 10/5
y = 2
Therefore, the solution to the system of equations is x = -3 and y = 2. The y-value of the solution is 2.
For more details of equations :
https://brainly.com/question/21620502
#SPJ4
Order the set of numbers from least to greatest: (4 points)
2.71 repeating 71, 2 and 3 over 4, square root 5, 5 over 2
Group of answer choices
2.71 repeating 71, 2 and 3 over 4, square root 5, 5 over 2.
square root 5, 5 over 2, 2.71 repeating 71, 2 3 over 4
5 over 2, square root 5, 2.71 repeating 71, 2 3 over 4
2 3 over 4, 2.71 repeating 71, 5 over 2, square root 5
Answers
The correct order of the set of numbers from least to greatest is 2, 3 over 4, 2.71 repeating 71, 5 over 2, square root 5 option (D) is correct.
To order the given set of numbers from least to greatest, we need to compare their values. We start by noticing that 2 and 3 over 4 are equivalent to 2.75. Next, we compare the values of the remaining numbers. Since the square root of 5 is approximately 2.236 and 5 over 2 is equivalent to 2.5, we have:
2, 3 over 4 < 2.71 repeating 71 < 2.5 < square root 5
Therefore, the set of numbers in order from least to greatest is 2, 3 over 4, 2.71 repeating 71, 5 over 2, square root 5.
Ordering the set of numbers from least to greatest, we get:
2, 3 over 4, 2.71 repeating 71, square root 5, 5 over 2.
To learn more about root follow the link:
https://brainly.com/question/1527773
#SPJ1
The complete question is:
Order the set of numbers from least to greatest: (Group of answer choices)
A. 2.71 repeating 71, 2, and 3 over 4, square root 5, 5 over 2.
B. square root 5, 5 over 2, 2.71 repeating 71, 2 3 over 4
C. 5 over 2, square root 5, 2.71 repeating 71, 2 3 over 4
D. 2, 3 over 4, 2.71 repeating 71, 5 over 2, square root 5
an analyst takes a random sample of 25 firms in the telecommunications industry and constructs a confidence interval for the mean return for the prior year. holding all else constant, if he increased the sample size to 30 firms, how are the standard error of the mean and the width of the confidence interval affected? standard error of the meanwidth of confidence interval aincreasesbecomes wider bincreasesbecomes narrower cdecreasesbecomes wider ddecreasesbecomes narrower multiple choice
Answers
The width of the confidence interval decreases and becomes narrower (D) when the analyst takes a random sample of 30 firms and constructs a confidence interval for the mean return for the prior year, holding all else constant.
When the sample size is increased, the sample mean becomes a better estimator of the population mean, and therefore the standard error of the mean decreases.
Thus, the option (c) which states that the standard error of the mean decreases and becomes wider is incorrect.
Hence, the option (d) is the correct answer.
When the sample size is increased, the standard error of the mean decreases, resulting in a narrower width of the confidence interval.
The formula to calculate the width of the confidence interval is given by;
Width of the Confidence Interval = 2 x Standard Error of the Mean
Thus, if the standard error of the mean decreases, the width of the confidence interval will also decrease.
Hence, the option (d) which states that the width of the confidence interval decreases and becomes narrower is the correct answer.
The standard error of the mean (SEM) is the standard deviation of the sample means' distribution.
It is used to estimate the population mean's standard deviation based on the sample data.
The standard error of the mean (SEM) is inversely proportional to the sample size.
As the sample size grows larger, the SEM gets smaller, indicating a more precise estimate of the population mean.
Learn more about confidence interval here:
https://brainly.com/question/29586058
#SPJ11
Write (6,0) and (0,4) in standard form show all work
Answers
Answer:
There is answer for you sorry but this problem can not be put into standard form i tried but it didn't make any sense maybe you could rewrite the problem but that all i can do for you
Step-by-step explanation:
Describe the steps you would take to solve the given literal equation for m as shown.

Answers
For finding the given literal equation is necessary to apply the power 2 on both sides of the given equation and after that you should rewrite the equation as a function of the variable m .
Power RulesThere are different power rules, see some them:
1. Multiplication with the same base: you should repeat the base and add the exponents.
2. Division with the same base: you should repeat the base and subctract the exponents.
3.Power. For this rule, you should repeat the base and multiply the exponents.
4. Zero Exponent. When you have an exponent equals to zero, the result must be 1.
The question gives: \(t=2\pi *\sqrt{\frac{m}{k}}\) and from the previous equation, you should find \(m=\frac{kt^2}{4\pi ^2}\).
STEP 1 - Applying the power 2 on both sides of the equation \(t=2\pi *\sqrt{\frac{m}{k}}\).
\(t=2\pi *\sqrt{\frac{m}{k}}\\ \\ t^2=(2\pi *\sqrt{\frac{m}{k}})^2\\ \\ t^2=4\pi ^2*\frac{m}{k}\)
STEP 2 - Rewriting the equation \(t^2=4\pi ^2*\frac{m}{k}\) as a function of the variable m .
\(t^2=4\pi ^2*\frac{m}{k}\\ \\ t^2k=4\pi ^2m\\ \\ m=\frac{kt^2}{4\pi ^2}\)
Read more about power rules here:
brainly.com/question/12140519
#SPJ1
Answer:
1. divide both sides of the equation by 2pi
2. square both sides of the equation
3. use the rule to simplify a fraction to a power
4. multiply both sides of the equation by k
Step-by-step explanation:
edg '22
PLEASE HELP
Graph the triangle with the given vertices. Find the length and the slope of each side of the triangle. Then find the coordinates of the midpoint of each side. Is the triangle a right triangle? isosceles? Explain (Assume all variables are positive and m ≠ n)
D (0,n), E(m,n), F(m,0)
Answers
The given triangle is a right triangle
The length and slopeThe coordinates are given as:
D (0,n), E(m,n), F(m,0)
The length is calculated using:
\(l=\sqrt{(x_2-x_1)^2 + (y_2-y_1)^2\)
So, we have:
\(DE=\sqrt{(0-m)^2 + (n-n)^2} = m\)
\(DF=\sqrt{(0-m)^2 + (n-0)^2} = \sqrt{m^2 + n^2\)
\(EF=\sqrt{(m-m)^2 + (n-0)^2} = n\)
The slope is calculated using:
\(m = \frac{y_2 -y_1}{x_2 - x_1}\)
So, we have:
\(DE = \frac{n -n}{0- m} = 0\)
\(DF = \frac{n -0}{0- m} = -\frac nm\)
\(EF = \frac{n -0}{m- m} = \mathbf{unde fined}\)
The coordinates of the midpointsThis is calculated using
\(m = 0.5 * (x_1 + x_2, y_1 + y_2)\)
So, we have:
\(DE = 0.5 * (0 + m, n+ n)=(0.5m, n)\)
\(DF = 0.5 * (0 + m, n+ 0)=(0.5m, 0.5n)\)
\(EF = 0.5 * (m + m, n+ 0)=(m, 0.5n)\)
The type of triangleIn (a), we have:
Lengths
\(DE= m\)
\(DF = \sqrt{m^2 + n^2\)
\(EF= n\)
Slope
\(DE = 0\)
\(DF = -\frac nm\)
\(EF = \mathbf{unde fined}\)
The sides are not equal.
However, the 0 and the undefined slope implies that the triangle is a right triangle because the sides are perpendicular
It should be noted that the triangle cannot be graphed because the coordinates are not numeric
Read more about triangles at:
https://brainly.com/question/26331644
#SPJ1
Please Help ASAP
Jorge has a swimming pool that is rectangular in shape. The length and width of the pool are 24 feet and 14 feet. He also knows that the pool has a consistent depth of 5 feet. He needs to add 4 drops of chlorine for every 50 cubic feet in his pool. How many drops of chlorine should Jorge use to chlorinate his pool properly?
Answers
Answer:
140 drops
Step-by-step explanation:
Length of the pool = 24 feet
Width of the pool = 14 feet
Depth of the pool = 5 feet
Volume of the pool = length × width × depth
= 24 ft * 14 ft * 5 ft
= 1,750 ft³
Volume of the pool = 1,750 ft³
He needs to add 4 drops of chlorine for every 50 cubic feet in his pool.
Number of 50 cubic feet in the volume of the pool = Volume of the pool / 50 ft³
= 1,750 ft³ / 50 ft³
= 35
Number of 50 cubic feet in the volume of the pool = 35
How many drops of chlorine should Jorge use to chlorinate his pool properly?
Number of drops of chlorine Jorge should use = number of drops per 50 ft³ × Number of 50 cubic feet in the volume of the pool
= 4 × 35
= 140 drops
Number of drops of chlorine Jorge should use = 140 drops
Solve For X. Solve similar triangles (advanced)

Answers
7^2 +6^2
49+36 = 85
Radical85 = 9.21
So x will be 3.9
The value of x in the given similar triangles ADB and BCE is 4.5.
To solve for x in the given similar triangles ADB and BCE, we can set up the proportion of corresponding sides:
In triangle ADB, we have:
AB/BD = x/DE
Substitute the given values:
3/4 = x/6
Now, cross-multiply and solve for x:
3 * 6 = 4x
18 = 4x
x = 18/4
x = 4.5
So, the value of x is 4.5.
To know more about similar triangles, refer here:
https://brainly.com/question/29731302
#SPJ6
What is the area of the triangle below (in square units)?
2 mm
14 mm

Answers
Answer:
A = 14 mm²
Step-by-step explanation:
The area (A) of a triangle is calculated as
A = \(\frac{1}{2}\) bh ( b is the base and h the height )
Here b = 14 and h = 2 , then
A = \(\frac{1}{2}\) × 14 × 2 = 7 × 2 = 14 mm²
P L Z H E L P!!!!!!!!!!:)

Answers
The range is how far it stretches from y
The domain is how far it stretches from x
find the steady state solution of the heat conduction equation
Answers
The steady-state solution of the heat conduction equation refers to the temperature distribution that remains constant over time. This occurs when the heat flow into a system is balanced by the heat flow out of the system.
To find the steady-state solution of the heat conduction equation, follow these steps:
1. Set up the heat conduction equation: The heat conduction equation describes how heat flows through a medium and is typically given by the formula:
q = -k * A * dT/dx,
where q represents the heat flow, k is the thermal conductivity of the material, A is the cross-sectional area through which heat flows, and dT/dx is the temperature gradient in the direction of heat flow.
2. Assume steady-state conditions: In the steady-state, the temperature does not change with time, which means dT/dt = 0.
3. Simplify the heat conduction equation: Since dT/dt = 0, the equation becomes:
q = -k * A * dT/dx = 0.
4. Apply boundary conditions: Boundary conditions specify the temperature at certain points or surfaces. These conditions are essential to solve the equation. For example, you might be given the temperature at two ends of a rod or the temperature at the surface of an object.
5. Solve for the steady-state temperature distribution: Depending on the specific problem, you may need to solve the heat conduction equation analytically or numerically. Analytical solutions involve techniques like separation of variables or Fourier series expansion. Numerical methods, such as finite difference or finite element methods, can be used to approximate the solution.
It's important to note that the exact method for solving the heat conduction equation depends on the specific problem and the boundary conditions given. However, the general approach is to set up the heat conduction equation, assume steady-state conditions, simplify the equation, apply the boundary conditions, and solve for the steady-state temperature distribution.
Know more about steady-state solution here:
https://brainly.com/question/15073499
#SPJ11
Please help with graph question!!
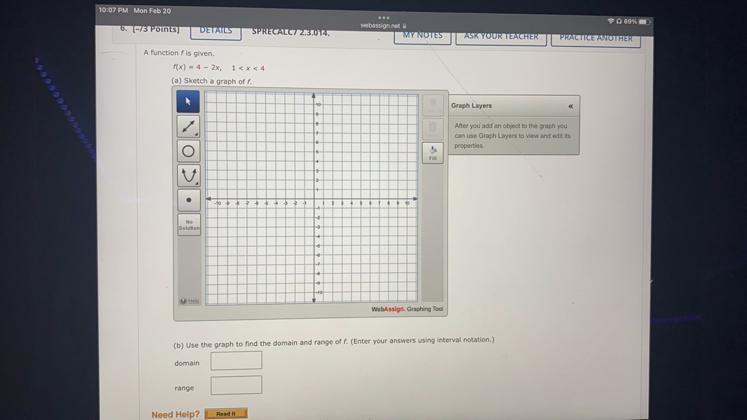
Answers
For the linear function we have:
domain: (1, 4)
range: (-12, 2)
And the graph is on the image at the end.
How to graph the function?Here we want to graph the function:
f(x) = 4 - 2x
We want to graph this on the interval 1 < x < 4
Then the domain is (1, 4)
And the range is given by:
(f(4), f(1))
These values are:
f(4) =4 - 4*4 = 4 - 16 =-12
f(1) = 4 - 2*1 = 2
So the range is (-12, 2)
And the graph of the linear function is on the image below:
Learn more about linear functions at:
https://brainly.com/question/4025726
#SPJ1

Mika has a goal of folding at least 120 paper swans. He can fold 12 paper swans per hour. Which inequaility can Mika use to find h, the number of hours he should fold paper swans to meet or exceed his goal?
Answers
Answer: it would take him 10 hours to make 120 paper
Suppose S is the set of integers that are multiples of 3, and T is the set of integers that are odd. Prove that |S| = |T| by constructing a bijection between S and T.
Answers
The bijection could be defined from the set of numbers and it is proved.
We can define a bijection f from S to T as follows: for any integer x in S, let f(x) = x + 1.
To show that f is a bijection, we need to prove that it is both injective and surjective.
To prove that f is injective, suppose f(x) = f(y) for some x, y in S. Then x + 1 = y + 1, which means x = y. Thus, f is injective.
To prove that f is surjective, let y be an arbitrary element of T. Since y is odd, we can write y = 2n + 1 for some integer n. Now consider the element x = 3n in S. We have f(x) = x + 1 = 3n + 1 = y, so f is surjective.
Therefore, f is a bijection from S to T, so |S| = |T|.
Learn more about Bijection at:
brainly.com/question/13837935
#SPJ4
Consider the following table. What is the probability of blue? Red Blue Green Total Yes 33 44 14 91 No 18 5 28 51 Total 51 49 42 142 91/142 44/49 51/142 49/142
Answers
The probability of Blue is 0.345 (rounded to three decimal places)
Probability is a measure of the likelihood or chance of an event occurring.
In the given table, we have been asked to find the probability of getting the color blue. From the table, we see that the color blue occurs 49 times out of a total of 142 items.
We see that there are 51 red items, 49 blue items, and 42 green items, for a total of 142 items.
Finally, we can calculate the probability of getting the color blue by dividing the number of blue items by the total number of items:
P(Blue) = Number of blue items / Total number of items
= 49 / 142
= 0.345 (rounded to three decimal places)
Learn more about probability here
brainly.com/question/11234923
#SPJ4
Determine which integer will make the inequality 2(n + 2) < 5(n − 1) true.
S:{10}
S:{3}
S:{2}
S:{0}
Answers
Hence, when \(n=10\) the given inequality is true.
What is the inequality?
An inequality is said to be sharp if it cannot be relaxed and still be valid in general.
Here given that,
Which integer will make the inequality \(2(n + 2) < 5(n -1)\) true.
So,
\(2(n + 2) < 5(n-1)\)
When
\(n=10\\\\\\2(10+2) < 5(10-1)\\\\2(12) < 5(9)\\\\24 < 45\)
Hence, when \(n=10\) the given inequality is true.
To know more about the inequality
https://brainly.com/question/23575974
#SPJ1
One of the primary goals of constructing a frequency distribution for quantitative data is to summarize the data in a manner that accurately depicts the data as a whole. True/False?
Answers
One of the primary goals of creating a frequency distribution for quantitative data is to properly represent the data as a whole. As a result, it is correct, as it accurately depicts the data as a whole.
what is a frequency distribution?Frequencies are organized into frequency distributions, which are visual displays, to make the information easier to understand. A frequency distribution can display absolute or relative frequencies, such as ratios or percentages. A frequency distribution can be represented by a graph or a frequency table. A frequency distribution can show both the exact number of observations and the proportion of observations that fall within each range. In the latter case, the distribution is referred to as a relative frequency distribution. The frequency of observation is the number of times it appears in the data. For example, the frequency of the number 9 in the following list of numbers is 5. (Because it happens five times). 1, 2, 3, 4, 6, 9, 9, 8, 5, 1, 1, 9
One of the primary goals of creating a frequency distribution for quantitative data is to properly represent the data as a whole.
As a result, it is correct, as it accurately depicts the data as a whole.
To know more about frequency distribution visit:
brainly.com/question/14926605
#SPJ 4
PLEASE HELP I REAALLY NEED HELP!


Answers
The domain of the exponential function in this problem is given as follows:
0 ≤ n ≤ 5.
How to obtain the domain of the exponential function?The exponential function in this problem is defined by the rule presented as follows:
g(n) = 10(1.02)^n.
In which the variables are listed as follows:
n is the number of days.g(n) is the height of flower after n days.When the study was finished, the height of the flower was of 11.04 inches, hence the day at which the study was finished is obtained as follows:
10(1.02)^n = 11.04
(1.02)^n = 11.04/10
log[(1.02)^n] = log(11.04/10)
Applying the logarithm of power property, we have that:
nlog(1.02) = log(11.04/10)
n = log(11.04/10)/log(1.02)
n = 5.
The domain is the number of days for which the student was realized, hence:
0 ≤ n ≤ 5.
As the number of days cannot assume negative values.
More can be learned about exponential functions at https://brainly.com/question/25537936
#SPJ1
How many elementary events are in the sample space of the experiment of rolling three fair coins? O2 09 O 8 6
Answers
this rectangular prism is intersected by a plane that contains points d, e, k, and l. what is the perimeter of the cross section?
Answers
The perimeter of the cross-section of the rectangular prism is 42 meters.
The cross-section formed by the plane that contains points D, E, K, and L is a trapezoid with bases DE and KL and height 5.
The length of DE can be found using the Pythagorean theorem: DE = √((EK - DK)² + (EL - DL)²) = √((12 - 4)² + (0 - 0)²) = 8 meters.
Similarly, the length of KL is also 8 meters.
Therefore, the perimeter of the cross-section is the sum of the lengths of the sides of the trapezoid, which is given by
perimeter = DE + KL + 2√((EK - DL)² + 5²)
perimeter = 8 + 8 + 2√((12 - 0)² + 5²)
perimeter = 16 + 2*√(169)
perimeter = 16 + 26
perimeter = 42 meters (rounded to the nearest tenth)
Hence, the perimeter of the cross-section is 42 meters.
To know more about rectangular prism:
https://brainly.com/question/21308574
#SPJ4
--The given question is incomplete, the complete question is given
" The rectangular prism is intersected by a plane that contains points D, E, K, and L.
What is the perimeter of the cross section?
Enter your answer in the box. Round only your final answer to the nearest tenth.
m
A rectangular prism with height 5 meters, length 12 meters, and width 4 meters. The vertices are labeled as G, D, H, L, E, F, J, and K."--
Totally out of points
Need triangle geom help !

Answers
Answer:
B. ∠BAC
Step-by-step explanation:
The angle opposite the largest side has the largest angle.

In a clinical trial, 18 out of 863 patients taking a prescription drug daily complained of flulike symptoms. Suppose that it is known that 1.6% of patients taking competing drugs complain of flulike symptoms. Is there sufficient evidence to conclude that more than 1.6% of this drug's users experience flulike symptoms as a side effect at the a = 0.01 level of significance?
Answers
The P-value is > α, do not reject the null hypothesis and conclude that there is not sufficient evidence that more than 1.6% of the people complain about fluelike symptoms.
What is the null hypothesis?
A null hypothesis is a sort of statistical hypothesis that asserts that there is no statistical significance in a particular set of observations. Using sample data, hypothesis testing is performed to determine the believability of a theory.
Here, we have
Given: In a clinical trial, 18 out of 863 patients taking a prescription drug daily complained of flu-like symptoms. Suppose that it is known that 1.6% of patients taking competing drugs complain of flu-like symptoms.
np₀(1-p₀) = 863×0.016×(1-0.016) = 13.6 > 10,
The sample size is greater than 5% of the population size, and the sample mean is 13.8 and the variance is 13.6, the requirements for testing the Hypothesis are satisfied.
H₀: P=0.016
H₁: P>0.016
Test-Statistic:
Z₀ = P₀-P/√P*(1-P)/n)
~ N(0,1)
Under : H₀
Z₀ = (18/863)-0.016/Sqrt(0.016*0.984/864)
Z₀ = 1.14
The P-value is given by :
P( Z>1.14)= 0.127 ( Find using Z table)
Hence, the P-value is > α, do not reject the null hypothesis and conclude that there is not sufficient evidence that more than 1.6% of the people complain about fluelike symptoms.
To learn more about the null hypothesis from the given link
https://brainly.com/question/4436370
#SPJ4
which fraction is halway between 1/2 and and 1 1/4
Answers
Answer:
3/8
Step-by-step explanation:
= (1/4 + 1/2 )/2
= 3/4 ÷ 2
= 3/(4×2)
= 3/8 (final answer)
Answer:
3/8
Step-by-step explanation:
Which point lies on the qraph of 3y=3x
Answers
Answer:
1,1 2,2 x=y so it would be the same numbers for both points
Step-by-step explanation:
Solve the equation.
7x2 + 5 - x = 6x2 + 10
X =
Answers
Answer:
x = -5
Step-by-step explanation:
7 × 2 + 5 - x = 6 × 2 + 10
Multiply.
14 + 5 - x = 12 + 10.
Combine like terms.
19 - x = 24
Isolate x (I'm going to add x and subtract 24 from both sides to avoid changing signs)
-5 = x
Final answer
x = -5
Sketch the region enclosed by y = 3 x and y = 6 x 2 . find the area of the region.
Answers
The area of the region enclosed by the curves: Y = 3X and Y = 6X² is
1/8 sq. unit.
What are Linear Equation and Quadratic Equation?Linear equation are those in which power is 1 or maximum degree is 1. For example: x + y = 3 here the maximum degree is 1.
Quadratics equation are those in which power is 2 or maximum degree is 2. For example: X² + 6X + 3 = 0. here the maximum degree is 2.
Here, we have given two equation:
Y = 3X
Y = 6X²
To find the limit we will equate the both equation:
3X = 6X²
6X² - 3X = 0
3X ( 2X - 1 ) = 0
here we get two values of X, X = 0 and X = 1/2
so, Lower limit = 0 and Upper limit = 1/2.
Area = \(\int\limits^ \frac{1}{2} _0 {(3x - 6x^{2} ) } \, dx\)
Area = ( 3/2 ( x ⁸ ) - 2 ( x³ ) ) --- Limit from: 0 to 1/2
Area = (3/2 ( 1/2 ² ) - 2 ( 1/2 ³) ) - ( 3/2 ( 0² ) - 2 ( 0³) )
Area = 3/8 - 1/4 - 0 + 0
Area = 1/8 sq. unit
Hence,
The area of the region enclosed by the curves: Y = 3X and Y = 6X² is
1/8 sq. unit. For graph See the attached image.
Learn more about " Integration" from here: https://brainly.com/question/14502499
#SPJ4

Question in the picture

Answers
Answer:
180
Step-by-step explanation:
The definition of angles that are supplementary is that their measures add to 180°.
Please help me :( pls help

Answers
Answer:
See below for an explanation
Step-by-step explanation:
I'm unsure of your answer choices, so I'll show you how to solve for the remainder of the whole triangle:
Since we are given a hypotenuse of 5 and a side length of 4 which is adjacent to ∅, the trig function to solve for ∅ would be cosine because cos∅=adjacent/hypotenuse (SOHCAHTOA). This means cos∅=4/5 and taking the arccos (the inverse of cosine aka. cos^-1) of both sides gets us ∅=36.87°
Side AC can be solved by using the Pythagorean Theorem since a²+b²=c² can be turned into 4²+b²=5². We would then have 16+b²=25 which is also b²=9, thus taking the square root of both sides gives us b=3 (since distance is positive). Thus, side AC has a length of 3 units.
∠A can be found by using the Triangle Angle-Sum Theorem. Since all the interior angles of a triangle must add up to 180°, then ∠A+∠B+∠C=180°. We know that since ∠B=36.87° and ∠C=90°, then we have the equation ∠A+36.87°+90°=180°. Combining like terms on the left side gets us ∠A+126.87°=180° and subtracting on both sides get us ∠A=53.13°
Final sides and angles of the triangle:
∠A=53.13°
∠B=36.87°
∠C=90°
BC=4
BA=5
AC=3
an implicit equation for the plane through (4,−3,1) normal to the vector ⟨3,0,1⟩ is
Answers
The implicit equation is 3x+ z -13=0
An implicit equation for the plane through (4,-3,1) normal to the vector <3,0,1> is:
ax + by + cz + d = 0
where a = 3, b = 0, c = 1, d = -13
Implicit function is a function with multiple variables, and one of the variables is a function of the other set of variables. A function f(x, y) = 0 such that it is a function of x, y, expressed as an equation with the variables on one side, and equalized to zero. An example of implicit function is an equation y2 + xy = 0.
The relation y = f(x) is an explicit function, with y represented in terms of x. Further in a implicit function, the relationship between x and y is expressed as g(x, y) = 0, where y is an implicit function of x. More specifically the value of x defines the value of y such that on substitution of x, y in the expression on the left-hand side, equalizes it to zero.
Also, a function f(x, y, z) = 0 such that one variable is dependent on the other two variables, is an implicit function.
To know more about implicit equation, refer here:
https://brainly.com/question/30482202#
#SPJ11