Answers
Answer:
Step-by-step explanation:
11 is the same as 9
10 is the same as number 9
12 i dont know
Related Questions
Miguel can use all or part of his $25 gift card to make a music purchase. Each song costs $1.50, and there is a $1.00 per account activation fee.
Which inequalities can represent this situation if m is the number of songs he can buy? Select two options.
1 + 1.5 m less-than-or-equal-to 25
Answers
Answer:
A, E or 1, 5
Step-by-step explanation:
Its not A, D
Here are two rectangles.
EH = 10 cm
BC= EF
The perimeter of ABCD is 25 cm.
The area of EFGH is 35 cm²
2
Find the length of AB.
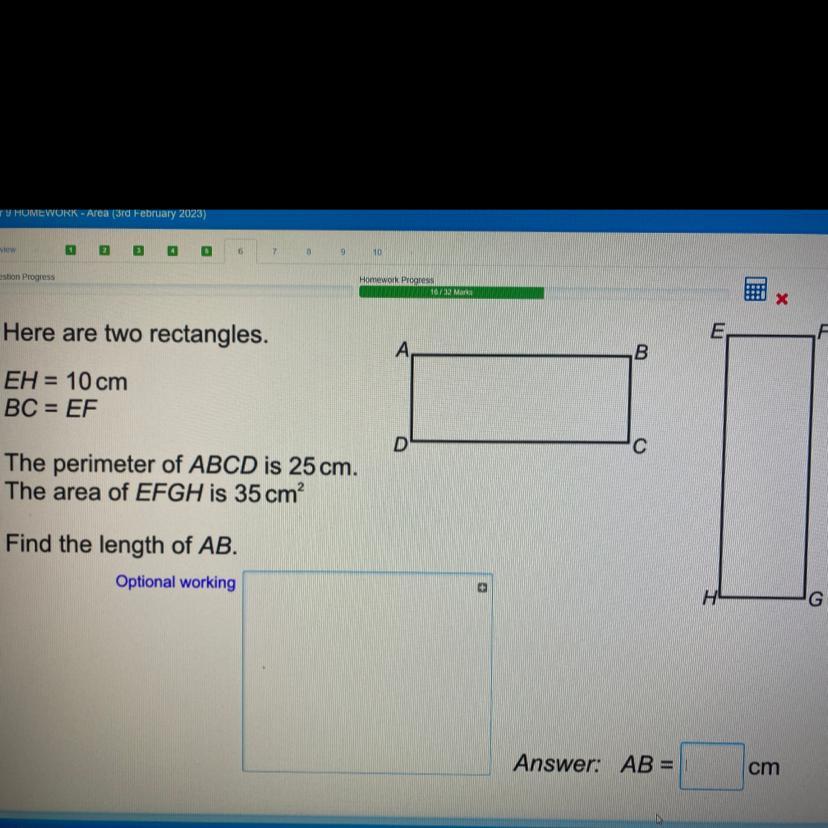
Answers
Answer:
The length of AB is 9 cm.
Step-by-step explanation:
EH = 10 cm, area of EFGH is 35 cm, so
EF = 3.5 cm
BC = AD = 3.5 cm, perimeter of ABCD = 25 cm, so AB = 9 cm since 2(9 + 3.5) = 2(12.5) = 25
the operation manager at a tire manufacturing company believes that the mean mileage of a tire is 30,641 miles, with a variance of 14,561,860 . what is the probability that the sample mean would be less than 31,358 miles in a sample of 242 tires if the manager is correct? round your answer to four decimal places.
Answers
The probability that the sample mean would be less than 31,358 miles in a sample of 242 tires if the manager is correct is 0.9925 (or 99.25%).
What is probability?
Probability is a measure of the likelihood of an event occurring. It is a number between 0 and 1, where 0 means the event is impossible and 1 means the event is certain to happen.
We can use the central limit theorem to approximate the distribution of the sample mean. According to the central limit theorem, if the sample size is sufficiently large, the distribution of the sample mean will be approximately normal with a mean of 30,641 and a standard deviation of sqrt(variance/sample size).
So, we have:
mean = 30,641
variance = 14,561,860
sample size = 242
standard deviation = sqrt(variance/sample size) = sqrt(14,561,860/242) = 635.14
Now, we need to calculate the z-score corresponding to a sample mean of 31,358 miles:
z = (sample mean - population mean) / (standard deviation / sqrt(sample size))
= (31,358 - 30,641) / (635.14 / sqrt(242))
= 2.43
Using a standard normal distribution table or calculator, we can find the probability that a z-score is less than 2.43. The probability is approximately 0.9925.
Therefore, the probability that the sample mean would be less than 31,358 miles in a sample of 242 tires if the manager is correct is 0.9925 (or 99.25%).
To learn more about probability from the given link:
https://brainly.com/question/30034780
#SPJ4
Autumn’s lunch costs $16. 80, and she pays a total of $19. 32 including tip. Autumn left a tip that was what percent of her bill?
Answers
Autumn paid $2.52 as a tip which was 15% of her bill.
A tip is a financial gift or gratuity given to someone as appreciation for their service, often in a restaurant or other service-related setting. To express gratitude for excellent service, it is common practice in many nations to leave a tip that is a portion of the entire cost. In many nations, leaving a tip is customary, especially in the service sector, which includes restaurants, hair salons, hotels, and taxis. For example, it is typical to tip between 15% and 20% of the whole bill in restaurants in the United States for good service.
The total amount Autumn paid includes the cost of the lunch and the tip, so we need to subtract the cost of the lunch to find the amount of the tip.
Tip = Total amount paid - Cost of lunch
Tip = $19.32 - $16.80
Tip = $2.52
To find the percentage of the tip, we need to divide the tip amount by the cost of the lunch and then multiply by 100:
Tip percentage = (Tip / Cost of lunch) x 100
Tip percentage = ($2.52 / $16.80) x 100
Tip percentage = 0.15 x 100
Tip percentage = 15%
Therefore, Autumn left a tip that was 15% of her bill.
To learn more about tip, refer to:
https://brainly.com/question/29279015
#SPJ4
Its line segment please help

Answers
Step-by-step explanation:
\(here \: c \: is \: the \: mid \: point \\ so \: bc = cd \\ 6x - 16 = 4x + 10 \\ x \: terms \: to \: gather \\ 6x - 4x = 10 + 16 \\ 2x = 26 \\ x = \frac{26}{2} = 13 \\ \\ thank \: you\)
What is the volume of this sphere? Use 3.14 and round your answer to the nearest hundredth. 1777561 cubic inches ST
Answers
we have that
the radius is
r=12.1 in
the volume of a sphere is
\(V=\frac{4}{3}\cdot\pi\cdot r^3\)substitute the given values
\(\begin{gathered} V=\frac{4}{3}\cdot3.14\cdot12.1^3 \\ \\ V=7,416.94\text{ in3} \end{gathered}\)Part 2
In this problem we have the diameter of the sphere
D=13.6 mm
so
r=D/2=13.6/2=6.8 in
substitute in the formula
\(\begin{gathered} V=\frac{4}{3}\cdot3.14\cdot6.8^3 \\ V=1,316.42\text{ in3} \end{gathered}\)is this table proportional or nonproportal please help

Answers
Answer:
The table is proportional
50
points :) + brainliest
Answers
Answer:
ok? Whatever. What do you need?
Step-by-step explanation:
Answer:
Step-by-step explanation:
According to the words used by the poet, they made the reader visualize a little village with a group of hardworking men. I know this because of the little details the poet used, like ‘washing their red hands’, or ‘easing their stiff limbs’, meaning that they are tired for an exhausting day of work. To sum it up, the poet gave the effect of a hard working, nostalgic effect.
how many possible number of facemask and face shield can an online seller could sell to get an amount of the least php2 000 based on the data above
Answers
Claudio noticed that the sides of his $1 bill formed angles. Tell whether the angles are right angles, less than right angles, or greater than right angles. Explain.
(no picture to insert)
Answers
Hope this helped you out a bit
In a random sample of 300 elderly men, 65% were married, while in a similar sample of 400 elderly women, 48% were married. Determine a 99% confidence interval estimate for the DIFFERENCE between the percentages of elderly men and women who were married.
Answers
The 99% confidence interval estimate for the difference between the percentages of married elderly men and women is (0.0741, 0.2659).
To determine a 99% confidence interval estimate for the difference between the percentages of elderly men and women who were married, we can use the formula for the confidence interval of two proportions:
CI = (p1 - p2) ± Z * √[(p1 * (1-p1) / n1) + (p2 * (1-p2) / n2)]
Where p1 and p2 are the proportions of married elderly men and women, n1 and n2 are the sample sizes, and Z is the Z-score for a 99% confidence level (which is 2.576).
First, convert the percentages to proportions:
p1 = 0.65 (65% married elderly men)
p2 = 0.48 (48% married elderly women)
n1 = 300 (sample size of elderly men)
n2 = 400 (sample size of elderly women)
Now, plug the values into the formula:
CI = (0.65 - 0.48) ± 2.576 * √[((0.65 * 0.35) / 300) + ((0.48 * 0.52) / 400)]
CI = 0.17 ± 2.576 * √[(0.2275 / 300) + (0.2496 / 400)]
CI = 0.17 ± 2.576 * √(0.00075833 + 0.000624)
CI = 0.17 ± 2.576 * √(0.00138233)
CI = 0.17 ± 2.576 * 0.0372
CI = 0.17 ± 0.0959
Thus, the 99% confidence interval estimate for the difference between the percentages of married elderly men and women is (0.0741, 0.2659).
Visit here to learn more about confidence interval : https://brainly.com/question/29680703
#SPJ11
The vertical axis of the managerial grid measures ________, whereas the horizontal axis measures ________.
Answers
The vertical axis of the managerial grid measures concern for people, whereas the horizontal axis measures concern for production.
A managerial grid model is a self-assessment tool by which individuals and organizations can help identify a manager's or leader's style. The grid was originally developed by Robert R. Blake and Jane S. Mouton in the 1960s and has evolved in subsequent decades.
Based on the care for people and the concern for output, this model first identified five alternative leadership philosophies. Based on Theory Y, the ideal leadership stance in this approach. The grid hypothesis has always developed and changed.
Resilience was included as a new component and two more leadership philosophies were added to the theory. A new text, The Power to Change, was introduced to the grid management seminar in 1999.
To learn more about the managerial grid:
https://brainly.com/question/15737512
#SPJ4
the service time is exponentially distributed with a mean of 10 / hour. what is the probability that the next customer will take more than 5 minutes?
Answers
First, we need to convert the mean from hours to minutes, which is 10 * 60 = 600 minutes.
The exponential distribution is given by the formula:
$P(X > x) = e^{-\lambda x}$
where $\lambda$ is the rate parameter, which is equal to 1/mean for an exponential distribution.
Thus, for this problem, we have:
$\lambda = 1/600$
We want to find the probability that the next customer will take more than 5 minutes, or in other words, $P(X > 5)$.
$P(X > 5) = e^{-\lambda \cdot 5} = e^{-(1/600) \cdot 5} \approx 0.991$
Therefore, the probability that the next customer will take more than 5 minutes is approximately 0.991.
To learn more about probability refer below:
https://brainly.com/question/32004014
#SPJ11
Evaluate the function?

Answers
Answer:
187
Step-by-step explanation:
fill in (-9) where it says x, so 2(-9)^2 -5(-9) -20, which gives 187
Hi can someone please help me with this

Answers
Answer:
Since Q is a center of gravity, we can apply these formulas

Which of the following statements is not true about chi-square distributions? The mean decreases as the degrees of freedom increase. OPG? < 0) = 0 O PU2 > 3) is larger for a chi-square distribution with df = 10 than for df = 1 There are an infinite number of chi-square distributions, depending on degrees of freedom. They are always skewed to the right Previous Only saved at 4:44pm
Answers
The statement "The mean decreases as the degrees of freedom increase" is not true about chi-square distributions.
Is it true that the mean of a chi-square distribution decreases as the degrees of freedom increase?In fact, the mean of a chi-square distribution is equal to its degrees of freedom. It does not decrease as the degrees of freedom increase.
The mean remains constant regardless of the degrees of freedom. This is an important characteristic of chi-square distributions.
Regarding the other statements:
The statement "OPG? < 0) = 0" is true. The probability of a chi-square random variable being less than zero is always zero, as chi-square values are non-negative.The statement "OPU2 > 3) is larger for a chi-square distribution with df = 10 than for df = 1" is true. As the degrees of freedom increase, the right-tail probability of a chi-square distribution also increases.The statement "There are an infinite number of chi-square distributions, depending on degrees of freedom" is true. The number of chi-square distributions is infinite because the degrees of freedom can take any positive integer value.The statement "They are always skewed to the right" is generally true. Chi-square distributions tend to be skewed to the right, especially when the degrees of freedom are small.In summary, the statement that is not true about chi-square distributions is that the mean decreases as the degrees of freedom increase.
Learn more about chi-square distributions:
brainly.com/question/31027207
#SPJ11
The probability that the sum of the two numbers rolled is greater than 3, given that the sum of the numbers is not greater than 5, is . the probability that one of the numbers is a 6 and the sum of the two numbers is an odd number is .
Answers
The probability of this is (1/6) x (3/6) or 1/12. So, the total probability is 3/36 + 1/12, which simplifies to 1/6.
To find the probability that the sum of two numbers rolled is greater than 3, given that the sum is not greater than 5, we need to first find the probability of the sum being less than or equal to 5. This can be done by finding the probability of rolling a 2, 3, 4 or 5 on the two dice. This probability is 4/36 or 1/9. Now, to find the probability that the sum is greater than 3, given that the sum is not greater than 5, we need to use Bayes' theorem. This gives us (probability of sum>3 and sum<=5)/ (probability of sum<=5), which is (2/36)/(4/36) or 1/2.
The probability that one of the numbers is a 6 and the sum of the two numbers is an odd number can be found by considering the cases where the other number is odd or even. If the other number is odd, then the sum will be even and the probability is 3/36. If the other number is even, then the sum will be odd only if the other number is 1, 3 or 5.
To know more about probability visit:-
https://brainly.com/question/31828911
#SPJ11
What do you do to the equation y = x to make its graph move up on the y-axis?
Write two examples of such equations.
Answers
Recall that in Linear Functions, we wrote the equation for a linear function from a graph. Now we can extend what we know about graphing linear functions to analyze graphs a little more closely. Begin by taking a look at Figure 8. We can see right away that the graph crosses the y-axis at the point (0, 4) so this is the y-intercept.
Then we can calculate the slope by finding the rise and run. We can choose any two points, but let’s look at the point (–2, 0). To get from this point to the y-intercept, we must move up 4 units (rise) and to the right 2 units (run). So the slope must be
\displaystyle m=\frac{\text{rise}}{\text{run}}=\frac{4}{2}=2m=
run
rise
=
2
4
=2
Substituting the slope and y-intercept into the slope-intercept form of a line gives
\displaystyle y=2x+4y=2x+4
HOW TO: GIVEN A GRAPH OF LINEAR FUNCTION, FIND THE EQUATION TO DESCRIBE THE FUNCTION.
Identify the y-intercept of an equation.
Choose two points to determine the slope.
Substitute the y-intercept and slope into the slope-intercept form of a line.
EXAMPLE 4: MATCHING LINEAR FUNCTIONS TO THEIR GRAPHS
Match each equation of the linear functions with one of the lines in Figure 9.
\displaystyle f\left(x\right)=2x+3f(x)=2x+3
\displaystyle g\left(x\right)=2x - 3g(x)=2x−3
\displaystyle h\left(x\right)=-2x+3h(x)=−2x+3
\displaystyle j\left(x\right)=\frac{1}{2}x+3j(x)=
2
1
x+3
Graph of three lines, line 1) passes through (0,3) and (-2, -1), line 2) passes through (0,3) and (-6,0), line 3) passes through (0,-3) and (2,1)
Figure 9
SOLUTION
Analyze the information for each function.
This function has a slope of 2 and a y-intercept of 3. It must pass through the point (0, 3) and slant upward from left to right. We can use two points to find the slope, or we can compare it with the other functions listed. Function g has the same slope, but a different y-intercept. Lines I and III have the same slant because they have the same slope. Line III does not pass through (0, 3) so f must be represented by line I.
This function also has a slope of 2, but a y-intercept of –3. It must pass through the point (0, –3) and slant upward from left to right. It must be represented by line III.
This function has a slope of –2 and a y-intercept of 3. This is the only function listed with a negative slope, so it must be represented by line IV because it slants downward from left to right.
This function has a slope of \displaystyle \frac{1}{2}
2
1
and a y-intercept of 3. It must pass through the point (0, 3) and slant upward from left to right. Lines I and II pass through (0, 3), but the slope of j is less than the slope of f so the line for j must be flatter. This function is represented by Line II.
Now we can re-label the lines as in Figure 10.
Figure 10
Finding the x-intercept of a Line
So far, we have been finding the y-intercepts of a function: the point at which the graph of the function crosses the y-axis. A function may also have an x-intercept, which is the x-coordinate of the point where the graph of the function crosses the x-axis. In other words, it is the input value when the output value is zero.
To find the x-intercept, set a function f(x) equal to zero and solve for the value of x. For example, consider the function shown.
\displaystyle f\left(x\right)=3x - 6f(x)=3x−6
Set the function equal to 0 and solve for x.
⎧
⎪
⎪
⎨
⎪
⎪
⎩
0
=
3
x
−
6
6
=
3
x
2
=
x
x
=
2
The graph of the function crosses the x-axis at the point (2, 0).
Q & A
Do all linear functions have x-intercepts?
No. However, linear functions of the form y = c, where c is a nonzero real number are the only examples of linear functions with no x-intercept. For example, y = 5 is a horizontal line 5 units above the x-axis. This function has no x-intercepts.
Graph of y = 5.
Figure 11
A GENERAL NOTE: X-INTERCEPT
The x-intercept of the function is value of x when f(x) = 0. It can be solved by the equation 0 = mx + b.
EXAMPLE 5: FINDING AN X-INTERCEPT
Find the x-intercept of \displaystyle f\left(x\right)=\frac{1}{2}x - 3f(x)=
2
1
x−3.
SOLUTION
Set the function equal to zero to solve for x.
\displaystyle \begin{cases}0=\frac{1}{2}x - 3\\ 3=\frac{1}{2}x\\ 6=x\\ x=6\end{cases}
⎩
⎪
⎪
⎪
⎪
⎪
⎨
⎪
⎪
⎪
⎪
⎪
⎧
0=
2
1
x−3
3=
2
1
x
6=x
x=6
The graph crosses the x-axis at the point (6, 0).
Analysis of the Solution
A graph of the function is shown in Figure 12. We can see that the x-intercept is (6, 0) as we expected.
Figure 12. The graph of the linear function \displaystyle f\left(x\right)=\frac{1}{2}x - 3f(x)=
2
1
The tree diagram shows the sample space of two-digit numbers that can be created using the digits 2, 1, 3, and 6. What is the probability of choosing a number from the sample space that contains 1 or 6 ?
Answers
If we look at the tree diagram, we can see that we have 12 numbers, they are:
{12, 13, 16, 21, 23, 26, 31, 32, 36, 61, 62, 63}
That's our sample space, if we look at each tree we can count how many numbers has 1 or 6, on the first tree we have 2 numbers: 21 and 26. On the second tree we have three numbers: 12, 13 and 16, that's expected because the first digit is 1. For the second tree we have just two, 31 and 36. For the last tree we have three again because of the digit 6.
Therefore the probability will be how many numbers that has 1 or 6 divided by the sample space, then
\(\begin{gathered} P=\frac{2+3+2+3}{12} \\ \\ P=\frac{10}{12} \\ \\ P=\frac{5}{6} \end{gathered}\)The probability is
\(P=\frac{5}{6}\)
Two functions, f and g, are represented by these function machines.

Answers
Answer:
x = -2
Step-by-step explanation:
Since we know they will have the same output, we can set them equal to each other in an equation. We'll use x to represent the input.
3x + 6 = 8(x + 2) (Given)
3x + 6 = 8x + 16 (Distributed the 8)
6 = 5x + 16 (Subtracted 3x on both sides)
-10 = 5x (Subtracted 16 on both sides)
-2 = x (Divided 5 on both sides)
MHS Student Bookmarks Kami Export - How10.4 ReviewA group of 80 trees in a forest aren't growing properly. A botanist determines:• 68 of the trees have a disease or are being damaged by insects• 54 of the trees have a disease• 30 of the trees are being damaged by insectsWhat is the probability that a randomly selected tree has both a disease and is being damaged by insects?0꾧00 %0o1720ΟΟΟRE10123 9 5
Answers
Let the event that a tree has disease be D and the event that tree is being damaged by insects be I.
Therefore,
\(\begin{gathered} |D|=54 \\ |I|=30 \\ |D\cup I|=68 \\ \end{gathered}\)Recall that:
\(\begin{gathered} |D\cup I|=|D|+|I|-|D\cap I| \\ 68=54+30-|D\cap I| \\ \text{ Therefore,} \\ |D\cap I|=84-68=16 \end{gathered}\)The required probability is given by:
\(\frac{|D\cap I|}{80}=\frac{16}{80}=20\%\)Hence, the required probability is 20%=0.2
A certain state uses the following progressive
tax rate for calculating individual income tax:
Income
Progressive
Range ($)
Tax Rate
0
2000
2%
2001 - 9000
5%
9001 and
up
5.4%
Calculate the state income tax owed on a $60,000
per year salary.
tax = $[?]
Round your answer to the nearest whole dollar amount.

Answers
The amount of state income tax owed on a $60,000 per year salary is $3,000.
What should we know about Progressive tax?A progressive tax is one in which the tax rate rises in proportion to the taxable amount. The term progressive refers to the manner in which the tax rate rises from low to high, resulting in a taxpayer's average tax rate being less than the person's marginal tax rate.
We are given that the income is $60,000. The applicable Progressive tax for this income range is 5%.
The state income tax owed on a $60,000 per year salary goes as follows:
= $60,000 * 5%
= $3,000
Read more about Progressive tax
brainly.com/question/1022789
#SPJ1
Write the equation of a circle whose diameter has endpoint (-3,0) and (3,0).
Answers
The equation of the circle is with the diameter given is x² + y² = 9.
What is equation of a circle?The standard form of the equation of a circle is (x - h)² + (y - k)² = r², where (h,k) is the center of the circle.
We can quickly determine the circle's centre and radius thanks to this form. We must first square the x and y terms before rearranging the components in order to transform a circular equation into standard form.
The endpoints of the diameter is (-3,0) and (3,0).
Now, using the section formula the coordinates of radius are:
((-3+3)/2, (0+0)/2) = (0,0
The standard form of the equation of a circle is (x - h)² + (y - k)² = r², where (h,k) is the center of the circle.
Substituting the values:
(x - 0)² + (y - 0)² = 3²
Hence, the equation of the circle is x² + y² = 9.
Learn more about equation of circle here:
https://brainly.com/question/23799314
#SPJ1
The product of two integers is 84. If one
integer is (-12), find the other.
Answers
Answer:
-7
Step-by-step explanation:
-12x = 84
/-12 /-12
x = -7
Answer:
The other integer is - 7.
Step-by-step explanation:
Let the 2 integers be x and y
Given:
one of the integer is -12, Let x = - 12
Product of two integers is 84,
that is,
x × y = 84
- 12 × y = 84 [ given one of the integer is -12 ]
\(\frac{-12 \times y }{-12} = \frac{84 }{-12}\) [ divide both sides by -12 ]
\(y = - 7\)
Joshua bought one pound of strawberries for $3.20. What is the price, in dollars per ounce of strawberries?
\text{1 pound}=
1 pound=
\,\,\text{16 ounces}
16 ounces
Before you try that problem, answer the question below.
How many ounces of strawberries did Joshua buy?
HELP 10
Answers
Answer:
0.20 dollar per oz and Joshua brought 16 oz of strawberry
Step-by-step explanation:
1 lbs = 16 oz
3.20÷ 16
= 20 cent
20 cent per one ounce of strawberry
1 cent = 0.01 dollar
20 cent = 20 × 0.01
20 cents = 0.2 dollar
Twelve is fourteen plus an un-
known number.
Answers
Answer:
The unknown number is -2
Step-by-step explanation:
Let n represent the unknown number then...
12=14+n
Subtract 14 from both sides:
12-14=14-14+n
-2=n
n=-2
Smallest to largest
Help!

Answers
Answer:
I believe it is C... since the largest angle is always across from the longest side, where as the smallest angle is across from the shortest side.
Step-by-step explanation:
hope this helps :P hav a fantastic day
unit 7 right triangles and trigonometry homework 3 similar right triangles and Geometric Mean, pls help.


Answers
The results are listed below:
11) The value of \(x\) is approximately 32.404 units.
12) The value of \(x\) is approximately 10.392 units.
13) The value of \(x\) is approximately 15.875 units.
14) The value of \(x\) is approximately 22.450 units.
15) The value of \(x\) is 11.571 units.
16) The value of \(x\) is 17 units.
17) The value of \(x\) is approximately 21.333 units.
18) The value of \(x\) is 24.857 units.
19) The solution of this system is: \(x \approx 16.155\), \(y \approx 6.462\), \(z\approx 2.4\).
20) The solution of this system is: \(x = 37.5\), \(y = 18\), \(z = 22.5\).
How to calculate missing sides in right triangles
In this question we should apply Pythagorean theorem and algebraic methods to find the missing lengths. Now we proceed to the procedure for each case:
Figure 11We construct the system of equations and find its solution:
\(-x^{2}+y^{2} = 40^{2}\) (1)
\(y^{2}+z^{2} = 62^{2}\) (2)
\(-x^{2}+z^{2} = 12^{2}\) (3)
The solution of this system is: \(x \approx 32.404\), \(y\approx 51.478\), \(z\approx 34.554\). \(\blacksquare\)
Figure 12We construct the system of equations and find its solution:
\(-x^{2} + y^{2} = 3^{2}\) (1)
\(-x^{2}+z^{2} = 36^{2}\) (2)
\(y^{2}+z^{2} = 39^{2}\) (3)
The solution of this system is: \(x \approx 10.392\), \(y \approx 10.817\), \(z \approx 37.470\). \(\blacksquare\)
Figure 13We construct the system of equations and find its solution:
\(x^{2}-y^{2} = 14^{2}\) (1)
\(-y^{2}+z^{2} = 4^{2}\) (2)
\(x^{2}+z^{2} = 18^{2}\) (3)
The solution of this system is: \(x \approx 15.875\), \(y\approx 7.483\), \(z \approx 8.485\). \(\blacksquare\)
Figure 14We construct the system of equations and find its solution:
\(x^{2}-y^{2} = 8^{2}\) (1)
\(-y^{2}+z^{2} = 8^{2}\) (2)
\(x^{2}+z^{2} = 63^{2}\) (3)
The solution of this system is: \(x \approx 22.450\), \(y \approx 20.976\), \(z \approx 58.865\). \(\blacksquare\)
Figure 15We construct the system of equations and find its solution:
\(-x^{2}+y^{2} = 18^{2}\) (1)
\(z^{2} = 28^{2}+18^{2}\) (2)
\(z^{2}+y^{2} = (x+28)^{2}\) (3)
The solution of this system is: \(x = 11.571\), \(y \approx 21.398\), \(z\approx 33.287\). \(\blacksquare\)
Figure 16We construct the system of equations and find its solution:
\(y^{2} = 20^{2}-8^{2}\) (1)
\(x^{2}+y^{2}-z^{2} = 0\) (2)
\((x+8)^{2}-z^{2}= 20^{2}\) (3)
The solution of this system is: \(x = 17\), \(y \approx 18.330\), \(z = 25\). \(\blacksquare\)
Figure 17
We construct the system of equations and find its solution:
\(y^{2} = 16^{2}-12^{2}\) (1)
\((x-12)^{2}+y^{2}-z^{2} = 0\) (2)
\(x^{2}-z^{2} = 16^{2}\) (3)
The solution of this system is: \(x \approx 21.333\), \(y\approx 10.583\), \(z \approx 14.110\). \(\blacksquare\)
Figure 18We construct the system of equations and find its solution:
\(-(x-21)^{2}+y^{2} = 9^{2}\) (1)
\(z^{2} = 21^{2}+9^{2}\) (2)
\(-x^{2}+y^{2}+z^{2} = 0\) (3)
The solution of this system is: \(x = 24.857, y \approx 9.791, z \approx 22.847\). \(\blacksquare\)
Figure 19We construct the system of equations and find its solution:
\(y^{2}-z^{2} = 6^{2}\) (1)
\(x^{2} = 6^{2}+15^{2}\) (2)
\(x^{2}+y^{2}-(15+z)^{2} = 0\) (3)
The solution of this system is: \(x \approx 16.155\), \(y \approx 6.462\), \(z\approx 2.4\). \(\blacksquare\)
Figure 20We construct the system of equations and find its solution:
\(y^{2} = 30^{2}-24^{2}\) (1)
\((x-24)^{2}+y^{2}-z^{2} = 0\) (2)
\(x^{2}-z^{2} = 30^{2}\) (3)
The solution of this system is: \(x = 37.5\), \(y = 18\), \(z = 22.5\). \(\blacksquare\)
To learn more on right triangles, we kindly invite to check this verified question: https://brainly.com/question/7894175
What is the square number of 5?
a) 125
b) 16
c) 10
d) 5
Answers
Answer:
25 is your answer .
hope it is helpful to you
Answer:
25 is the correct answer
Hope it helps you have a good day ☺
Can you help me with this please. It was question on my test but it didn't make any sense since i got a repeating decimal.
3.5g-2.7g=-6
Answers
The solution of the linear equation 3.5g - 2.7g = -6 will be negative 7.5.
What is the solution to the equation?The allocation of weights to the important variables that produce the calculation's optimum is referred to as a direct consequence.
If the maximum power of the equation is one. Then the equation will be a linear equation.
The linear equation is given below.
3.5g - 2.7g = -6
Simplify the equation, then we have
3.5g - 2.7g = -6
0.8g = -6
g = - 6 / 0.8
g = - 7.5
The solution of the linear equation 3.5g - 2.7g = -6 will be negative 7.5.
More about the solution of the equation link is given below.
https://brainly.com/question/545403
#SPJ1
