order of fractions with like-numerator
Answers
When comparing fractions with the same numerator, the order is determined by the size of their denominators, with the fraction having the smaller denominator placed first or to the left.
When comparing fractions with the same numerator, the order is determined by the size of their denominators. The fraction with the smaller denominator is considered to be larger.
Here's a step-by-step process to order fractions with the same numerator:
Identify the fractions with the same numerator.
Compare the denominators of the fractions.
The fraction with the smaller denominator is greater than the fraction with the larger denominator. In other words, the fraction with the smaller denominator is placed to the left or comes first when ordering from least to greatest.
For example, let's order the fractions 3/5, 3/7, and 3/4:
Comparing the denominators:
The denominator of 3/5 is 5.
The denominator of 3/7 is 7.
The denominator of 3/4 is 4.
Since 4 < 5 < 7, the order from least to greatest is:
3/4, 3/5, 3/7.
Therefore, when comparing fractions with the same numerator, the order is determined by the size of their denominators, with the fraction having the smaller denominator placed first or to the left.
for such more question on fractions
https://brainly.com/question/78672
#SPJ8
Related Questions
which number has an absolute value that is greater then 50?
Answers
PLEASE THESE 2 HELPPPPPPPPPPPP

Answers
Answer:
c and g
Step-by-step explanation:
\(494 divided by 19,594\)
Answers
x - 47 = 47 help pls
Answers
do the inverse operation to both sides to isolate x (to make it alone on one side)
X - 47 = 47
+47 on both sides
x = 94
Explanation)
You have to get the x by itself so you’d have to move the -47 to the other side. Once you do that the 47 will turn positive so you’d have
47+47 = 94
X=94
Adam wraps the top edge of the gift box shown with gold ribbon. The top and bottom edges of the box are square. If Adam has cm of gold ribbon, does he have enough to decorate the top of the box?
Answers
This question is incomplete and lacks the required diagram
Complete Question
Adam wraps the top edge of the gift box shown with gold ribbon. The top and bottom edges of the box are square. If Adam has 24 1/4 inches of gold ribbon, does he have enough to decorate the top of the box?
Answer:
Yes, he has enough ribbon to decorate the top of the box.
Step-by-step explanation:
From the above question, we are told that the volume of the box = 296cm³
Volume of the box = a³
296cm³ = a³
a = √296cm³
a = 6.6644437033cm
The side length of the box = 6.6644437033cm
The length of the ribbon = 24 1/4 inches
= 24.25 inches long.
Converting this to centimeters =
1 inch = 2.54cm
24.25 inches =
24.25 × 2.54 cm
= 61.595cm.
Length of the ribbon = 61.595cm.
Since from the above, we know the edges of the cube = square
Edge length of the box = 6.6644437033cm
The perimeter of a top of the box
= 4a
= 6.6644437033cm × 4
= 26.657774813 cm
Subtracting the perimeter of the top of the box from the length of the ribbon
61.595cm - 26.657774813 cm
= 34.937225187 cm ( ribbon left)
Therefore, he has enough ribbons left

Leila wants to rent a boat and spend at most $93. The boat costs $8 per hour, and Leila has a discount coupon for $3 off. What are the possible numbers of
hours Leila could rent the boat?
Use t for the number of hours.
Write your answer as an inequality solved for t.
Answers
Answer:
0 ≤ t ≤ 18
Step-by-step explanation:
The cost of renting the boat without any discount is $8 per hour. However, Leila has a discount coupon for $3 off, so the effective cost per hour would be $8 - $3 = $5.
Let's assume Leila rents the boat for t hours. The total cost of renting the boat for t hours would be $5 multiplied by t, which is 5t.
According to the problem, Leila wants to spend at most $93. Therefore, we can set up the following inequality:
5t ≤ 93
This inequality represents the condition that the total cost of renting the boat (5t) should be less than or equal to $93.
Simplifying the inequality:
5t ≤ 93
Dividing both sides by 5 (since the coefficient of t is 5):
t ≤ 93/5
t ≤ 18.6
Since we cannot rent the boat for a fraction of an hour, we can round down the decimal value to the nearest whole number:
t ≤ 18
0 ≤ t ≤ 18
Answer: 0≤t≤12
Step-by-step explanation:
(I’m not sure if it’s 5 dollars off per hour, or total, but here’s what I did!)
If Leila has a $3 coupon, than she can spend +$3 because when you get a coupon, you can spend more, so 93+3 is equal to 96, now we just divide by 8 (because a boat costs $8 per hour) and we get 96/8=12.
Then, in inequality form it’s t≤12, because she can rent the boat for at most 12 hours, you could also do 0≤t≤12, because you can’t rent it for a negative amount of time, but either works.
exercise 6.1.7: find the laplace transform of a cos(ωt) b sin(ωt).
Answers
The Laplace transform of a cos(ωt) b sin(ωt) is [(a + ib)s]/[(s^2) + ω^2].
We can use the identity cos(a)sin(b) = (1/2)(sin(a+b) - sin(a-b)) to write:
a cos(ωt) b sin(ωt) = (a/2)(e^(iωt) + e^(-iωt)) + (b/2i)(e^(iωt) - e^(-iωt))
Taking the Laplace transform of both sides, we get:
L{a cos(ωt) b sin(ωt)} = (a/2)L{e^(iωt)} + (a/2)L{e^(-iωt)} + (b/2i)L{e^(iωt)} - (b/2i)L{e^(-iωt)}
Using the fact that L{e^(at)} = 1/(s-a), we can evaluate each term:
L{a cos(ωt) b sin(ωt)} = (a/2)((1)/(s-iω)) + (a/2)((1)/(s+iω)) + (b/2i)((1)/(s-iω)) - (b/2i)((1)/(s+iω))
Combining like terms, we get:
L{a cos(ωt) b sin(ωt)} = [(a + ib)/(2i)][(1)/(s-iω)] + [(a - ib)/(2i)][(1)/(s+iω)]
Simplifying the expression, we obtain:
L{a cos(ωt) b sin(ωt)} = [(a + ib)s]/[(s^2) + ω^2]
Therefore, the Laplace transform of a cos(ωt) b sin(ωt) is [(a + ib)s]/[(s^2) + ω^2].
Learn more about Laplace transform here
https://brainly.com/question/29583725
#SPJ11
The Laplace transform of acos(ωt) + bsin(ωt) is (as + bω) / (s^2 + ω^2).
To find the Laplace transform of a function, we can use the standard formulas and properties of Laplace transforms.
Let's start with the Laplace transform of a cosine function:
L{cos(ωt)} = s / (s^2 + ω^2)
Next, we'll find the Laplace transform of a sine function:
L{sin(ωt)} = ω / (s^2 + ω^2)
Using these formulas, we can find the Laplace transform of the given function acos(ωt) + bsin(ωt) as follows:
L{acos(ωt) + bsin(ωt)} = a * L{cos(ωt)} + b * L{sin(ωt)}
= a * (s / (s^2 + ω^2)) + b * (ω / (s^2 + ω^2))
= (as + bω) / (s^2 + ω^2)
Know more about Laplace transform here:
https://brainly.com/question/31481915
#SPJ11
A supermarket gives a special
offer to cus-
tomers who purchase at least a pack of
vests and a pack of T-shirts. The offer is
restricted to a total of 7 of these items.
a) Write down three inequalities which
must be satisfied.
(b) Draw the graphs of the above condi-
tions and shade the region that satis-
fies them.
(c) If the supermarket makes a gain of N5
on each vest and N8 on each T-shirt,
find the maximum gain made by the
supermarket.
Answers
A) the three inequalities that must be satisfied are:
The number of vests, represented by x, must be a non-negative integer: x ≥ 0.The number of T-shirts, represented by y, must also be a non-negative integer: y ≥ 0.The total number of vests and T-shirts must not exceed 7: x + y ≤ 7.B) Graph shaded and satisfying all conditions is attached.
What is an inequality?An inequality in mathematics is a relationship that makes a non-equal comparison between two integers or other mathematical expressions.
It is most commonly used to compare the sizes of two numbers on a number line.
Learn more about Inequality:
https://brainly.com/question/29773201
#SPJ1

use cylindrical coordinates to evaluate the triple integral ∫∫∫ex2 y2−−−−−−√dv, where e is the solid bounded by the circular paraboloid z=1−9(x2 y2) and the xy -plane.
Answers
The triple integral ∫∫∫ex^2 y^2 dv in cylindrical coordinates evaluates to ∫ from 0 to 1, ∫ from 0 to 2π, and ∫ from 0 to (1-9r^2) e^r^2cos^2θsinr drdθdz.
In cylindrical coordinates, the given solid e is represented by the inequality 0 ≤ z ≤ 1-9r^2. Therefore, the limits of integration for z are 0 to 1-9r^2. The circular base of the solid is given by x^2 + y^2 ≤ 1/(9z), which can be rewritten as r^2 ≤ 1/(9z).
Thus, the limits of integration for r are 0 to √(1/(9z)). The angle θ ranges from 0 to 2π. Hence, the triple integral can be expressed as ∫ from 0 to 1, ∫ from 0 to 2π, and ∫ from 0 to √(1-9r^2) e^r^2cos^2θsinr drdθdz. Solving this integral yields the required answer.
For more questions like Integral click the link below:
https://brainly.com/question/18125359
#SPJ11
PLEASE HELP ASAP
Question 1(Multiple Choice Worth 2 points)
(Identifying Transformations LC)
Use the image to determine the direction and angle of rotation.
Graph of polygon ABCD in quadrant 1 with point A at 1 comma 5. A second polygon A prime B prime C prime D prime in quadrant 3 with point A prime at negative 1 comma negative 5.
90° counterclockwise rotation
180° counterclockwise rotation
90° clockwise rotation
270° clockwise rotation
Answers
The direction and angle of rotation needed to transform polygon ABCD to polygon A' B' C' D' is a 90° counterclockwise rotation.
What is a polygon?A polygon is a compact geometric object composed of three or more straight line segments known as sides. The sides of a polygon only meet at their ends, which are known as vertices. Polygons can have any number of sides, although most are studied with three to twelve sides.
In this question,
We may deduce from the image and the polygon position that a 90° anticlockwise rotation would transform polygon ABCD into polygon A' B' C' D'.
Consider rotating the whole quadrant 1 graph 90° anticlockwise around the origin, which is the graph's centre. Point A at (1,5) would then be moved to point A' at (-5,-1) in quadrant 3, while the remaining points of polygon ABCD would be moved to points B', C', and D' in quadrants 4, 3, and 2, producing polygon A' B' C' D'.
As a result, the rotation required to convert polygon ABCD to polygon A' B' C' D' is a 90° anticlockwise rotation.
To know more about polygon, visit
brainly.com/question/24464711
#SPJ1

what is definition of the matrix
Answers
Answer:
Matrix is an arrangement of numbers into rows and columns. Make your first introduction with matrices and learn about their dimensions and elements. A matrix is a rectangular arrangement of numbers into rows and columns. For example, matrix A has two rows and three columns.
Step-by-step explanation:
Rows and columns of numbers are arranged in a matrix.
What is Matrix ?The term "matrix" refers to an assortment of numbers put up in rows and columns to form a rectangular array. The elements, or entries, of the matrix are the integers. A rectangular table of numbers is known as a matrix, or more generally, a table made up of abstract quantities that may be multiplied and added.
Defined and indicated :In a matrix, the horizontal lines are referred to as rows, and the vertical lines as columns. An m-by-n matrix is one that has m rows and n columns; m and n are referred to as the matrix's dimensions. Always list the number of rows before listing the number of columns when describing a matrix's dimensions.
To know more about matrix :
https://brainly.com/question/29861416
#SPJ4
What are binary integer variables?
a. Variables with any two values, a and b.
b. Variables with values 0 and 1.
c. Variables whose sum of digits is 2.
d. Variables with values between 0 and 1.
Answers
Binary integer variables are variables whose values consist of two values, 0 and 1.
Correct answer will be :- b. Variables with values 0 and 1.
These values are also known as bits, which are represented as 0 and 1 in computers. Binary integer variables are used in computing as a way to represent numbers, characters, and instructions. Binary integer variables are used to represent information in digital systems, because they can be used to represent any value with a single bit.
For example, a single bit can represent a number, letter, or instruction. Binary integer variables are also used in computer programming, as they can be used to represent boolean values, such as true and false. Additionally, they can be used to represent various types of data, such as numbers, characters, and images.
know more about integer variables here
https://brainly.com/question/27855584#
#SPJ11
If the allele frequency of the dominant allele is 0.4, what value is used for the term p^2 in the equation p^2+ 2pq + q^2 = 1?
Answers
The value is used for the term p^2 in the equation p^2+ 2pq + q^2 = 1 is 0.16.
According to the statement
we have given that the allele frequency of the dominant allele is 0.4, and we have to find that the value of p^2 in the equation p^2+ 2pq + q^2 = 1.
So, For this purpose, we know that the
The allele frequency represents the incidence of a gene variant in a population. Alleles are variant forms of a gene that are located at the same position, or genetic locus, on a chromosome.
And here
allele frequency is the 0.4 and represent the value of P.
So, The value of p is 0.4 and the
Then p^2 = (0.4)^2
so, the value becomes
p^2 = (0.4)^2
p^2 = 0.16.
So, The value is used for the term p^2 in the equation p^2+ 2pq + q^2 = 1 is 0.16.
Learn more about allele frequency here
https://brainly.com/question/6139888
#SPJ4
$20,000 is deposited into an account at an APR of 8%. What will be the new account balance after 9 months?
Answers
STEP - BY - STEP EXPLANATION
What to find?
The new the new account balance after 9 months.
Given:
Principal (p) = $20 000
Rate(R) = 8
Time(t) = 9 months = 9/12 year = 0.75 years.
We will solve the given problem using the steps below:
Step 1
State the formula that will be use to solve the problem.
\(I=\frac{P\times R\times T}{100}\)Where I is the interest.
Step 2
Substitute the values into the formula.
\(I=\frac{20000\times8\times0.75}{100}\)\(=\frac{120000}{100}\)\(=1200\)Hence, interest(I) = $1200
But;
Amount = principal + Interest
= 20 000 + 1200
=21200
Therefore, the account balance after 9 months is $21200
ANSWER
$21200
If you invest $900 in a bank where it will earn 8 percent compounded annually, how much will it be worth at the end of seven years? Complete the steps below using cell references to given data or previous calculations. In some cases, a simple cell reference is all you need. To copy/paste a formula across a row or down a column, an absolute cell reference or a mixed cell reference may be preferred. If a specific Excel function is to be used, the directions will specify the use of that function. Do not type in numerical data into a cell or function. Instead, make a reference to the cell in which the data is found. Make your computations only in the green cells highlighted below. In all cases, unless otherwise directed, use the earliest appearance of the data in your formulas, usually the Given Data section. Given Data: Annual Interest Rate 8% Number of years 7 Money available for investing S900.00 Value of investment after 7 years
Answers
The investment will be worth approximately $1,546.45 at the end of 7 years. To calculate the value of the investment after 7 years, we can use the formula for compound interest:
Value = Principal * (1 + interest rate)^time
Given Data:
Principal (P) = $900
Annual Interest Rate (r) = 8% or 0.08
Number of years (t) = 7
Substituting the values into the formula, we have:
Value = $900 * (1 + 0.08)^7
Calculating the exponent:
(1 + 0.08)^7 = 1.08^7 ≈ 1.718279
Now we can calculate the value of the investment:
Value = $900 * 1.718279 ≈ $1,546.45
Therefore, the investment will be worth approximately $1,546.45 at the end of 7 years.
In this calculation, we used the compound interest formula, which takes into account the initial principal, the annual interest rate, and the number of compounding periods (in this case, 7 years). The interest is compounded annually, meaning that at the end of each year, the interest earned is added to the principal for the next year's calculation.
Learn more about interest here:
brainly.com/question/7571656
#SPJ11
.Question 7. (12 points) Show that if f(z) = u(x, y) +iv(x, y) is an entire function and the real part is bounded. i.e. there exists M >0 such that |u(x, y)|
Answers
To show that if f(z) = u(x, y) + iv(x, y) is an entire function and the real part is bounded, i.e., there exists M > 0 such that |u(x, y)| < M for all (x, y), we can use the Cauchy-Riemann equations and Liouville's theorem.
Since f(z) is entire, it satisfies the Cauchy-Riemann equations:
∂u/∂x = ∂v/∂y and ∂u/∂y = -∂v/∂x.
Taking the derivative of the first equation with respect to y and the derivative of the second equation with respect to x, we have:
∂²u/∂x∂y = ∂²v/∂y² and ∂²u/∂y² = -∂²v/∂x².
Combining these two equations, we get:
∂²u/∂x∂y + ∂²u/∂y² = 0.
Since the mixed partial derivatives of u with respect to x and y are equal and continuous, u satisfies the Laplace's equation in the entire complex plane.
Now, using Liouville's theorem, since u is a harmonic function (satisfying Laplace's equation) and it is bounded, it must be constant. Therefore, u(x, y) is a constant function.
Since u(x, y) is constant and bounded, we can choose M = |u(x, y)| as the bound for the real part of f(z). Hence, |u(x, y)| < M for all (x, y), as required.
Therefore, we have shown that if f(z) = u(x, y) + iv(x, y) is an entire function and the real part is bounded, there exists M > 0 such that |u(x, y)| < M for all (x, y).
Learn more about Cauchy-Riemann here:
https://brainly.com/question/30385079
#SPJ11
Construct a truth table for each of these compound propositions
a) p → ⇁p
b) p ↔ ⇁p
c) p ⊕ (p V q) d) (p ∧ q) → (p V q) e) (p → ⇁p) ↔ (p ↔ q) f) (p ↔ q) ⊕ (p ↔ ⇁q)
Answers
After considering the given data we conclude that there truth table is possible and is placed in the given figures concerning every sub question.
A truth table is a overview that projects the truth-value of one or more compound propositions for each possible combination of truth-values of the propositions starting up the compound ones.
Every row of the table represents a possible combination of truth-values for the component propositions of the compound, and the count of rows is described by the range of possible combinations.
For instance, if the compound has just two component propositions, it comprises four possibilities and then four rows to the table. The truth-value of the compound is projected on each row comprising the truth functional operator.
To learn more about truth table
https://brainly.com/question/28605215
#SPJ4



PLEASE HELP DUE IN 30 MINUTES :((
Which best describes the effect on the graph of f(x) = 4x+8 if the y-intercept is changed to -3?

Answers
a signal can be formed by running different colored flags up a pole. how many distinct signals can be made with 6 flasgs if 3 of them are red, 2 of them are blue, and 1 is green?
Answers
Answer:
6
Step-by-step explanation:
The number of signals will be the factorial of 3 which is 6
That is
3×2×1=6
This question is similar to asking the combination of clothes you'll put on when you have 3 shirts and 6 pants and 1 hat, the answer is 18.
On a coordinate plane, 2 triangles are shown. Triangle A B C has points (negative 3, negative 1), (negative 1, 2), and (negative 5, 3). Triangle R S T has points (1, 1), (3, 4), and (5, 0).
Triangle RST is rotated 180° about the origin, and then translated up 3 units. Which congruency statement describes the figures?
ΔRST ≅ ΔACB
ΔRST ≅ ΔABC
ΔRST ≅ ΔBCA
ΔRST ≅ ΔBAC
Answers
The congruency statement that describes the figure is: D. ΔRST ≅ ΔBAC.
What is a Congruency Statement?A congruency statement is a statement that states that two triangles are congruent to each other.
180 degrees rotation around the origin will give us the rule: (x, y) → (-x, -y)
Therefore, applying this rule to triangle RST that was rotated, we would have:
R'(-1, -1)
S'(-3, -4)
T'(-5, 0)
Translating R'S'T' 3 units up, using the rule, (x, y + 3) will give us:
B(-1, 2)
A(-3, -1)
C(-5, 3)
Therefore, we can conclude that, D. ΔRST ≅ ΔBAC.
Learn more about congruency statement on:
https://brainly.com/question/1675117
#SPJ1

Answer:its D just took test:)
Step-by-step explanation:
The American Medical Association reported: "During the first hour after using cocaine, the user's risk of heart attack increases nearly 24 times. The average (mean) age of people in the study who suffered heart attacks soon after using cocaine was only 44. That's about 17 years younger than the average heart attack patient. Of the 38 cocaine users who had heart attacks, 29 had no prior symptoms of heart disease." Assume that the standard deviation of the age of people who suffered heart attacks soon after using cocaine was 10 years. In a random sample of size 49, what is the probability the mean age at heart attack after using cocaine is greater than 42?
A. 0.4207
B. 0.5793
C. 0.0808
D. 0.9192
Answers
The probability the mean age at heart attack after using cocaine is greater than 42 is 0.9192. Hence, the correct option is D. 0.9192.
The standard deviation of the age of people who suffered heart attacks soon after using cocaine was 10 years. In a random sample of size 49, what is the probability the mean age at heart attack after using cocaine is greater than 42?We are given the following details:
The mean age of people in the study who suffered heart attacks soon after using cocaine was only 44.
Standard deviation = 10
Sample size = 49
Now we need to find the z-score using the formula:
z = (x - μ) / (σ / √n)
wherez is the z-score
x is the value to be standardized
μ is the mean
σ is the standard deviation
n is the sample size.
Substitute the values in the formula as given,
z = (42 - 44) / (10 / √49)z = -2 / (10/7)
z = -1.4
Probability of z > -1.4 can be found using the standard normal distribution table or calculator.
P(z > -1.4) = 0.9192
Therefore, the probability the mean age at heart attack after using cocaine is greater than 42 is 0.9192. Hence, the correct option is D. 0.9192.
Learn more about probability here:
https://brainly.com/question/31828911
#SPJ11
8) When 2. 49 is multiplied by 0. 17, the result (rounded to 2 decimal places) is:
A) 0. 04
B) 0. 42
C) 4. 23
D) 0. 423
Answers
When 2.49 is multiplied by 0.17, the result (rounded to 2 decimal places) is 0.42. Therefore, the answer is option b) 0.42
To find the result of multiplying 2.49 by 0.17, we can simply multiply these two numbers together. Performing the multiplication, we get 2.49 * 0.17 = 0.4233.
Since we are asked to round the result to 2 decimal places, we need to round 0.4233 to the nearest hundredth. Looking at the digit in the thousandth place (3), which is greater than or equal to 5, we round up the hundredth place digit (2) to the next higher digit. Thus, the rounded result is 0.42.
Therefore, when 2.49 is multiplied by 0.17, the result (rounded to 2 decimal places) is 0.42, which corresponds to option B) 0.42.
Learn more about decimal places here:
https://brainly.com/question/20563248
#SPJ11
Help quickly this problem. Very important.

Answers
Answer:
It is translated left 6 units.
a museum room, circular in shape, has 5 equally spaced sensors on its walls. there are no dead or overlapping spots along the perimeter of the room. each sensor has the same angle of detection. what is the detection angle of each sensor?
Answers
According to the given information the detection angle is 72.
How would you define an angle?An angle is formed anytime two straight lines and rays meet at a single terminal. The intersection of two points at such an angle is known as the vertex. The word "angle" comes from the Latin word "angulus," which meaning "corner."
What is the math behind angles?Degrees are a measure representing circularity or rotation and are used to express angles. You would turn 360° in order to face back in the exact direction after completing a full revolution. As a result, a quarter-circle, or straight angle, is 90°, and a half-circle is 180°. The sum of any two or maybe more angles on the a straight line is 180°.
\(\begin{aligned}5\theta & =360^{\circ} \\\theta & =\frac{360^{\circ}}{6} \\& =72^{\circ}\end{aligned}\)
To know more about angle visit:
https://brainly.com/question/15277238
#SPJ4
nancy, an economist, would like to make the claim that the average weekly grocery budget for households in a small u.s. city is less than $253. nancy samples 18 households in the same city and obtains a sample mean of $229.60 spent on groceries per week.at the 2.5% significance level, should nancy reject or fail to reject the null hypothesis given the sample data below?
Answers
The P-value is greater than a, which means we should not reject the null hypothesis.
Therefore, Fail to reject the null hypothesis.
As per the given data,
We need to find out that should Nancy reject or fail to reject the null hypothesis given the sample data ?
According to the above stated question,
it is given that,
Nancy is an economist who would like to make the claim that the average weekly grocery budget for households in a u.s. city is less than $253.
No. of households in which Nancy take samples in the same city is 18.
Nancy obtains a sample mean of $229.60.
Nancy spent on groceries per week at the 2.5% significance level.
Now, according to the given above stated information:
The null and alternative hypotheses are
\(H_{0} =\) μ = 253
\(H_{a}=\)μ <253
Thus, this is a left-tailed test.
The significance level, \(\alpha\) = 0.05
The test statistic is t = - 0.75
The sample size, n = 18
The degrees of freedom is given by:
degrees of freedom=n - 1
Now, putting value of n we get:
=n - 1
= 18 - 1 = 17
P-value = P(\(t_{17}\) < - 0.75) = 0.2318
Therefore, the P-value is greater than a, which means we should not reject the null hypothesis.
Fail to reject the null hypothesis.
For more such questions on Null Hypothesis:
https://brainly.com/question/1626181
#SPJ4
Nancy, an economist, would like to make the claim that the average weekly grocery budget for households in a small U.S. city is less than $253. Nancy samples 18 households in the same city and obtains a sample mean of $229.60 spent on groceries per week.
At the 2.5% significance level, should Nancy reject or fail to reject the null hypothesis given the sample data below?
\(H_{0} =\): $253 per week: \(H_{a}=\): < $253) per week\(\alpha\)= 0.025 (significance level) test statistic = -0.75Use the graph below to select the type of test (left, right-, or two-tailed).
Then set the a and the test statistic to find the p-value. Use the results to determine whether to reject or fail to reject the null hypothesis.
Transcribed image text:
This activity is to ensure you know the difference in interpreting the value of a function f(x) vs. the derivative of a function f′(x). Part I: The time for a chemical reaction, T (in minutes), is a function of the amount of catalyst present, a (in milliliters), so T=f(a). a) Interpret with units f(5)=18 in terms of the chemical reaction. b) If f′(5)=−3 what are the units of 5 ? What are the units of −3 ? What does this statement tell us? Part II: The time that a turkey cooks is measured by y minutes for x pounds and is given by the function y=f(x). What are the units and meaning of f(10)=180 and f′(10)=20 ?
Answers
Interpreting functions and derivatives: understanding value and rate of change in chemical reactions and turkey cooking time.
Part I:
a) The function T(a) represents the time (in minutes) for a chemical reaction based on the amount of catalyst present (in milliliters). When we have f(5) = 18, it means that when the amount of catalyst is 5 milliliters, the time for the chemical reaction is 18 minutes. In other words, it indicates that with 5 milliliters of catalyst, the reaction takes 18 minutes to complete.
b) Here, f'(5) = -3 represents the derivative of the function f(a) at a = 5. The units of 5 are milliliters since it corresponds to the amount of catalyst. The units of -3 are minutes per milliliter because the derivative measures the rate of change of the function with respect to the input variable.
The statement f'(5) = -3 tells us that when the amount of catalyst is 5 milliliters, the time for the chemical reaction is changing at a rate of -3 minutes per milliliter. In practical terms, it implies that as we increase the amount of catalyst by 1 milliliter, the time for the reaction decreases by approximately 3 minutes. Conversely, if we decrease the amount of catalyst by 1 milliliter, the time for the reaction increases by approximately 3 minutes.
Part II:
In this case, the function y = f(x) represents the time (in minutes) it takes for a turkey to cook based on its weight, x, in pounds.
For f(10) = 180, it means that when the turkey weighs 10 pounds, it takes 180 minutes to cook. The units of f(10) are minutes, indicating the cooking time.
For f'(10) = 20, the units of 20 are minutes per pound, as it represents the rate of change of the cooking time with respect to the weight of the turkey. Therefore, it tells us that for every additional pound of turkey weight, the cooking time increases by approximately 20 minutes.
To summarize, f(10) = 180 means that a 10-pound turkey takes 180 minutes to cook, while f'(10) = 20 means that the cooking time increases by approximately 20 minutes for every additional pound of turkey weight.
Learn more about Function interpretation.
brainly.com/question/30597508
#SPJ11
HELPPPPP ASAP!!!! Thx!! and NOOO FILESSSS!!

Answers
Answer:
\(x=32\)°
Step-by-step explanation:
The law of sines is a property of all triangles that relates the sides and angles of a triangle. This property states the following:
\(\frac{sin(a)}{A}=\frac{sin(b)}{B}=\frac{sin(c)}{C}\)
Where side (A) is the side opposite angle (<a), side (B) is the side opposite angle (<b), and side (C) is the property opposite angle (<c).
Substitute each of the sides and respective angles into the formula, and solve for the unknown angle (<x). Please note that a triangle with two congruent sides (referred to as an isosceles triangle) has a property called the base angles theorem. This states that the angles opposite the congruent sides in an isosceles triangle are congruent. Therefore, there can be two (<x)'s in this triangle.
\(\frac{sin(a)}{A}=\frac{sin(b)}{B}=\frac{sin(c)}{C}\)
\(\frac{sin(x)}{10}=\frac{sin(116)}{17}=\frac{sin(x)}{10}\)
One can shorten the equation so it only holds the parts that will play a role in solving this equation,
\(\frac{sin(x)}{10}=\frac{sin(116)}{17}\)
Now take the cross product in this equation to simplify it further,
\(\frac{sin(x)}{10}=\frac{sin(116)}{17}\)
\(17(sin(x))=10(sin(116))\)
Inverse operations, solve this equation for (x),
\(17(sin(x))=10(sin(116))\)
\(sin(x)=\frac{10(sin(116))}{17}\)
\(x=sin^-^1(\frac{10(sin(116))}{17})\)
\(x=31.91782...\)
\(x\approx32\)
Answer:
hello,
Step-by-step explanation:
the triangle is isocele,
x+x+116=180
x=(180-116)/2
x= 32° (thirty-two , trente-deux in french)
a) Brigita is an IT technician. She is paid £24.17 per hour. a) Write down a formula for her total pay in pounds (P) if she works h hours.
b) use your formula to work out her total pay if she works five (5) hours.
Answers
After considering all the given data we come to the conclusion that the equation that will satisfy the given demand is P = 24.17× h and the total amount of money generated by Brigita when she works for 5 hours is £120.85.
Here we have to apply the principle of basic multiplication to derive the formula to evaluate the money earned by Brigita.
For the given case Brigita is paid £24.17 per hour. This means that for every hour she works, she earns £24.17.
To calculate her total pay (P) if she works h hours, we can use the formula:
P = 24.17× h
In the given case that Brigita works 5 hours, we could apply a substitution along h with 5 in the formula:
P = 24.17 × 5 = £120.85
So her total pay would be £120.85.
To learn more about multiplication
https://brainly.com/question/29793687
#SPJ1
A stream of crude oil has a molecular weight of 4.5x10² kg/mol and a mean average boiling point of 370 °C. Estimate the followings: 1. The crude specific gravity at 60 °F? 2. The crude gravity (API°) at 60 °F? 3. Watson characterization factor? 4. Refractive index? 5. Surface tension? 6. Is this crude oil paraffinic, naphthenic or aromatic? Explain, briefly and qualitatively.
Answers
The crude oil is likely to be paraffinic. Paraffinic crude oils are characterized by having a high API°, low Watson characterization factor, and low refractive index. They also tend to have a high surface tension.
Specific gravity at 60 °F: 0.88
API° at 60 °F: 28
Watson characterization factor: 1.014
Refractive index: 1.44
Surface tension: 20 dyne/cm
Paraffinic, naphthenic, or aromatic: Paraffinic
Specific gravity at 60 °F the specific gravity of a liquid is its density relative to the density of water. The specific gravity of crude oil is typically between 0.8 and 1.0. A specific gravity of 0.88 means that the crude oil is 88% as dense as water.
API° at 60 °F: The API°, or American Petroleum Institute gravity, is a measure of the lightness or darkness of crude oil. A higher API° indicates a lighter crude oil. A crude oil with an API° of 28 is considered to be a medium-heavy crude oil.
Watson characterization factor the Watson characterization factor is a measure of the aromaticity of crude oil. A higher Watson characterization factor indicates a more aromatic crude oil. A crude oil with a Watson characterization factor of 1.014 is considered to be a paraffinic crude oil.
Refractive index the refractive index of a liquid is a measure of how much light is bent when it passes through the liquid. The refractive index of crude oil is typically between 1.4 and 1.5. A refractive index of 1.44 indicates that the crude oil is slightly more refractive than water.
Surface tension the surface tension of a liquid is a measure of the force that acts at the surface of the liquid, tending to minimize the surface area. The surface tension of crude oil is typically between 20 and 30 dyne/cm. A surface tension of 20 dyne/cm indicates that the crude oil has a relatively high surface tension.
Based on the estimated values, the crude oil is likely to be paraffinic. Paraffinic crude oils are characterized by having a high API°, low Watson characterization factor, and low refractive index. They also tend to have a high surface tension.
Learn more about tension with the given link,
https://brainly.com/question/138724
#SPJ11
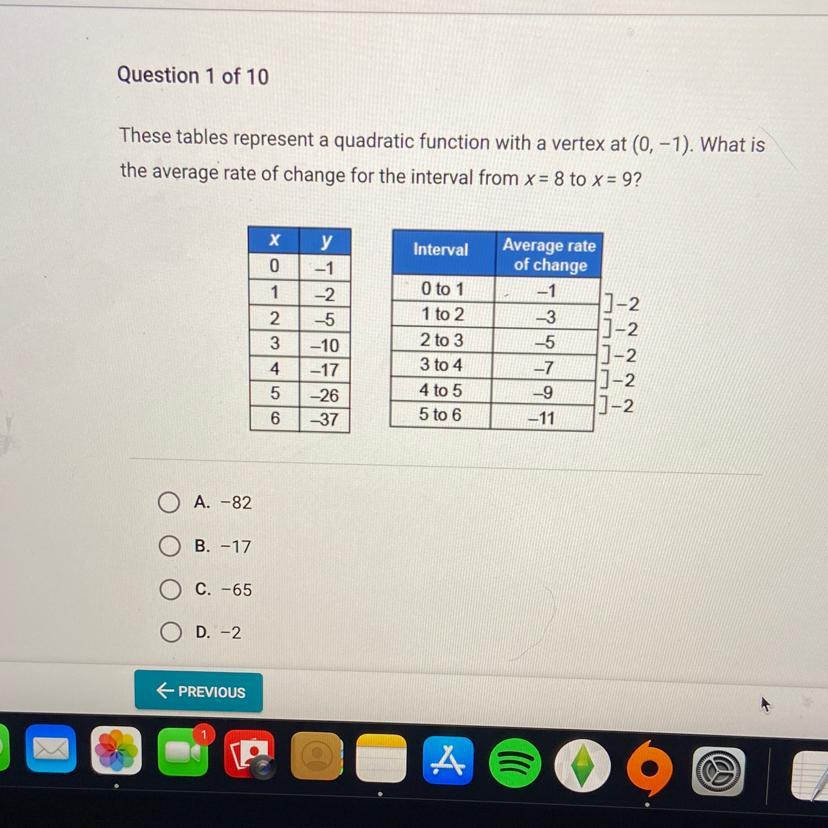
These tables represent a quadratic function with a vertex at (0, -1). What is
the average rate of change for the interval from x = 8 to x = 9?
OA. -82
OB. -17
C. -65
D. -2
x y
0
1
23456
125
-10
-17
-26
-37
Interval
0 to 1
1 to 2
2 to 3
3 to 4
4 to 5
5 to 6
Average rate
of change
-1
-3
-5
-7
-9
-11
3-2
1-2
3-2
1-2
3-2
Answers
Answer:
B
Step-by-step explanation:
We can notice that based on the given pattern, the average rate of change from \(x=n\) to \(x=n+1\), where \(n \in \mathbb{Z}\), is \(-2n-1\).
So, the average rate of change from \(x=8\) to \(x=9\) is \(-2(8)-1=-17\).
The rate of change for the interval from x = 8 to x = 9 is -17.
What is Rate of change?The pace at which one quantity changes in relation to another quantity is known as the rate of change function. Simply said, the rate of change is calculated by dividing the amount of change in one item by the equal amount of change in another.
The relationship defining how one quantity changes in relation to the change in another quantity is given by the rate of change formula.
We know the standard form of quadratic function
y = a (x - b)² + c with vertex (b, c)
Since the vertex is (0, -1) then b= 0, c= -1.
Then, the quadratic equation is:
y= ax² - 1 passes through (1, 2), (2, -5),.....
So, the rate of change in interval (8, 9) is
= y(9)- y(8)/ (9-8)
= -9² -1 - 8² - 1/ 1
= -17.
Learn more about Rate of change here:
https://brainly.com/question/29518179
#SPJ5