Qualitative data a. can not be numeric b. indicate either how much or how many c. must be nonnumeric d. are labels used to identify attributes of elements
Answers
Qualitative data is a non-numerical, descriptive data that indicates the properties of an element or population. This kind of data cannot be expressed in a numerical form, and thus, must be non-numeric. Qualitative data represents the labels that identify the attributes of the elements or the population. Qualitative data is descriptive and usually takes on the form of a label or a name.
Some examples of qualitative data include names, colors, and flavors. It is the opposite of quantitative data, which is numerical and expresses how much or how many.In qualitative research, the researcher aims to understand and interpret social phenomena. They do this by gathering data through unstructured or semi-structured techniques such as interviews, observations, or surveys. This type of research usually involves a smaller sample size, as the data gathered is more in-depth and detailed.
Qualitative data is essential in social science research, where understanding complex social phenomena requires a deep understanding of the behaviors, attitudes, and perceptions of the participants involved. It can also be used in other fields such as marketing, education, and healthcare to understand customer preferences, attitudes, and behaviors. In conclusion, qualitative data are non-numerical and descriptive data that indicate the attributes of an element or population. It is used in social science research, and its purpose is to understand and interpret social phenomena.
To know more about descriptive visit:
https://brainly.com/question/32171827
#SPJ11
Related Questions
help !!!! I will mark as brainliest 20 points !!!! solve both of question !!!
pls show work !!! thanks

Answers
Answer:
a). k ≥ -1
b). k < 5
c). k ≤ 2
d). k > -3
Step-by-step explanation:
a). k ≥ - 3 + 2
k ≥ -1
b). 3k < 16 - 1
3k < 15
k < 5
c). -4k ≥ -5 - 3
4k ≤ 8
k ≤ 2
d). 3k > -10 + 1
3k > -9
k > -3
Answer:
solution is on the picture attached
Hope this helps

For a physics experiment, the class drops a golf ball of a bridge toward the pavement below. The bridge is 75 feet high. The function h = -16+75 gives the golf ball's height h above the pavement (in feet) after 1 seconds. Graph the function. How many seconds does it take for the golf ball to hit the pavement?
Answers
It will take the golf ball some amount of time to hit the pavement.
Using the function h = -16t^2 + 75, where t is the time in seconds since the ball was dropped. To graph this function, we can plot points on a coordinate grid. For example, when t = 0, the ball is at its initial height of 75 feet, so we plot the point (0, 75). When t = 1, we can use the function to find that the ball is at a height of h = -16(1)^2 + 75 = 59 feet. So we plot the point (1, 59). Continuing in this way, we can plot more points and connect them to form a smooth curve.
To find out how many seconds it takes for the golf ball to hit the pavement, we need to find the time t when the height h is equal to 0 (since the ball will have hit the pavement by then). So we set h = 0 in the function and solve for t:
0 = -16t^2 + 75
16t^2 = 75
t^2 = 75/16
t = sqrt(75/16) ≈ 1.85
Therefore, it will take about 1.85 seconds for the golf ball to hit the pavement.
Learn more about coordinate grid: https://brainly.com/question/29000992
#SPJ11
A rectangular garden's length is 12 feet longer than its width. Write a function for the garden's perimeter
Answers
The function for the garden's perimeter in terms of the width "w" would be P(w) = 4w + 24
What is the perimeter?
The perimeter is a mathematical term that refers to the total distance around the outside of a two-dimensional shape. It is the length of the boundary or the sum of the lengths of all the sides of a closed figure.
Let's call the width of the rectangular garden "w".
According to the problem, the length of the garden is 12 feet longer than its width. So, the length would be w + 12.
The perimeter is the sum of all four sides of the rectangular garden. So,
Perimeter = w + w + (w + 12) + (w + 12)
Simplifying this expression, we get:
Perimeter = 4w + 24
Therefore, the function for the garden's perimeter in terms of the width "w" would be P(w) = 4w + 24.
To learn more about the perimeter visit:
brainly.com/question/397857
#SPJ4
As a candle burns, it decreases in height by 2 inches every hour. If the candle is 12 inches tall when it is lit, how will the height change over time? If the candle burned for 2 hours, how many inches tall will it be?
Answers
The height of the candle change over time as a linear function
How will the height of the candle change over time?The height of the candle decreases by 2 inches every hour, which means the height of the candle is a linear function of time.
We can write the equation for the height of the candle as:
h(t) = 12 - 2t
where h is the height of the candle in inches and t is the time in hours.
If the candle burned for 2 hours, we can plug in t=2 into the equation to find the height:
h(2) = 12 - 2(2) = 8
So the height of the candle after 2 hours of burning is 8 inches.
Read more about linear relation at
https://brainly.com/question/30318449
#SPJ1
Write an expression to describe the sequence below. Use n to represent the position of a term in the sequence, where n = 1 for the first term. -91, -194, -291, -388
Answers
Answer:
\(T_n = -97n\)
Step-by-step explanation:
The actual sequence is: -97, -194, -291, -388
Required
Determine the nth term
nth term of an arithmetic progression is:
\(T_n = T_1 + (n - 1)d\)
Where
\(T_1 = First\ Term = -97\)
\(d = Common\ Difference = -194 - (-97) =-97\)
So:
\(T_n = T_1 + (n - 1)d\) becomes
\(T_n = -97 + (n - 1) * -97\)
\(T_n = -97 -97n + 97\)
Collect Like Terms
\(T_n = -97n + 97-97\)
\(T_n = -97n\)
Hence, a term of the sequence is:
\(T_n = -97n\)
if 124 people attend a concert and tickets for adults cost $2.5 while tickets for children cost $2 and total receipts for the concert was $274, how many of each went to the concert?
Answers
Answer: (52,72) (52 adults and 72 children)
Step-by-step explanation:
1) Create 2 formulas that show the information show above, 2.5x+2y=274 showing how the x is how many adults paid for a ticket and y is how many children paid for a ticket. The second formula being x+y=124 meaning the combination of children and adults equal 124 people.
2) Take those formulas and input them into a graph as shown in the picture (Graph 1)
3) Find the common point shown on the graph (should be the middle grey dot if on Desmos as shown (Graph 2)
4) The common point is (52, 72)
And if you plug these numbers in by replacing the x's and y's i shown in step 1 they bring an output of 274 and 124
Hope this helps :)


If the original quantity is 8 and the new quantity
is 2, what is the percent decrease?
Answers
If the original quantity is 8 and the new quantity is 2, then the correct answer is 75%.
How did we figure this out?
For this question we need to subtract and multiply the numbers. We know that 2 = 25% of 8 so:
\(\boxed{8-2=6}\\\boxed{6/2=3}\)
We are going to take that 25% and multiply it with 3 to get are final answer.
What is the missing number of 25 and 3?\(\boxed{25*3=75}\\\boxed{So,2=75}\)
Therefore, If the original quantity is 8 and the new quantity is 2, then the correct answer is 75%.
Suppose an item cost $6.99 in 1977. What would have been the equivalent cost in 2017?

Answers
Explanation:
In 1977, the cpi value is
\(60.600\)In 2017 the cpi value is
\(245.120\)To figure out the cost of the value in 2017, we will interpolate below
\(\begin{gathered} 60.600=245.120 \\ 6.99=x \\ cross\text{ multiply, we will have} \\ 60.6x=6.99\times245.120 \\ \frac{60.6x}{60.6}=\frac{1713.3888}{60.6} \\ x=28.27 \end{gathered}\)Hence,
The final answer is
\(\Rightarrow\text{ \$}28.27\)PLS ANSWER I NEED HELPPP i have posted this question several times, i am desperate for an answer pls
right angle trigonometry

Answers
Answer:
Step-by-step explanation:
Triangle A)
use Pythagoras to find hypotenuse
c =\(\sqrt{a^{2} +b^{2} }\)
c = √(\(8^{2}\)+\(5^{2}\))
c= √(64+25)
c=√89
c=9.43398 mm
it's a right triangle so just find one angle using SOH CAH TOA to remember how the trig function fit on the triangle
Tan(Ф) = Opp / Adj
Ф = arcTan( 5/8)
Ф = 32.0053°
Ф = 32° approx
∠A=Ф
∠B= 180-90-32
∠B=58°
Triangle B)
∠L = 180-90-72
∠L = 18°
use CAH
Cos(Ф) = Adj / Hyp
Cos(18) =Adj / 10
10 * Cos(18) = Adj
9.51056 cm = Adj
KL = Adj
now use SOH
Sin(Ф) = Opp / Hyp
Sin(18) =Opp / 10
10* Sin(18) = Opp
3.09016 cm = Opp
KJ = Opp
Triangle C)
∠Q = 180-90-42
∠Q = 48°
CAH
Cos(∅) = Adj / Hyp
Cos(48) = 13 / Hyp
Hyp = 13 / Cos(48)
Hyp = 19.4281 km
QS = Hyp
SOH
Sin(∅) = Opp / Hyp
Sin(48) = Opp / 19.4281
19.4281 * Sin(48) = Opp
14.4379 Km = Opp
Opp = RS
Using SOH CAH TOA is super helpful to place the trig function onto a triangle :)
Find the x-intercept of the equation 3x - 5y = -30
Answers

Solve |x+ 4| = -6 im struggling with this problem
Answers
Recall that:
\(\begin{gathered} |a|\ge0 \\ \text{for all a}\in\R. \end{gathered}\)Therefore the given equation has no solutions.
Answer: Option B.
Write the statement "--5 times x subtracted from 22 is less than 9 more than
x" as a mathematical inequality. I dont know this sorry
Answers
Answer:
5x-22<9<x
Step-by-step explanation:
5 times x = 5x
subtract 22 = 5x-22
less then = 5x-22<
9 more then x = 5x-22<9<x
The cost y (in dollars) to spend an evening bowling is proportional to the number of games x that are bowled. It costs $16 to bowl 4 games. Write an equation that represents the situation.
Answers
Hi , please help and explain fully ! ( I don't understand how to work this one out)

Answers
2. Now we subtract 1 from both sides.
x+1=12x+30
-1 -1
x=12x+29
3. Now subtract 12x from each side.
x=12x+29
-12x -12x
-11x=29
4. Now we need to divide both sides by the same factor.
-11x/-11=29/-11
X= -29/11
If you need more help I can draw it out on paper for you:))
The depth of the water in a tank after t minutes is modeled by f(t) = 5+1.5t, measured in inches. Find and interpret (10).
A) 20; The depth of the water after 10 minutes is 20 inches.
B) 20; The water reaches a depth of 10 inches after 20 minutes.
C) 65; The depth of the water after 10 minutes is 65 inches.
D) 65; The water reaches a depth of 10 inches after 65 minutes.
Answers
The depth of water after 10 minutes is 20 inches which is the option A.
Given that:-
Depth of water in a tank after t minutes is modeled by the function f(t) = 5+1.5t, measured in inches.
Let us check for Option A.
Putting t = 10 min in the given equation, we get,
f(10) = 5 + 1.5*10 = 5 + 15 = 20 inches.
Hence, option A is the right answer.
We can also check the other options to confirm our answer.
Putting t = 20 minutes,
f(20) = 5 + 1.5*20 = 5 + 30 = 35 inches
As 10 inches is written in B, hence, it is not the right answer.
We already know that t = 10 gives 20 inches.
Hence, C is not the answer.
Putting t = 65 minutes,
f(20) = 5 + 1.5*65 = 5 + 97.5 = 102.5 inches
As 10 inches is written in D, hence, it is not the right answer.
To learn more about function, here:-
https://brainly.com/question/12431044
#SPJ1
(2a^3)^4(a^3)^3
simplify
Answers
Answer:
16a^21
Step-by-step explanation:

Answer:
16 a^21
Step-by-step explanation:
= (2a^3)^4 (a^3)^3
= (2^4 a^3*4) (a^3*3)
= (16a^12) (a^9)
= 16 a^21
Help pleaseeeee I’m confused
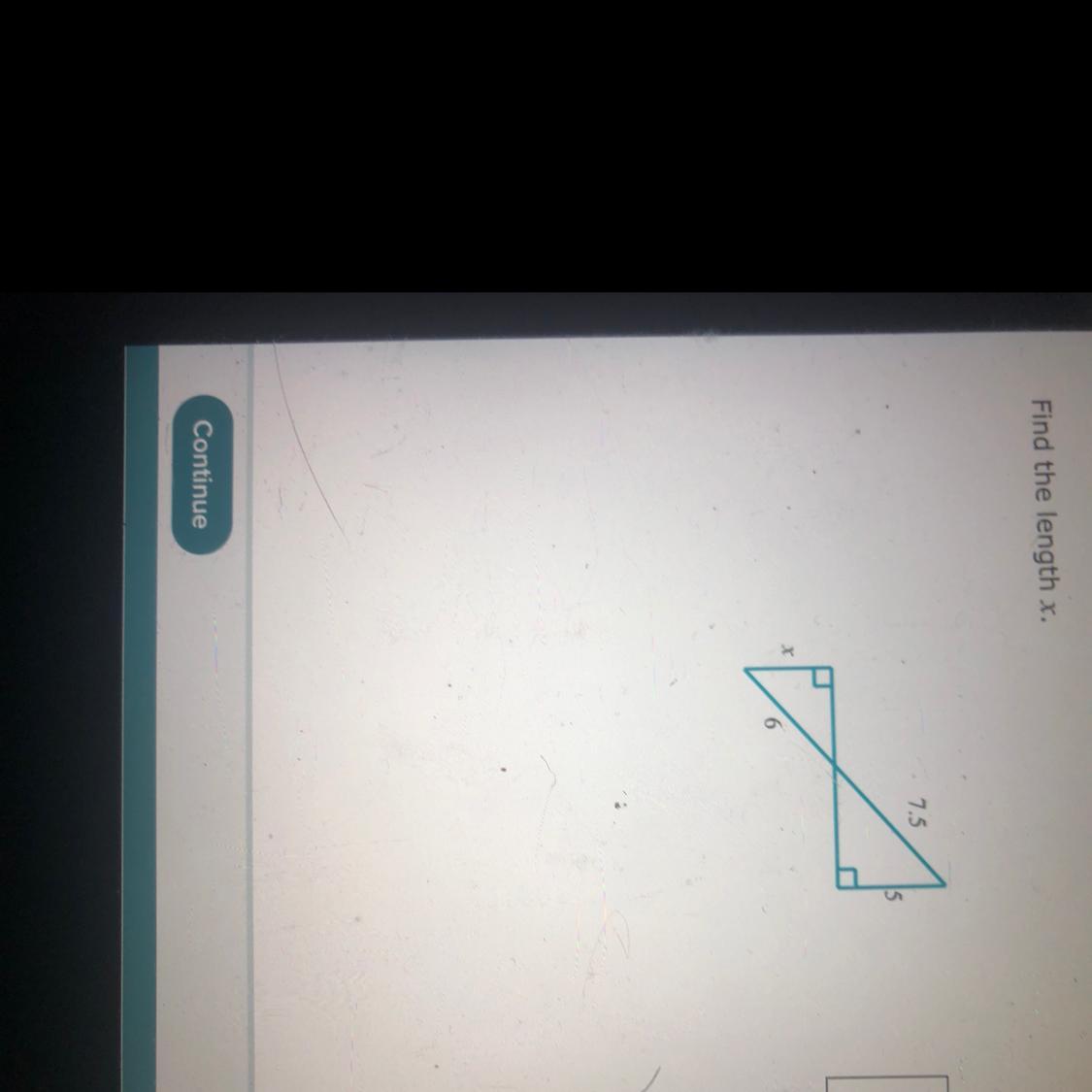
Answers
Step by step solution:
Since it’s a scale copy the equation is 6/7.5=x/5
6/7.5=0.8
5*0.8=4
x=4
Hope that helps:)
answer i cant figure this out

Answers
Answer:
I would say it's 8 because if the y=8 so if 8 is there you times it by 2 to get 16 so now you get 2 from x the exponent is 4 so 4x2 is 8 so 16-8=8 if that's wrong it's probable -8 because I haven't done exponents in a long time
help me please help me please

Answers
3 =15
7=35
1=5
Constant of 1 is 5
there are 30 red marble and 150 blue marblee in a box what is the ratio of blue marblee tonred marbles
Answers
Answer: 150:30
Step-by-step explanation: First, put 150. Next, put a : sign. Lastly, put the number 30. Hopefully this helped! :D
a high school has 44 players on the football team. the summary of the players' weights is given in the box plot. approximately, what is the percentage of players weighing less than or equal to 229 pounds?
Answers
Approximately 62.5% of the 44 players or 27.75 players, weigh less than or equal to 229 pounds.
Given the information from the box plot, we can estimate the percentage of players weighing less than or equal to 229 pounds. First, we need to find the number of players by dividing the summary of weights by the weight of one player: 44 players. Next, we can estimate the number of players weighing less than or equal to 229 pounds by finding the percentage of the data that is below 229 pounds and multiplying it by the total number of players. Based on the quartiles given in the box plot, we know that 50% of the data is below 214 pounds and 75% of the data is below 253 pounds. We can estimate that approximately 62.5% of the data is below 229 pounds by taking the average of these two values: (50% + 75%) / 2 = 62.5%. So, approximately 62.5% of the 44 players, or 27.75 players, weigh less than or equal to 229 pounds
To lean more about quartiles click here
brainly.com/question/15642066
#SPJ4
Complete Question
a high school has 44 players on the football team. the summary of the players' weights is given in the box plot. approximately, what is the percentage of players weighing less than or equal to 229 pounds?
The box Plot, the minimum weight is 154 pounds. The first quartile Q1 is 159 which 25% of the data. The second Quartile Q2 is 214 which 50% of the data. The third Quartile Q3 is 253 which is 75% and the maximum weight is 268.

compute the convergence set for the following power series. use interval notation for your answers.
[infinity]
Σ xn/(n+1)2n converges for
n=0
[infinity]
Σ (x-1)n/nconverges for
n=1
[infinity]
Σ (x-n)n/n! converges for
n=1
[infinity]
Σ (x+1)n/3n converges for
n=17
Answers
Thus, the series converges for -4 < x < 2.In interval notation, the convergence sets are:
1)(-2, 2)
2)(-∞, ∞)
3)(-∞, ∞)
4)(-4, 2)
1) The power series ∑(n=0)∞ xn/(n+1)^(2n) converges for all x in (-1,1) by the ratio test.
2) The power series ∑(n=1)∞ (x-1)^n/n converges for x in the interval (0,2), with the endpoints excluded. To see this, we can use the ratio test and find that |(x-1)/(n+1)| → |x-1| as n → ∞. Thus, the series converges absolutely when |x-1| < 1, and diverges when |x-1| > 1. At x = 0, the series is the harmonic series which diverges, and at x = 2, the series becomes the alternating harmonic series which converges but not absolutely.
3) The power series ∑(n=1)∞ (x-n)^n/n! converges for all x in (-∞,∞). We can use the ratio test and find that |(x-n)/(n+1)| → 0 as n → ∞, and thus the series converges absolutely for all x.
4) The power series ∑(n=1)∞ (x+1)^n/3^n converges for x in the interval (-4,2) by the ratio test. When x = -4, the series becomes ∑(-1)^n/3^n which converges by the alternating series test. When x = 2, the series becomes ∑3^n which diverges.
For such more questions on Series converges:
https://brainly.com/question/15415793
#SPJ11
The series converges if |x+1|/3 < 1, i.e. if -4 < x < 2. The series converges at x=2 and diverges at x=-4, so the convergence set is (-4,2].
For the first power series, we use the ratio test:
lim |(x_{n+1}/(n+2)^{2(n+2)})/(x_n/(n+1)^{2n+2})| = lim |x_{n+1}|(n+1)^{2n+3}/|x_n|(n+2)^{2n+2}
= lim |x_{n+1}/x_n| ((n+1)/(n+2))^{2n+3} (n+1)/(n+2)
= lim |x_{n+1}/x_n| lim ((n+1)/(n+2))^{2n+3} lim (n+1)/(n+2)
= |x| lim (1/4)^n lim 1/2 = 0
Therefore, the series converges for all x.
For the second power series, we also use the ratio test:
lim |((x-1)^(n+1)/(n+1))/((x-1)^n/n)| = lim |x-1| (n+1)/n = |x-1|
Therefore, the series converges if |x-1| < 1 and diverges if |x-1| > 1. The series converges at x=0 and x=2, so the convergence set is [0,2].
For the third power series, we use the ratio test again:
lim |((x-(n+1))/(n+1)) ((x-n)/n!)| = lim |x-(n+1)|/|n+1| lim |x-n|/n! = 0
Therefore, the series converges for all x.
For the fourth power series, we use the root test:
lim sup |(x+1)/3|^n = |x+1|/3
Know more about convergence set here:
https://brainly.com/question/16407117
#SPJ11
if axis deviation is present, which leads that normally have positive qrs complexes will have negative qrs complexes?
Answers
If there is an axis deviation, it can result in a reversal of the QRS complex polarity in certain leads. Leads that normally have positive QRS complexes may have negative QRS complexes, and vice versa.
For example, if there is a left axis deviation, leads I and aVL, which normally have positive QRS complexes, may have negative QRS complexes.
Axis deviation refers to the direction in which the electrical activity is traveling through the heart. If the electrical activity is deviated from its normal pathway, it can cause a change in the orientation of the QRS complex, which is a graphical representation of the electrical activity generated by the ventricles during the cardiac cycle.
The change in polarity occurs because the direction of the electrical activity is now opposite to what it would be in a normal heart. This change in polarity can be used to help diagnose the location and type of axis deviation. Understanding the significance of the polarity changes can help healthcare providers determine appropriate treatment plans for patients.
Learn more about polarity here:
https://brainly.com/question/1946554
#SPJ4
-3 + 17p + 2 -p, simplify expression by combining like terms
Answers
Answer:
16p-1
Step-by-step explanation:
-3+17p+2-p
-1+16p
Answer:
16p - 1
Step-by-step explanation:
= -3 + 17p + 2 - p
= 17p - p - 3 + 2
= 16p - 1
Which value, if any, from the set {4, 5, 6} makes 15f = 85 true?
A. 5
B. 4
C. None of these values
D. 6
Answers
Volume of a cube (cm') = width (cm) x height (cm) x length (cm). 1.1) Using the equation above, determine the volume of a cube that measures 3 cm wide, 3 cm tall, and 3 cm long. 1.2) Let's say this cube is made out of ice and has a mass of 24.76 grams (g). What is this ice cube's density? 1.3) The density of liquid water is slightly higher than that of frozen water ice. Liquid water's density at standard pressures and temperatures is 1.00 grams per cubic centimeter (g/cm'). Given that density, what is the mass of a cube of water measuring 3 cm wide, 3 cm tall, and 3 cm long? 1.4) Compare the weight of the water you calculated in question 1.3 with the weight of the ice of the same volume given in question 1.2. Which is heavier, the liquid water or the ice? Notice that the cube of water is the same size (or volume) as the cube of ice. 1.5) You know that ice floats on water. Explain why.
Answers
1.1) The volume of the cube is 27 cubic centimeters. 1.2)the density of the ice cube is approximately 0.917 grams per cubic centimeter (g/cm³).
1.3) the mass of the water cube is 27 grams. 1.4) the weight of the water and the ice would be the same under the same conditions. 1.5)In simpler terms, ice floats on water because it is lighter (less dense) than the water, allowing it to displace an amount of water equal to its weight and float on the surface.
1.1) The volume of the cube can be calculated using the equation: Volume = width x height x length. In this case, the cube measures 3 cm wide, 3 cm tall, and 3 cm long, so the volume is:
Volume = 3 cm x 3 cm x 3 cm = 27 cm³.
Therefore, the volume of the cube is 27 cubic centimeters.
1.2) Density is defined as mass divided by volume. The mass of the ice cube is given as 24.76 grams, and we already determined the volume to be 27 cm³. Therefore, the density of the ice cube is:
Density = Mass / Volume = 24.76 g / 27 cm³ ≈ 0.917 g/cm³.
Therefore, the density of the ice cube is approximately 0.917 grams per cubic centimeter (g/cm³).
1.3) The volume of the water cube is the same as the ice cube, which is 27 cm³. Given the density of liquid water as 1.00 g/cm³, we can calculate the mass of the water cube using the equation:
Mass = Density x Volume = 1.00 g/cm³ x 27 cm³ = 27 grams.
Therefore, the mass of the water cube is 27 grams.
1.4) The weight of an object depends on both its mass and the acceleration due to gravity. Since the volume of the water cube and the ice cube is the same (27 cm³), and the mass of the water cube (27 grams) is equal to the mass of the ice cube (24.76 grams), their weights would also be equal when measured in the same gravitational field.
Therefore, the weight of the water and the ice would be the same under the same conditions.
1.5) Ice floats on water because it is less dense than liquid water. The density of ice is lower than the density of water because the water molecules in the solid ice are arranged in a specific lattice structure with open spaces. This arrangement causes ice to have a lower density compared to liquid water, where the molecules are closer together.
When ice is placed in water, the denser water molecules exert an upward buoyant force on the less dense ice, causing it to float. The buoyant force is the result of the pressure difference between the top and bottom surfaces of the submerged object.
In simpler terms, ice floats on water because it is lighter (less dense) than the water, allowing it to displace an amount of water equal to its weight and float on the surface.
Learn more about cube;
brainly.com/question/15420947
#SPJ4
Define the domain of the following:
{-2, -1, 0, 2, 5}
{-2, -1, 0, 1, 2, 3, 4, 5}
All Real Numbers
{3, -1, 3, 1, 2}

Answers
The domain of the relation in the graph is:
{-2, -1, 0, 2, 5}
How to define the domain for the graph?A relation maps elements from one set (the domain) into elements from another set (the range).
Such that the domain is represented in the horizontal axis.
In the graph, we can see the points:
{(-2, -3), (-1, -1), (0, 3), (2, 1), (5, 2)}
The domain is the set of the first values of these points, then the domain is:
{-2, -1, 0, 2, 5}
The correct option is the first one.
Learn more about domains at:
https://brainly.com/question/1770447
#SPJ1
second time asking , pls help ! i’ll give points

Answers
Answer:
Don't have the ability to graph here but graph as follows:
X=1, Y=4
X=2, Y=8
X=3, Y=12
etc....etc....etc
The points will be proportional so your line will be straight.
Step-by-step explanation:
a rectangular poster is to contain 200 square inches of print. the margins at the top and bottom of the poster are to be 2 inches, and the margins on the left and right are to be 1 inch. what should the dimensions of the poster be so that the least amount of poster is used?
Answers
dimensions of the poster with margin are L = 12 in and h = 24 in
What is the area of a rectangular poster?The shape/polygon of a rectangle is two dimensional, having four sides, four vertices, and four right angles. The rectangle's two opposing sides are equal and parallel to one another. The space a rectangle occupies is known as its area. The area of a rectangle can also be defined as the region inside its border.
We utilise the unit squares to calculate a rectangle's area. Rectangle ABCD should be divided into unit squares. The total number of unit squares that make up a rectangle ABCD is its area.
Rectangle area equals length times width.
SolutionLet call length of printed area of the poster be " x " and height of printed area of the poster be " y ".
Area of the poster = length and height
200 = x*y
y = 200/x
We also know that dimensions of the poster with margin is:
L = x + 2 in and H = y + 4 in
Therefore area of the poster is:
A(p) = ( x + 2 ) * ( y + 4 )
And area as function of x is:
A(x) = ( x + 2 ) * ( 200/x + 4 )
A(x) = 200 + 4*x + 400 /x + 8
Taking derivatives on both sides of the equation we have:
A´(x) = 4 - 400/x²
By taking A´(x) = 0
4 - 400/x² = 0 ⇒ 4*x² - 400 = 0
x² = 400 / 4
x² = 100
x = 10 in
and y = 200/x ⇒ y = 20
The second derivative A´´(x) = 400/x4 which is > 0
there is a minimum for the function at the point x = 10
As x and y are dimensions of the printing area of the poster, dimensions of the poster with margin are
L = x + 2 = 10 + 2 = 12 in and
h = y + 4 = 20 + 4 = 24 in
to learn more about area of rectangle visit:
brainly.com/question/28700994
#SPJ4
Kara had 350 in his bank account.His bank account charges a fee of 7.50 each month.If he makes no other deposits or withdrawals. how much money in Kara's account at the end of the month?
Answers
The amount of money in Kara's account at the end of the month is 342.50.
How much money in Kara's account at the end of the month?The amount of money in Kara's account would be reduced by the amount of money that the bank fee charged by the bank.
In order to determine the amount of money in the account at the end of the month, subtract the amount of bank fee from the amount of money in the account.
Subtraction is the mathematical operation that is used to determine the difference between two or more numbers. The sign used to represent subtraction is -. Other mathematical operations are addition, division and multiplication.
Money in the account at the end of the month = amount in the account - bank fee
350 = 7.50 = 342.50
To learn more about subtraction, please check: https://brainly.com/question/854115
#SPJ1