the name Joe is very common at a school in one out of every ten students go by the name. If there are 15 students in one class, what is the probability that at least one of them is named Joe
Answers
Using the binomial distribution, it is found that there is a 0.7941 = 79.41% probability that at least one of them is named Joe.
For each student, there are only two possible outcomes, either they are named Joe, or they are not. The probability of a student being named Joe is independent of any other student, hence, the binomial distribution is used to solve this question.
Binomial probability distribution\(P(X = x) = C_{n,x}.p^{x}.(1-p)^{n-x}\)
\(C_{n,x} = \frac{n!}{x!(n-x)!}\)
The parameters are:
x is the number of successes. n is the number of trials. p is the probability of a success on a single trial.In this problem:
One in ten students are named Joe, hence \(p = \frac{1}{10} = 0.1\).There are 15 students in the class, hence \(n = 15\).The probability that at least one of them is named Joe is:
\(P(X \geq 1) = 1 - P(X = 0)\)
In which:
\(P(X = x) = C_{n,x}.p^{x}.(1-p)^{n-x}\)
\(P(X = 0) = C_{15,0}.(0.1)^{0}.(0.9)^{15} = 0.2059\)
Then:
\(P(X \geq 1) = 1 - P(X = 0) = 1 - 0.2059 = 0.7941\)
0.7941 = 79.41% probability that at least one of them is named Joe.
To learn more about the binomial distribution, you can take a look at https://brainly.com/question/24863377
Related Questions
The circumference of a circle is 100.48 millimeters. What is the circle's radius?
Answers
Answer:
r=15.99
Step-by-step explanation:
r=C
2π=100.48
2·π≈15.99189
Answer:
15.99 or 16
Step-by-step explanation:
Plsssssss help and no one send me a file just tell me the answer

Answers
Either F or J
I think it's J, but it might be F :|
HELPP PLS FAST MATH MATH MATH

Answers
The answer to each part is given above.
What are algebraic expressions?In mathematics, an expression or mathematical expression is a finite combination of symbols that is well-formed according to rules that depend on the context.Mathematical symbols can designate numbers (constants), variables, operations, functions, brackets, punctuation, and grouping to help determine order of operations and other aspects of logical syntax.Given are the functions as follows -
p(x) = - 5x + 5
q(x) = - 4x - 2
We can write -
(p о q)(5) = (- 5x + 5)(- 4x - 2)
(p о q)(5) = - 5x(- 4x - 2) + 5(- 4x - 2)
(p о q)(5) = 20x² + 10 - 20x - 10
(p о q)(5) = 20x² - 20x
(p о q)(5) = 20 x 25 - 20 x 5
(p о q)(5) = 500 - 100
(p о q)(5) = 400
(p о q)(5) = - 5x(- 4x - 2) + 5(- 4x - 2) = (q о p)(5) = 400
(q о p)(5) = 400
Therefore, the answer to each part is given above.
To solve more questions on algebraic expressions, visit the link below -
brainly.com/question/1041084
#SPJ1
Let (-3,-2) be a point on the terminal side of theta. Find the csc(theta)
Answers
The csc(theta) = 1/sin(theta) = 1/(-2/√13) = -√13/2. So, the cosecant of theta is -√13/2.
To find the cosecant (csc) of theta when a point (-3, -2) lies on its terminal side, we need to determine the hypotenuse (r) of the right triangle formed by this point.
Using the Pythagorean theorem, r^2 = (-3)^2 + (-2)^2. So, r^2 = 9 + 4 = 13, and r = √13.
The csc(theta) is the reciprocal of sin(theta). Sin(theta) is calculated as the ratio of the opposite side (y-coordinate) to the hypotenuse, or sin(theta) = -2/√13.
To learn more about : cosecant
https://brainly.com/question/31708994
#SPJ8
solve for x x/6+5=4
x=
Answers
Answer: 30/23
Step-by-step explanation:
Step 1: Simplify both sides of the equation.
Step 2: Subtract 4x from both sides.
Step 3: Subtract 5 from both sides.
Step 4: Multiply both sides by 6/(-23).
lolz have a nice day!!!
Answer:
Multiply the whole equation to 6 to make it simple.
So you get:
X + 30 = 24
So: X = 24 - 30 = ( - 6 )
Given m∠14+m∠3=180° . Which lines are parallel, if any must be parallel, based on the given information? a∥b by the converse of the alternate interior angles theorem. c∥d by the converse of the corresponding angles theorem. a∥b by the converse of the same-side interior angles theorem. Not enough information to make a conclusion.
Answers
Answer:
c∥d by the converse of the corresponding angles theorem.
Step-by-step explanation:
giving brainliest!!!!!!!!!!!!!!!!!!!!!

Answers
Answer:
The function has 4 roots.
Step-by-step explanation:
Since the degree of the polynomial has is 4, there are a total of 4 roots (both real and complex).
Answer:
4 roots, two real and two complex.
Step-by-step explanation:
The degree of the polynomial indicates the number of solutions
Hope this helps
Help me verify this identity.Follow the structure from the notes by identify the properties used in each step.

Answers
Given that you have to verify that:
\(\frac{1}{1-sinx}+\frac{1-sinx}{cos^2x}=2sec^2x\)You need to follow these steps, in order to solve the exercise:
1. You need to use this Pythagorean Identify to rewrite the denominator of the second fraction:
\(cos^2x+sin^2x=1\)If you solve for:
\(cos^2x\)You get:
\(cos^2x=1-sin^2x\)Then, you can rewrite the expression on the right side in this form:
\(\frac{1}{1-sinx}+\frac{1-sinx}{1-sin^2x}=2sec^2x\)2. By definition:
\((a-b)(a+b)=a^2-b^2\)Then, you can rewrite the denominator of the second fraction in this form:
\(\frac{1}{1-sinx}+\frac{1-sinx}{(1-sinx)(1+sinx)}=2sec^2x\)3. Now you can cancel common terms:
\(\frac{1}{1-sinx}+\frac{1}{1+sinx}=2sec^2x\)4. Add the fractions using this formula for adding fractions with different denominators:
\(\frac{a}{b}+\frac{c}{d}=\frac{ad+bc}{ad}\)Then, you get:
\(\frac{(1)(1+sinx)+(1)(1-sinx)}{(1-sinx)(1+sinx)}=2sec^2x\)\(\frac{1+sinx+1-sinx}{(1-sinx)(1+sinx)}=2sec^2x\)\(\frac{2}{1-sin^2x}=2sec^2x\)5. You already know that:
\(cos^2x=1-sin^2x\)Then, you can rewrite the denominator as:
\(\frac{2}{cos^2x}=2sec^2x\)6. Remember this Reciprocal Identify:
\(\frac{1}{cosx}=secx\)Therefore, you can determine:
\(2sec^2x=2sec^2x\)Hence, the answer is:
Joyce saved $220 on an item that was 75% off what was the original price
Answers
Answer:
$880
Step-by-step explanation:
Use the equation:
\(P=(1-d)x\) with d being the discount in a decimal form, and P being the price that was bought at.
220=(1-0.75)x
simplify parenthesis terms
220=0.25x
divide both sides by 0.25
880=x
So, the original price was $880.
Hope this helps! :)
pls help me pls pls

Answers
Answer:
B
Step-by-step explanation:
the slope of parallel lines are equal
A triangular pyramid is formed from three right triangles as shown below.
Use the information given in the figure to find the length AC.
If applicable, round your answer to the nearest whole number.
The lengths on the figure are not drawn accurately.
A
41
B
85

Answers
Answer:
76 units
Step-by-step explanation:
You want the length of AC in the given triangular pyramid.
Pythagorean theoremThe Pythagorean theorem can be used to find the lengths of AD and CD.
AD² + 40² = 41²
AD² = 41² -40² = 81 . . . . . = 9²
and
CD² +40² = 85²
CD² = 85² -40² = 5625 . . . . . = 75²
It can also be used to find AC:
AD² + CD² = AC²
81 + 5625 = AC²
AC = √5706 = 3√634 ≈ 76
The length of side AC is about 76 units.
__
Additional comment
The Pythagorean theorem tells you the square of the hypotenuse is the sum of the squares of the legs of a right triangle.
<95141404393>

PLEASE HELP
Part A: Create a fifth-degree polynomial with three terms in standard form. How do you know it is in standard form? (5 points)
Part B: Explain the closure property as it relates to subtraction of polynomials. Give an example. (5 points)
Answers
A fifth-degree polynomial with three terms in standard form would be written as a\(x^{2}\) + b\(x^{4}\)+ c\(x^{3}\). The closure property states that a set is closed under a certain operation if performing that operation on any two elements of the set produces a result that is also an element of the set.
Part A: A fifth-degree polynomial with three terms in standard form would be written as a\(x^{2}\) + b\(x^{4}\)+ c\(x^{3}\). The coefficient of the highest degree term (x^5) must be non-zero and all the terms must be written in descending order of the degree. This is the definition of standard form for polynomials.
Part B: The closure property states that a set is closed under a certain operation if performing that operation on any two elements of the set produces a result that is also an element of the set. In this case, the set is polynomials and the operation is subtraction. An example of this property can be seen by subtracting two polynomials, such as 4\(x^{2}\) + 3x - 5 and \(x^{2}\) + 2. The result of this subtraction would be 3\(x^{2}\) + 3x - 5, which is also a polynomial and therefore an element of the set, demonstrating the closure property.
Learn more about polynomial here:
https://brainly.com/question/28936357
#SPJ1
A fifth-degree polynomial with three terms in standard form would be written as a\(x^{2}\) + b\(y^{2}\)+ c. The closure property states that a set is closed under a certain operation if performing that operation on any two elements of the set produces a result that is also an element of the set.
Part A: A fifth-degree polynomial with three terms in standard form would be written as a\(x^{2}\) + b\(y^{2}\)+ c. The coefficient of the highest degree term (\(x^{5}\)) must be non-zero and all the terms must be written in descending order of the degree. This is the definition of standard form for polynomials.
Part B: The closure property states that a set is closed under a certain operation if performing that operation on any two elements of the set produces a result that is also an element of the set. In this case, the set is polynomials and the operation is subtraction. An example of this property can be seen by subtracting two polynomials, such as 4\(x^{2}\) + 3x - 5 and + 2. The result of this subtraction would be 3\(x^{2}\) + 3x - 5, which is also a polynomial and therefore an element of the set, demonstrating the closure property.
Learn more about polynomial here:
https://brainly.com/question/28936357
#SPJ1
if the expression 4x + 2(x - 4) is equivalent to x +2 then what is the value of x?
Answers
Answer:
x=2
Step-by-step explanation:
4x + 2(x - 4) is equivalent to x +2
first, distribute. 4x+2x-8
next, combine like terms. 6x-8
6x-8 = x+2
we need to group all the variable terms on one side, and all the constant terms on the other side of the equation. (6x-8)+(8-x)=(x+2)+(8-x)
get rid of the parenthesis. 6x-8+8-x=x+2+8-x
combine like terms. 6x-x-8+8=x-x+2+8
solve. 5x=10
divide both by 5. 5x/5=10/5
x=2
If one card is drawn from a deck. find the probability of getting these results. Enter your answers as fractions or as decimals rounded to 3 decimal places.
Part 1
An ace and a heart
P(acc and heart)-
Part 2
An 8 or a diamond
P (8 or diamond)-
Part 3
A red 7
P(red 7)-
Answers
Answer: Part 1: 1/52 Part 2: 15/26 Part 3: 1/26
Step-by-step explanation:
A ace of hearts is only 1 card
a 8 or a diamond a 8 is a 4/52 chance and a diamond is a 26 / 52 chance
26 + 4 = 30
30/52 simplifies to 15/26
A red 7 has a 4/52 chance for a 7 and half of that for a red card so 2/52 then 1/26
5 11/12 + 3 9/12? Step by step
Answers
Answer:
Simplified: 9 2/3
Step-by-step explanation:
Convert the mixed numbers into improper fractions
To do this, multiply the denominator by the whole number and add the numerator: (denominator × whole number + numerator)/denoninator
First fraction:
5 11/12
(12 × 5 + 11)/12
(60 + 11)/12
71/12
Next fraction:
3 9/12
(12 × 3 + 9)/12
(36 + 9)/12
45/12
Substitute these fractions in the original problem now:
71/12 + 45/12
Add the numerators only. The denominators stay the same.
(71 + 45)/12
116/12
Now that the calculations are done, convert this back into a whole number
How many times does 12 fit into 116 equally? If you answered 9 times, then you are correct. This 9 will sit as the whole number in the fraction
12 × 9 = 108
This doesn't equal 116 perfectly so we must find the left over
116 - 108 = 8 left over (which is used as the numerator)
9 8/12
If your class or problem asks that you simplify your answer, then look at both the numerator and denominator to determine any common factors within them
8 and 12 both have common factors of 2 and 4
Since 4 is the bigger number, divide both 8 and 12 by 4 to simplify your answer
9 2/3
please refer to the image

Answers
Brief The Question Please, Thank You
b. 40 gallons
Identify the image of XYZ for a composition of a 150 rotation and a 30 rotation both about point y
Answers
Answer:
hlw its jess your ans is here ↑ ↓
:- 150 rotation and the other 30 rotation about the same point is equivalent to 150+30 = 180
rotation about that point

what is the value of the function when X equals four? on a coordinate plane.
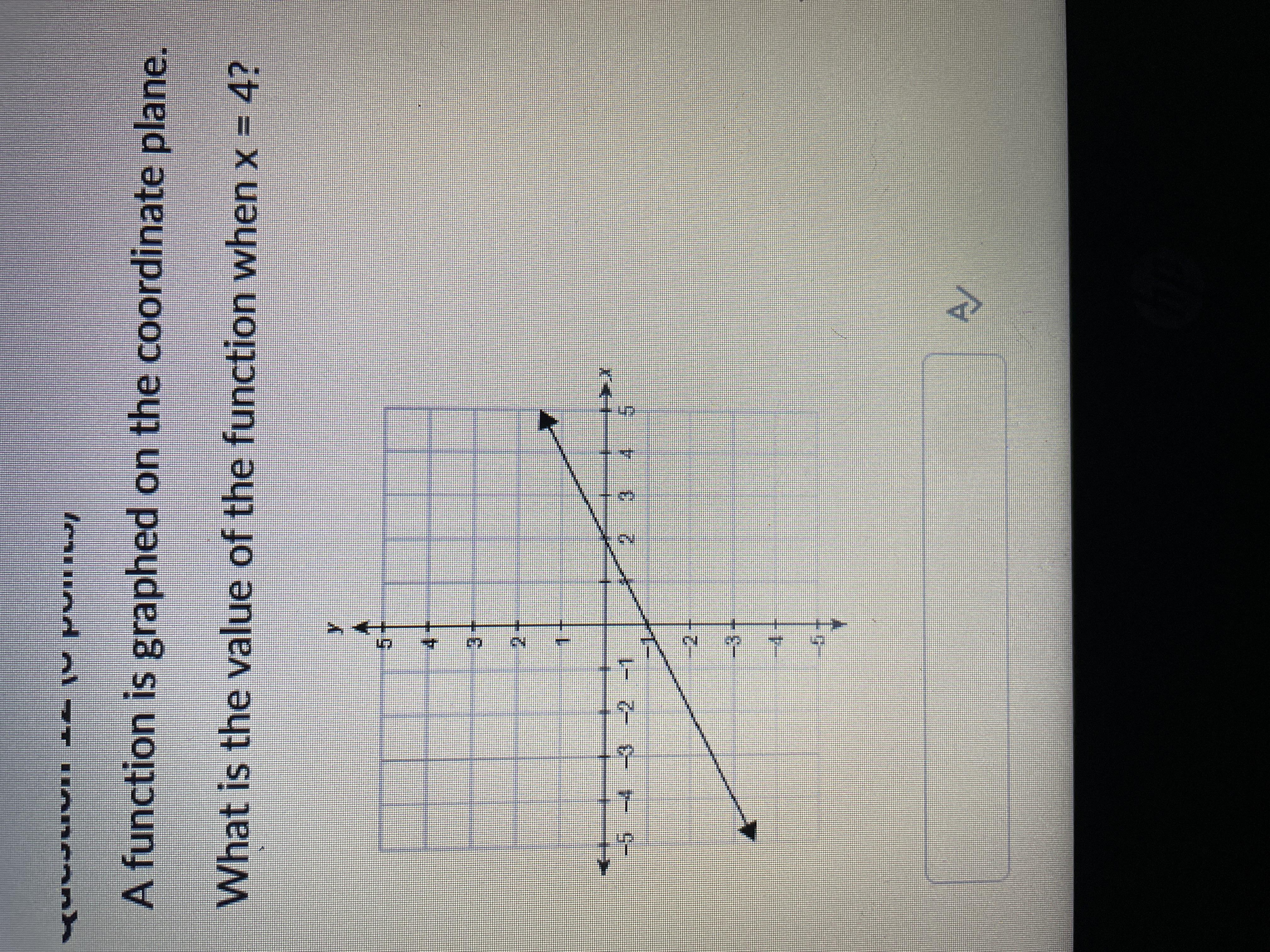
Answers
Solution :
From the given graph the x-intercept is 2 and y-intercept is -1 .
So, equation of line in intercept form is :
\(\dfrac{x}{2} + \dfrac{y}{-1} = 1\\\\x - 2y = 2\)
\(y = \dfrac{x-2}{2}\)
Now, putting x = 4 in above equation, we get :
\(y = \dfrac{4-2}{2}\\\\y = 1\)
Therefore, the value of function at x =4 is 1 .
Right angle ABC is taken by dilation with center P and scale factor 1/2 to angle A’B’C’. What is the measure of angle A’B’C’
Answers
The measure of angle A'B'C' will be 90 degree.
What is Translation?
A transformation that occurs when a figure is moved from one location to another location without changing its size or shape is called translation.
Given that;
Right angle ABC is taken by dilation with center P and scale factor 1/2 to angle A’B’C’.
Now,
Since, We know that;
When a figure is dilated by a scale factor then the shape of the figure is not change.
Thus, The measure of ABC is same as the measure of A'B'C'.
Here, The measure of angle ABC = 90 degree
Hence, The measure of angle A'B'C' = 90 degree.
Therefore, The measure of angle A'B'C' will be 90 degree.
Learn more about the dilation visit:
https://brainly.com/question/10855880
#SPJ1
Help asap please!!!
The temperature in Smallville is 30°F one morning. It rises 10 over 5 hours. The equation y = 2x+ 30 was given to represent this situation.
Which of the following tables demonstrates the relationship between the time and temperature in Smallville?
A x 0 1 2 . y 0 2 4
B x 0 1 2 . y 30 32 34
C x 30 35 40 . y 0 1 2
D x 30 32 34 . y 0 1 2

Answers
Considering the given linear function, it is found that table B represents the situation.
What is a linear function?A linear function is modeled by:
\(y = mx + b\)
In which:
m is the slope, which is the rate of change, that is, by how much y changes when x changes by 1.b is the y-intercept, which is the value of y when x = 0.In this problem, the function is given by:
y = 2x + 30.
Hence, the y-intercept is of b = 30, that is, when x = 0, y = 30, and the slope of m = 2 means that when x increases by 1, y increases by 2, hence table B is the answer for this question.
More can be learned about linear functions at https://brainly.com/question/24808124
Which inequality is true when the value of x is -5?
Answers
The true inequality from the list of options is (c) -x - 6 > -3.5
How to determine the true inequalityTo determine which inequality is true when x is -5, we need to substitute -5 for x in each inequality and simplify.
a) -x – 6 < -3.5
Substituting x = -5, we get:
-(-5) - 6 < -3.5
5 - 6 < -3.5
-1 < -3.5
This is false, so option a) is not true.
b) -x – 6 > 3.5
Substituting x = -5, we get:
-(-5) - 6 > 3.5
5 - 6 > 3.5
-1 > 3.5
This is false, so option b) is not true.
c) -x - 6 > -3.5
Substituting x = -5, we get:
-(-5) - 6 > -3.5
5 - 6 > -3.5
-1 > -3.5
This is true, so option c) is true.
Hence, the correct option is (c) -x - 6 > -3.5
Read more about inequality at
https://brainly.com/question/25275758
#SPJ1
Complete question
Which inequality is true when the value of x is -5?
a) -x – 6 < -3.5
b) -x – 6 > 3.5
c) -x - 6 > -3.5
d) x - 6 > -3.5
10 pounds of egg whites. You have 6 ounces to make one serving. How many servings can you make?
Answers
The answer is you can make 26 servings
Convert 506 minutes to hours and minutes.
Answers
Answer:
8 hours and 26 minutes
Step-by-step explanation:
To convert 506 minutes to hours and minutes, we can use the fact that there are 60 minutes in one hour.
First, we can divide 506 by 60 to find the number of hours:
506 ÷ 60 = 8 with a remainder of 26
This means that 506 minutes is equal to 8 hours and 26 minutes.
Therefore, the conversion of 506 minutes to hours and minutes is:
8 hours and 26 minutes
On a coordinate plane, a parabola opens down. It has an x-intercept at (negative 5, 0), a vertex at (negative 1, 16), a y-intercept at (0, 15), and an x-intercept at (3, 0).
The function f(x) = –x2 − 2x + 15 is shown on the graph. What are the domain and range of the function?
The domain is all real numbers. The range is {y|y < 16}.
The domain is all real numbers. The range is {y|y ≤ 16}.
The domain is {x|–5 < x < 3}. The range is {y|y < 16}.
The domain is {x|–5 ≤ x ≤ 3}. The range is {y|y ≤ 16}
Answers
The domain is all real numbers.
The range is {y|y ≤ 16}.
Option B is the correct answer.
We have,
The given parabola opens downwards and has a vertex at (-1,16).
So,
The parabola has a maximum value at y = 16.
Also, the y-intercept of the parabola is (0,15), which is below the vertex, and the parabola intersects the x-axis at (-5,0) and (3,0).
We can use the factored form of the equation of a parabola to find its equation in this case.
The factored form is:
y = a(x - h)² + k
where (h,k) is the vertex and "a" determines whether the parabola opens upwards or downwards.
Since the parabola opens downwards, "a" is negative.
Also, we know that the vertex is (-1,16), so we have:
y = a(x + 1)² + 16
To find "a", we can use one of the x-intercepts, say (-5,0).
Substituting these values into the equation gives:
0 = a(-5 + 1)² + 16
0 = 16a
a = 0
This indicates that the parabola is actually a line passing through (0,15) and (3,0). Therefore, the equation of the parabola is:
y = -x² - 2x + 15
The domain of this function is all real numbers because there are no restrictions on the values of x that can be plugged into the equation.
However, the range of the function is limited by the maximum value of y, which is 16.
Since the parabola opens downwards, all y values less than or equal to 16 are attainable.
Therefore, the domain is all real numbers, and the range is {y | y ≤ 16}.
Therefore,
The domain is all real numbers.
The range is {y|y ≤ 16}.
Learn more about functions here:
https://brainly.com/question/28533782
#SPJ1
In ΔHIJ, j = 69 cm, � m∠H=55° and � m∠I=30°. Find the length of i, to the nearest centimeter.
Answers
The length of side i in the triangle is approximately 35 cm.
What is the length of side i?To solve for the length of side i in triangle ΔHIJ, we can use the law of sines, which states:
sin A / a = sin B / b = sin C / c
wher;
A, B, and C are the angles opposite sides a, b, and c, respectively.First, we can use the fact that the sum of the angles in a triangle is 180° to find the measure of angle J:
m∠H + m∠I + m∠J = 180°
55° + 30° + m∠J = 180°
m∠J = 95°
Now we can use the law of sines:
sin I / i = sin J / j
where;
sin I is the sine of angle I, and sin J is the sine of angle J.Substituting the known values, we get:
sin 30° / i = sin 95° / 69
Solving for i, we get:
i = sin 30° x 69 / sin 95°
i = 35 cm
Learn more about length of triangle here: https://brainly.com/question/2217700
#SPJ1
PLEASE find SA Find the surface area to the nearest tenth. (Only round on the very last step). SA =
Answers
Answer:
885.2m²
Step-by-step explanation:
a is the side length of the square at the base
h is the height of the pyramid
Given
a = 18m
Get the height using the pythagoras theorem
h² =18²-9²
h² = 324 - 81
h² = 243
h = √243
h = 15.5884m
Area of the triangle = 1/2 * 18 * 15.5884
Area of the triangle = 9 * 15.5884
Area of the triangle = 140.2956m²
Area of the 4 triangles = 4(140.2956)
Area of the 4 triangles = 561.1824m²
Base area = 18 * 18
Base area = 324m²
Total surface area = Base area + Area of the 4 triangles
Total surface area = 324 + 561.1824
Total surface area = 885.1824
Total surface area = 885.2m² (to the nearest tenth)

A room has a square floor and its height is 5m. If its volume is 720 metre cube, find the dimensions of its floor.
Answers
Answer:
12mStep-by-step explanation:
A room has a square floor and its height is 5m. If its volume is 720 metre cube, find the dimensions of its floor.
we find the area of the square base
720 : 5 = 144m²
we find the side of the square room
√144 = 12m
Answer:
length = 12m
width = 12m
Step-by-step explanation:
720/5 = 144
144 = area of this floor
\(\sqrt{144}\) = 12m
this floor is square
then length and width are same
so length = 12m
width = 12m
Simplify.
Write without exponents.

Answers
Answer:
First one: 27
Second one: 1/8
Step-by-step explanation:
Hope this helps! :)
Represent 2x + 3y = 6 by a graph. Write the coordinates of the point where it meets: (a) x-axis
Answers
The point where the line 2x + 3y = 6 intersects the x-axis is (3, 0). This means that when x is equal to 3, y is equal to 0.
To graph the equation 2x + 3y = 6, we can rewrite it in the slope-intercept form, y = mx + b, where m represents the slope and b represents the y-intercept.
Starting with the given equation, we isolate y to one side:
3y = -2x + 6
y = (-2/3)x + 2
Now, we have the equation in slope-intercept form, y = (-2/3)x + 2. The slope is -2/3, and the y-intercept is (0, 2).
To find the point where the graph intersects the x-axis, we need to determine the coordinates where y is equal to zero. This occurs when the line crosses the x-axis.
Setting y = 0 in the equation, we have:
0 = (-2/3)x + 2
(-2/3)x = -2
x = (-2)(-3/2) = 3
Therefore, the point where the line 2x + 3y = 6 intersects the x-axis is (3, 0). This means that when x is equal to 3, y is equal to 0, indicating the point of intersection with the x-axis on the graph.
To more on Graph:
https://brainly.com/question/19040584
#SPJ8
19 pounds = how many
ounces
Answers
Answer:
304oz
Step-by-step explanation: