The neck of a gifaffe is 4 1/2 feet in length. Its neck is 30 perocent of it height whats the higet of the giffae

Step-by-step explanation:

To convert 6 3/4 meters to centimeters, we need to multiply the value by 100.

First, convert the mixed number to an improper fraction:

6 3/4 = (6 * 4 + 3)/4 = 27/4

Now, multiply the fraction by 100:

(27/4) * 100 = 675/2 = 337.5

Therefore, 6 3/4 meters is equal to 337.5 centimeters.

Answer:

The larger angle is 143

Step-by-step explanation:

x + y = 180 (This is the meaning of supplementary. Two angles make up 180 degrees)

4x - 5 = y

x + (4x - 5) = 180            Remove the brackets.

x + 4x - 5 = 180

5x - 5 =  180                  Add 5 to both sides

5x - 5 +5 = 180 + 5       Combine

5x = 185                        Divide by 5

5x/5=185/5

x = 37

y = 4*37 - 5

y = 148 - 5

y = 143

Answer:

what do we have to solve? surface area? volume?

Answer:

9 x 9 = 81

9 x 12.8 x 1/2 (basically just dividing by 2) = 57.6(area of 1 triangle),                     57.6 x 4(there are 4 sides of triangles) = 230.4

Let's add all the given areas:

81 + 230.4 = 311.4 ft^2

The probability that the proportion of flops in a sample of 506 released films would differ from the population proportion by greater than 3% is approximately 0.0192 or 1.92% (rounded to four decimal places).

Given:

Population proportion of flops: p = 9% = 0.09

Sample size: n = 506

First, let's calculate the standard deviation (σ) of the sampling distribution, which represents the standard error of the proportion:

σ = √[(p * (1 - p)) / n]

  = √[(0.09 * (1 - 0.09)) / 506]

  ≈ √[(0.0819) / 506]

  ≈ √[0.000161955]

  ≈ 0.012736

Next, we need to find the z-score associated with a difference of 3% from the population proportion:

z = (0.03 - 0) / σ

  = 0.03 / 0.012736

  ≈ 2.3542

Using a standard normal distribution table or calculator, we can find the probability associated with the z-score of 2.3542. However, since we are interested in the probability that the proportion differs from the population proportion by greater than 3%, we need to consider both tails of the distribution.

The probability of a difference greater than 3% is equal to the probability of a z-score less than -2.3542 or greater than 2.3542.

P(z < -2.3542) + P(z > 2.3542)

Looking up the values in the standard normal distribution table or using a calculator, we find:

P(z < -2.3542) ≈ 0.0096

P(z > 2.3542) ≈ 0.0096

Adding these probabilities:

0.0096 + 0.0096 ≈ 0.0192

To learn more about probability click here:

https://brainly.com/question/30034780

#SPJ11

Answer:

It would take 4 minutes.

Step-by-step explanation:

Step 1: divide 63 by 3 to get how many hotdogs the champion can eat in a minute which equals 21

Step 2: Divide 84 by 21 to get 4 minutes

The velocity of the top of the ladder is 20.62 feet per second.

We can use the Pythagorean theorem to relate the distance between the wall and the base of the ladder to the height of the ladder. Let h be the height of the ladder, then we have:

h² + 7² = 25²

h² = 576

h = 24 feet

We can then use the chain rule to find the velocity of the top of the ladder. Let v be the velocity of the base of the ladder, then we have:

h² + (dx/dt)² = 25²

2h (dh/dt) + 2(dx/dt)(d²x/dt²) = 0

Simplifying and plugging in h = 24 and dx/dt = -2, we get:

(24)(dh/dt) - 2(d²x/dt²) = 0

Solving for (d²x/dt²), we get:

(d²x/dt²) = (12)(dh/dt)

We can find (dh/dt) using the Pythagorean theorem and the fact that the ladder is sliding down the wall at a rate of 2 feet per second:

h² + (dx/dt)² = 25²

2h(dh/dt) + 2(dx/dt)(d²x/dt²) = 0

Substituting h = 24, dx/dt = -2, and solving for (dh/dt), we get:

(dh/dt) = -15/8

Finally, we can find (d²x/dt²) by plugging in (dh/dt) and solving:

(d²x/dt²) = (12)(dh/dt) = (12)(-15/8) = -45/2

Therefore, the velocity of the top of the ladder is 20.62 feet per second.

Learn more about Pythagorean theorem here: brainly.com/question/28361847

#SPJ4

Answer:

I think 5

Step-by-step explanation:

5u^2

just count the no. of squares

if they allocate sides or an area for the small square then:

sides

(side^2 • 5)

eg.  the side is equal to 2cm, then

(2^2 • 5) = 4(5)

              = 20 cm^2

allocated area for small square

square area • 5

eg. one square has an area of 4 cm^2

4 • 5 = 20 cm^2

Answer:

Answers are below

Step-by-step explanation:

The domain of the function is all real numbers.

The range of the function is also all real numbers.

I graphed the function on the graph below.

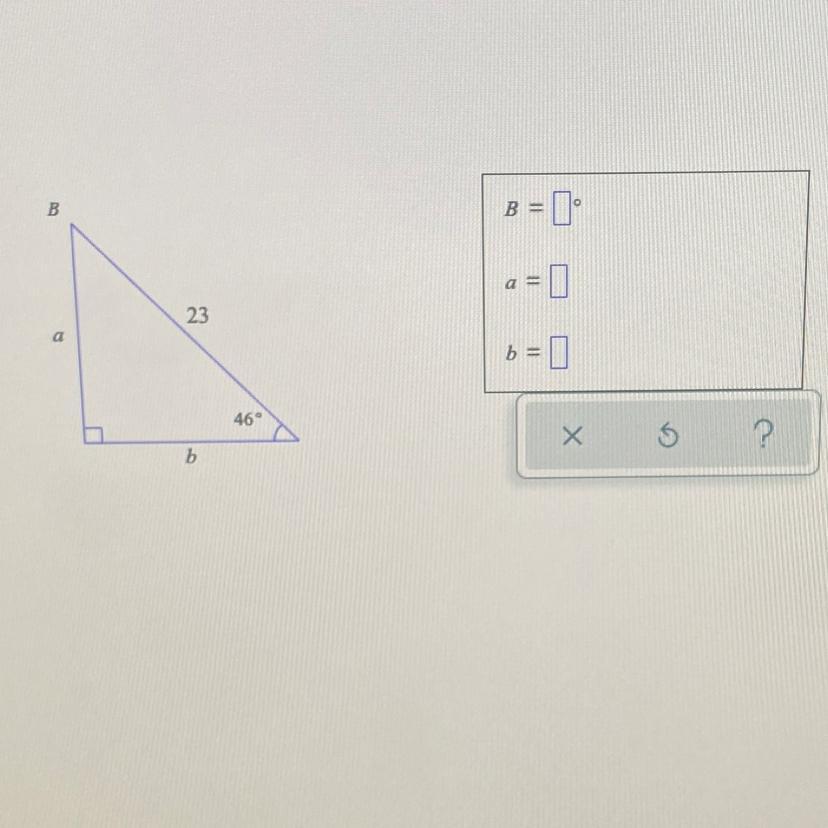
Answer:

Step-by-step explanation: we will first work out a

so 23 is the hypotenuse

a is the opposite

so what we need to do is 46sin(23) and that will give you 17.97 cm

next we will work out b

b is the adjacent

23 is the hypotenuse

so in this case we must do 46cos(23) and that will give you 42.34

last, we will work out B

a is 17.97 cm and its the adjacent

23 is the hypotenuse

so we do sin-1(17.97/23)= 51.38

It is true that the  Partial least squares structural equation modeling (PLS-SEM) is a type of statistical analysis that allows for the examination of relationships between latent variables.

In PLS-SEM, the outer model refers to the measurement model, which assesses the relationships between the observed variables and the latent constructs, while the inner model refers to the structural model, which examines the relationships between the latent variables themselves. Unlike traditional SEM, PLS-SEM measures both the outer and inner models simultaneously, which means that the results of the analysis are obtained in one step. This makes PLS-SEM a more efficient and user-friendly method for exploring complex relationships between variables.

Partial Least Squares Structural Equation Modeling (PLS-SEM) is a two-step approach for analyzing data. In the first step, the outer (measurement) model is assessed, which focuses on the relationships between the observed variables (indicators) and their respective latent variables. In the second step, the inner (structural) model is analyzed, examining the relationships between the latent variables themselves. This two-step process ensures the validity and reliability of the measurement model before testing the structural relationships. Therefore, PLS-SEM results are not studied in one step but rather involve a sequential examination of both outer and inner models.

Visit here to learn more about variables  : https://brainly.com/question/2466865
#SPJ11

The LLN states that if an experiment with a random outcome is repeated a large number of times, the empirical probability that is observed is likely to be close to the theoretical probability.

The Law of Large Numbers (LLN) is a statistical theorem which states that if an experiment with a random outcome is repeated a large number of times, the empirical probability that is observed is likely to be close to the theoretical probability. This means that the empirical probability will approximate the theoretical probability as the number of trials increases. Therefore, the statement that is true about the LLN is that if an experiment with a random outcome is repeated a large number of times, the empirical probability that is observed is likely to be close to the theoretical probability.

Learn more about probability here

https://brainly.com/question/11234923

#SPJ4

Answer:

look it up online

Step-by-step explanation:

look it up online

Answer:

Hello didi

Step-by-step explanation:

I am changing my account

so can u tell me how I sellect as friend on this app

so didi my new id is of nature lover

Answer: D. between 4.79 and 4.8

Step-by-step explanation:

The square root of 23 (√23) is 4.79583152331. We can obviously eliminate A and B. C and D is left. C is wrong because if we approximate the square root of 23, which is 4.79583152331, we get 4.79. 4.79 > 4.78, so it can't be C. Hope this helps :)

i'm on the same test-

Answer:

Step-by-step explanation:

Yes

You almost have an isosceles triangle. The lengths of the longest line segments differ by by 0.3. So the base of the triangle is 2.8 and the long segments are connected to each other and each of them at end of 2.8

The exponential model that calculates the concentration of bacteria after x weeks can be represented by the equation B(x) = 2.1 million * (3^x), the concentration after 1.6 weeks would be approximately 14.87 million bacteria per milliliter.

This equation assumes that the concentration triples each week, starting from the initial concentration of 2.1 million bacteria per milliliter.

To estimate the concentration after 1.6 weeks, we can substitute x = 1.6 into the exponential model. B(1.6) = 2.1 million * (3^1.6) ≈ 14.87 million bacteria per milliliter. Therefore, after 1.6 weeks, the estimated concentration of bacteria in the river would be approximately 14.87 million bacteria per milliliter.

The exponential model B(x) = 2.1 million * (3^x) represents the concentration of bacteria after x weeks, where the concentration triples each week. By substituting x = 1.6 into the equation, we estimate that the concentration after 1.6 weeks would be approximately 14.87 million bacteria per milliliter.

Learn more about equation here:

https://brainly.com/question/29538993

#SPJ11

The probability that at most 22 heads occur when a fair coin is tossed 25 times is 0.9313 (approximately).

When a fair coin is tossed, there are two possible outcomes: heads or tails. Since it is a fair coin, the probability of getting heads is equal to the probability of getting tails, which is 0.5. Therefore, the probability of getting a certain number of heads when the coin is tossed a certain number of times can be calculated using the binomial distribution formula.

P(X ≤ 22) = Σ P(X = i) for i = 0 to 22, where X is the random variable representing the number of heads obtained and P(X = i) is the probability of getting i heads in 25 tosses of a fair coin.

Using a binomial distribution calculator or a probability table, we can find that P(X = i) = (25 choose i) × 0.5^25 for i = 0 to 25. We can then add up the probabilities for i = 0 to 22 to get the probability that at most 22 heads occur:

P(X ≤ 22) = P(X = 0) + P(X = 1) + P(X = 2) + ... + P(X = 22)P(X ≤ 22) = Σ P(X = i) for i = 0 to 22P(X ≤ 22) = Σ (25 choose i) × 0.5^25 for i = 0 to 22Using a calculator or software, we can find that Σ (25 choose i) × 0.5^25 for i = 0 to 22 is approximately 0.9313.

Therefore, the probability that at most 22 heads occur when a fair coin is tossed 25 times is 0.9313 (approximately).

learn more about probability here

https://brainly.com/question/13604758

#SPJ11

Answer:

6

Step-by-step explanation:

1-3=-2

-2+8=6

At a significance level of 0.05, the one-way ANOVA analysis provides evidence to reject the null hypothesis and conclude that there is a difference among machines in the mean weight of the product.

To test the claim that there is a difference among machines in the mean weight of the product, we can perform a one-way ANOVA analysis. Let's go through the steps to conduct the analysis.

Step 1: State the null and alternative hypotheses:

Null hypothesis (H₀): There is no difference among machines in the mean weight of the product.

Alternative hypothesis (H₁): There is a difference among machines in the mean weight of the product.

Step 2: Set the significance level:

The significance level (α) is given as 0.05.

Step 3: Calculate the sample means and overall mean:

Calculate the sample means for each machine and the overall mean across all machines.

Machine 1: Mean = (35 + 20 + 40 + 30 + 25) / 5 = 30

Machine 2: Mean = (25 + 23 + 20 + 24 + 18) / 5 = 22

Machine 3: Mean = (40 + 45 + 26 + 34 + 45) / 5 = 38

Machine 4: Mean = (20 + 34 + 20 + 32) / 4 = 26

Overall mean = (30 + 22 + 38 + 26) / 4 = 29

Step 4: Calculate the sum of squares:

Calculate the sum of squares within groups (SSW) and the sum of squares between groups (SSB).

SSW = Σ(xi - Xi)², where xi represents each observation and Xi represents the mean of each machine.

SSB = Σ(ni × (Xi - X)²), where ni represents the number of observations for each machine and X is the overall mean.

SSW = (35-30)² + (20-30)² + (40-30)² + (30-30)² + (25-30)² + (25-22)² + (23-22)² + (20-22)² + (24-22)² + (18-22)² + (40-38)² + (45-38)² + (26-38)² + (34-38)² + (45-38)² + (20-26)² + (34-26)² + (20-26)² + (32-26)² = 314

SSB = (5 × (30-29)²) + (5 × (22-29)²) + (5 × (38-29)²) + (4 × (26-29)²) = 198

Step 5: Calculate the degrees of freedom:

Degrees of freedom within groups (dfW) = Total number of observations - Number of groups = 20 - 4 = 16

Degrees of freedom between groups (dfB) = Number of groups - 1 = 4 - 1 = 3

Degrees of freedom total (dft) = Total number of observations - 1 = 20 - 1 = 19

Step 6: Calculate the mean squares:

Mean square within groups (MSW) = SSW / dfW

Mean square between groups (MSB) = SSB / dfB

MSW = 314 / 16 = 19.625

MSB = 198 / 3 = 66

Step 7: Calculate the F statistic:

F statistic = MSB / MSW

F statistic = 66 / 19.625 ≈ 3.36

Step 8: Determine the critical value and compare with the F statistic:

Using the F-distribution table or a statistical software, find the critical value corresponding to α = 0.05, dfB = 3, and dfW = 16. Let's assume the critical value is 3.24.

We reject the null hypothesis since the F statistic (3.36) is greater than the critical value (3.24).

Step 9: Conclusion:

At the 0.05 significance level, we have sufficient evidence to conclude that there is a difference among machines in the mean weight of the product.

ANOVA Table -

Source of Variation    |  Sum of Squares  |  Degrees of Freedom  |  Mean Square  |  F-Value

-----------------------------------------------------------------------------------------

Between Groups    |       198        |          3           |      66          |   3.36

Within Groups        |       314         |          16          |      19.625   |

Total                        |       512        |          19          |                     |

-----------------------------------------------------------------------------------------

Learn more about the ANOVA analysis at

https://brainly.com/question/31747747

#SPJ4

The question is -

There are four different machines to produce one of the products. you claim that the weights of the products in the different machine is different. the machines need justification. You randomly select five products from each machine from trials on an automated driving machine. at the 0.05 significance level, is there a difference among machines in the mean weight of the product? test this claim showing all calculation steps clearly in the ms. A world file (25p) and draw an ANOVA table for the solution (10p).

Machine 1             Machine 2           Machine 3              Machine 4

     35                         25                        40                           20

     20                         23                        45                           34

     40                         20                        26                           34  

     30                         24                        34                           20

     25                         18                         45                           32

The equation representing the situation is:

5 + 2.25m = 38.75

Let's break down the equation step by step.

Jazmin's ride home consists of two components: an initial cost of $5 to pick her up and a variable cost based on the distance traveled, which is $2.25 for every mile to her final destination.

To represent the total cost of the ride, we add the initial cost to the variable cost. The variable cost is calculated by multiplying the rate of $2.25 per mile by the number of miles traveled, represented by the variable 'm'.

Therefore, the equation becomes:

Total Cost = Initial Cost + Variable Cost

38.75 = 5 + 2.25m

This equation states that the total cost of Jazmin's ride home, which is $38.75, is equal to the initial cost of $5 plus the variable cost of $2.25 multiplied by the number of miles traveled, denoted by 'm'.

By solving this equation, we can find the value of 'm', which represents the number of miles to Jazmin's home.

Learn more about linear equation here:

https://brainly.com/question/2030026

#SPJ11

Answer:

x+8*2=57.4

x=41.4

Step-by-step explanation:

Peter is thinking about a number. So, let's represent that number by x. So, he adds 8 to that number. x+8 so far. Then he doubles the result. x+8*2  to get an answer of 57.4. x+8*2=57.4. Ok, now in order to solve for x we would take 8*2 and get 16. 57.4-16=41.4. Now we know that x is 41.4. Substitute x into the equation. 41.4+8*2=57.4. 57.4=57.4. Have a great day! :)))))

Answer:

1/8 divided by -3/16 will be -1/6 

Solution

When dividing fractions, we can invert the second fraction (the divisor) and multiply it by the first fraction (the dividend).

So, to find the result of 1/8 divided by -3/16, we can invert -3/16 to get -16/3 and then multiply it by 1/8:

(1/8) / (-3/16) = (1/8) x (-16/3) 

We can simplify this expression by cancelling out a factor of 4 from both the numerator and the denominator of 1/8:

(1/8) x (-16/3) = (-1/2) x (1/3) = -1/6 

Therefore, 1/8 divided by -3/16 is equal to -1/6. 

What is a divisor?

A divisor is a number that divides another number exactly without leaving any remainder. It is also called the number by which another number is divided. 

What is a dividend?

A dividend is a number that is divided by another number (the divisor) in a division operation. It is the number that is divided into equal parts or groups. 

To know more about divisor visit:

brainly.com/question/26086130 

#SPJ1

The result of fraction will be (-2/3).

What is the fraction?

A fraction is a type of number which has two parts, numerator and denominator. The number on the upper part is called the numerator, and the number on the bottom part is called the denominator. For example 3/8, 9/7, 1/2 etc.

Given fractions are 1/8 and -3/16.

1/8 is divided by -3/16

(1/8)÷(-3/16)

= (1/8)×(16/(-3))

= (1×16)/(8×(-3))

=16/(-24)

= -2/3

Hence, The dividing the fractions we get -2/3.

To know more about  fraction

https://brainly.in/question/54113297  from the link.

#SPJ1

Answer:

Step-by-step explanation:

V=l*w*h

V=4* 2 1/2 * 3 1/2=4*5/2*7/2=35

Answer:

V=35

Step-by-step explanation:

V=Bh

v=volume

b=base(length & width)

h=height

V=(4)(2 1/2)(3 1/2)

V=(10)(3 1/2)

V=35

The 4 properties of the rhombus are:

A quadrilateral in Euclidean geometry is a rhombus. It's a parallelogram with all sides equal and diagonals intersecting at 90 degrees. In addition, opposing sides are parallel, and opposing angles are equal. This is a fundamental property of the rhombus. A rhombus is shaped like a diamond. As a result, it's also known as a diamond.

To know more about Rhombus visit the link

https://brainly.com/question/27870968

#SPJ4

Answer

The lines are parallel

Explanation:

Given the following equations

x + 4y = 8

3x + 12y = 2

Step 1: convert the equations into a slope - intercept equation

y = mx + b

where m = slope and b = intercept

4y = -x + 8

Divide through by 4

y = -1/4x + 2

Therefore, m = - 1/4 in the above equation

For equation 2

3x + 12y = 2

12y = -3x + 2

Divide through by 12

y = -3x/12 + 2/12

y = -1/4x + 1/6

Therefore, m2 = -1/4

Since the two equations has the same slope, therefore, the lines are parallel

Answer:

6 square feet

Step-by-step explanation:

The area of a triangle can be calculated using the formula:

Area = 1/2 × base × height

In this case, the base of the triangle-shaped window is 3 feet and the height is 4 feet. So we can substitute these values into the formula and get:

Area = 1/2 × 3 feet × 4 feet

Area = 6 square feet

Therefore, the area of the triangle-shaped window is 6 square feet.

The computer virus initially infects one file, then for every minute the number files gets tripled. So the first term will be 1, the second will be 3, the third will be 9 and so on. The sequence is:

This is a geometric sequence, where each term is related to its previous by the product of a constant number. For these cases we can represent the sequence as shown below:

To find how many files will be infected after 15 minutes we need to make n=15 and solve for a, we have: