Two objects are in motion towards each other. The first has a mass of 10 kg and a velocity of 7 m/s. The second object has a mass of 10 kg and a velocity of 14 m/s. What would be the total momentum between the objects during the collision?
Answers
The total momentum between the objects during the collision is 210 kgm/s
What is momentum?Momentum is the product of the mass of an object and its velocity.
What would be the total momentum between the objects during the collision?Since two objects are in motion towards each other. The first has a mass of 10 kg and a velocity of 7 m/s. The second object has a mass of 10 kg and a velocity of 14 m/s, from the law of conservation of momentum, the total momentum during the collision is the sum of their momenta.
So, the total momentum P = p + p' where
p = momentum of first object = mv where m = mass of first object and v = velocity of first object and p' = momentum of second object where m' = mass of second object and v' = velocity of second objectSo, P = mv + m'v'
Given that
m = 10 kg, v = 7 m/s, m' = 10 kg and v' = 14 m/sSubstituting the values of the variables into the equation for total momentum, we have
P = mv + m'v'
P = 10 kg × 7 m/s + 10 kg × 14 m/s
P = 70 kgm/s + 140 kgm/s
P = 210 kgm/s
So, the total momemtum is 210 kgm/s
Learn more about total momentum here:
https://brainly.com/question/27853632
#SPJ1
Related Questions
Use the figure at the right to answer questions 9-12.
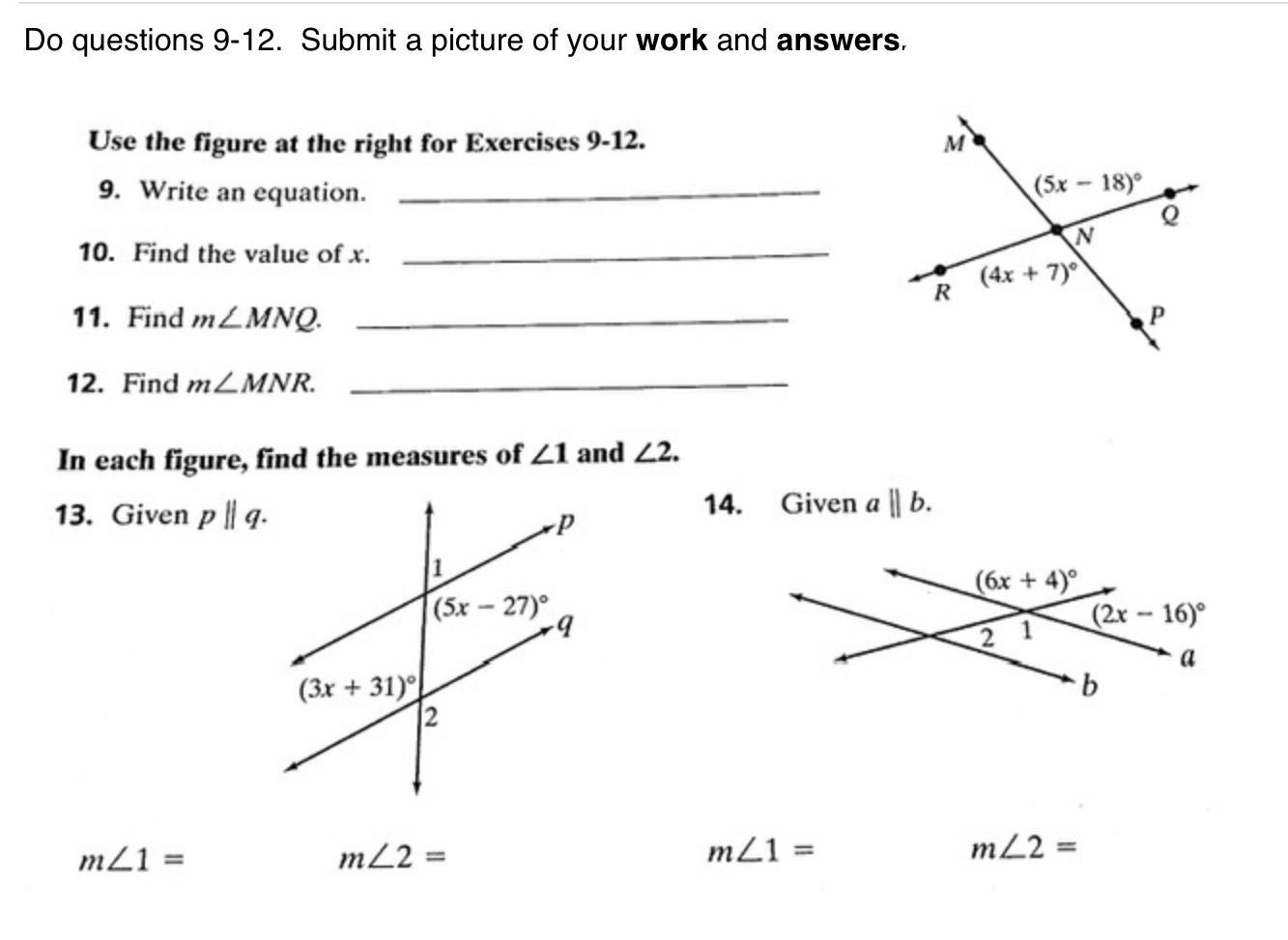
Answers
Answer:
9.) 5x - 18 = 4x + 7 10.) x = 25 11.) m∠MNQ = 107° 12.) m∠MNR = 73°
Step-by-step explanation:
9. & 10.)
∠MNQ and ∠RNP are vertical angles meaning they are equivalent to each other. Knowing that, we can set their values equal to each other and solve for x.
5x - 18 = 4x + 7 (Given)
x - 18 = 7 (Subtract 4x on both sides)
x = 25 (Add 18 on both sides)
Therefore, we know the equation is 5x - 18 = 4x + 7 and we know that x = 25.
Now we can solve for the angles.
11.)
5(25) - 18
125 - 18 = 107
Therefore, m∠MNQ = 107°.
12.)
∠MNR and ∠MNQ are supplementary angles meaning their angles will add up to 180°
∠MNR + 107 = 180
∠MNR = 73
Therefore, m∠MNR = 73°.
X-5=17??????????????????
Answers
Answer:
x=21
Step-by-step explanation:
add 5 to both sides. it gives u 21
HELP HELP HELP
HELP HELP HELP
HELP HELP HELP
(DIVISION) NO SYNTHETIC DIVISION NOR FACTORING
STEP BY STEP

Answers
Answer:
\(2x - 3 + \frac{1}{x-1}\)
Step-by-step explanation:
Attachment :)
We use long division

Find the volume of the rectangular prism.

Answers
To find the volume, you need to multiply all the values together.
1/3 x 5/6 x 2/3 = 5/27
find the area of the parallelogram determined by the points p(7, -5, 5), q(-7, 2, -2),r(10, 1, 3) and s(-4, 8, -4).
Answers
Area = 0.5 * |PQ x PR| = 0.5 * sqrt(12475) ≈ 55.93 square units.
To find the area of the parallelogram determined by these points, we need to find the cross product of the vectors formed by two adjacent sides of the parallelogram. Let's choose vectors PQ and PS:
Vector PQ = (-7 - 7, 2 - (-5), -2 - 5) = (-14, 7, -7)
Vector PS = (-4 - 7, 8 - (-5), -4 - 5) = (-11, 13, -9)
The cross product of these two vectors is:
(-7)(-9) - (-7)(13), (-2)(-9) - (-14)(-9), (-2)(13) - (-14)(-11)
= (-14, 126, -30)
The magnitude of this vector gives us the area of the parallelogram:
|(-14, 126, -30)| = sqrt(14^2 + 126^2 + (-30)^2) = sqrt(17308) ≈ 131.6
Therefore, the area of the parallelogram determined by the given points is approximately 131.6 square units.
To find the area of the parallelogram determined by the points P(7, -5, 5), Q(-7, 2, -2), R(10, 1, 3), and S(-4, 8, -4), we can use the cross product of the vectors PQ and PR.
First, let's find the vectors PQ and PR:
PQ = Q - P = (-7-7, 2-(-5), -2-5) = (-14, 7, -7)
PR = R - P = (10-7, 1-(-5), 3-5) = (3, 6, -2)
Next, find the cross product of PQ and PR:
PQ x PR = (7*(-7) - (-7)*6, (-14)*(-2) - 3*(-7), (-14)*6 - 7*3) = (-49+42, 28+21, -84-21) = (-7, 49, -105)
Now, calculate the magnitude of the cross product:
|PQ x PR| = sqrt((-7)^2 + 49^2 + (-105)^2) = sqrt(49 + 2401 + 11025) = sqrt(12475)
The area of the parallelogram is half the magnitude of the cross product:
Area = 0.5 * |PQ x PR| = 0.5 * sqrt(12475) ≈ 55.93 square units.
Learn more about parallelogram here: brainly.com/question/29147156
#SPJ11
Mila graphs the point A (5, 0) on a coordinate plane.
Which of the following statements are true?
Select all that apply.
Point A is 5 units to the right of the origin and up 1 unit______________
B. The x-coordinate of point A is 5.
C. The y-coordinate of point A is 5.
D. Point A is on the y-axis.
E. Point A is on the x-axis.
Answers
Answer:
answers B and E are true.
Step-by-step explanation:
(5,0) means x=5, y=0
the point of origin is (0,0) with x=0, y=0
therefore, A is wrong. yes, the point is 5 units to the right of the origin, but 0 (no) units up. and not 1.
B is therefore true.
C is therefore wrong.
D is wrong, because the point is to the right of y-axis (the vertical axis) and not on it.
E is true, because we go only to the right of the origin, but neither up or down. so, we stay on the x- axis (horizontal axis).
A notebook costs $2.39 at Walmart. If the tax rate is 7%, what is the total cost at the register?
Answers
$x is 7%
2.39 x 7 = 16,73
16,73/100 = 0,17
the total cost is $2,56
384 of the 403 people on a plane are passengers.
The rest of the people on the plane are crew.
What fraction of the people on the plane are crew?
Answers
19/403 of the people on the plane are crew
How to determine the crew fraction?The given parameters are:
Population = 403
Passengers = 384
The number of crew is calculated using:
Crew = 403 - 384
Evaluate
Crew = 19
The fraction of crew is then calculated using:
Fraction = Crew/Population
This gives
Fraction = 19/403
Hence, 19/403 of the people on the plane are crew
Read more about fractions at:
https://brainly.com/question/11562149
#SPJ1
Please help me with this question!!!!!

Answers
h = 11.9 cm
cos = adjacent/ hypotenuse
therefore:
cos(24) = h/ 13
rearrange:
h = 13cos(24)
put into calculator:
h = 11.8760...
rounded to one decimal point:
h = 11.9cm
Find the range of allowable values based on an expected mass of 250 grams for which values are allowed to vary by 5%.
Answers
250-12.5 < x < 250+12.5
237.5 < x < 262.5
Consider the matrix A = [1 1 0; 1 1 0; 0 0 1]
Find the singular value decomposition of A; verify that = Σ
Answers
The singular value decomposition of a matrix A is given by A = UΣV^T, where U is an orthogonal matrix, The singular value decomposition (SVD) of the matrix A = [1 1 0; 1 1 0; 0 0 1] can be found, and it can be verified that the matrix Σ is equal to the singular values of A.
The singular value decomposition of a matrix A is given by A = UΣV^T, where U is an orthogonal matrix, Σ is a diagonal matrix containing the singular values of A, and V^T denotes the transpose of the orthogonal matrix V.
To find the SVD of matrix A, we first calculate A^TA, which yields [2 2 0; 2 2 0; 0 0 1]. The eigenvalues of A^TA are λ1 = 3 and λ2 = 0, with corresponding eigenvectors u1 = [1 1 0]^T and u2 = [0 0 1]^T, respectively.
Normalizing the eigenvectors, we obtain U = [1/√2 0; 1/√2 0; 0 1].
Next, we calculate AA^T, which gives [2 2; 2 2]. The eigenvalue of AA^T is λ = 4, with the corresponding eigenvector v = [1 1]^T.
Normalizing the eigenvector, we have V = [1/√2 1/√2].
The diagonal matrix Σ is constructed using the square root of the eigenvalues, resulting in Σ = [√3 0 0; 0 0 0].
Verifying that A = UΣV^T, we multiply U, Σ, and V^T and obtain the original matrix A, confirming the validity of the SVD.
Therefore, it is verified that Σ is equal to the singular values of matrix A
Learn more about diagonal matrix here:
https://brainly.com/question/31053015
#SPJ11
bakit po ala kapong jowa
Answers
Answer:
tanong mopo sa pagong!
シ︎シ︎シ︎
Answer:
enewan nya na q :(((
Step-by-step explanation:
First step tingin salamin
sabay miss u balik kana
ahuhuhuhuh
Find the area of the figure below
77.25 cm^2 154.5 cm^2 87.55 cm^2 175.1 cm^2

Answers
The area of the given triangle is 87.55 square centimeter.
From the given triangle, base=17 cm and height=10.3 cm.
The basic formula for the area of a triangle is equal to half the product of its base and height, i.e., A = 1/2 × b × h.
Here, area of a triangle = 1/2 ×17×10.3
= 87.55 square centimeter
Therefore, area of the given triangle is 87.55 square centimeter.
To learn more about the area of a triangle visit:
brainly.com/question/27701864.
#SPJ1
PLZ HELP IM STUCK!!!!!!!!!!


Answers
Answer:
A. Because
Step-by-step explanation:
\( \frac{3 - 2}{ - 1 - 2} = \frac{1}{ - 3} \)
Kim is reading a book with 380 pages. She read 20 pages each day until she reached Part 2 of the book. Part 2 of the book is 160 pages long. She uses the equation 380−20=160 380 - 20 d = 160 to represent the number of days, d, it took her to read Part 1. How many days did it take Kim to read Part 1 of the book?
Answers
Answer:
11 days
Step-by-step explanation:
380-160=220
20 times 11 =220
answer 11 days
Nancy's morning routine involves getting dressed, eating breakfast, making her bed, and driving to work. Nancy spends ⅓ of the total time in the morning getting dressed, 10 minutes eating breakfast, 5 minutes making her bed, and the remaining time driving to work. If Nancy spends 35 ½ minutes getting dressed, eating breakfast, and making her bed, how long is her drive to work?
Answers
Answer: 26 minutes
Step-by-step explanation:
Assume that the total time spent in the morning which includes getting dressed, eating breakfast, making her bed, and driving to work is x.
Time getting dressed = ¹/₃x
Eating breakfast = 10 mins
Making bed = 5 mins
¹/₃x + 10 + 5 = 35 ½
¹/₃x + 15 = 35 ½
¹/₃x = 35 ½ - 15
¹/₃x = 20 ½
x = 20 ½ / ¹/₃
= 61.5 minutes
Total morning routine is is 61.5 mins
Time taken to drive to work is;
= 61.5 - 35 ½
= 26 minutes
Ryan needs 3 and 1/4 cups of flour for the cookie recipe she is baking. She has already added 2 and 1/2 cups of flour. How much more flour does she need to add? Please help-

Answers
The quantity of more flour that she needs to add would be = 3/4
What is a fraction?A fraction is defined as the representation of the part of a whole data in a form of numerator and denominator.
The quantity of flour Ryan needs for baking = 3¼
The quantity of flour she has already added = 2½
The remaining amount that she needs to add;
= 3¼-2½
= 3/4
Learn more about fraction here:
https://brainly.com/question/28699958
#SPJ1
The statements below can be used to prove that the triangles are similar. On a coordinate plane, right triangles A B C and X Y Z are shown. Y Z is 3 units long and B C is 6 units long. StartFraction A B Over X Y EndFraction = StartFraction 4 Over 2 EndFraction ? StartFraction A C Over X Z EndFraction = StartFraction StartRoot 52 EndRoot Over StartRoot 13 EndRoot EndFraction △ABC ~ △XYZ by the SSS similarity theorem. Which mathematical statement is missing?
Answers
Answer: c. BC/YZ=6/3
Step-by-step explanation:
trust
Answer: The answer is C. BC/YZ = 6/3
Step-by-step explanation:
Got it right on edge 2023
y=7x-3. y=-7x+3
What type of solution is this?
Answers
NEED HELP NOW One solution to a quadratic equation is x=-5/3 What is true about any remaining solutions? There are no complex solutions. There are no other real solutions. There are two or more complex solutions. There is exactly one complex solution. There is exactly one more real solution. There are at least two more real solutions.
Answers
Given:
One solution to a quadratic equation is \(x=-\dfrac{5}{3}\).
To find:
The true statement about remaining solutions.
Solution:
We know that, number of solution to a quadratic equation is 2.
According to the complex conjugate root theorem, if a complex number x+iy is a root of an equation then its conjugate x-iy is also a root of that equation.
It means, the complex roots are in the pair of two.
Using complex conjugate root theorem, a quadratic equation has either 0 complex roots or 2 complex roots.
One solution to a quadratic equation is \(x=-\dfrac{5}{3}\), which is real.
There are no complex solutions and there is exactly one more real solution.
Therefore, the correct options are A and E.
8 is the quotient of a number g and 3.
Answers
So whats the question?
Answer:
g ÷3 =8
Step-by-step explanation:
You randomly select one card from a 52-card deck. find the probability of selecting a red six or a black king.
Answers
The probability of randomly selecting a red six or a black king from a 52-card deck is 1/13.
To find the probability of selecting a red six or a black king from a 52-card deck, we need to determine the number of favorable outcomes (red six or black king) and divide it by the total number of possible outcomes (52 cards).
There are 2 red sixes (hearts and diamonds) and 2 black kings (spades and clubs) in a deck.
Since we want to select either a red six or a black king, we can add these numbers together to get a total of 4 favorable outcomes.
Since there are 52 cards in a deck, the total number of possible outcomes is 52.
Now, we can calculate the probability by dividing the number of favorable outcomes by the total number of possible outcomes: Probability = Number of favorable outcomes / Total number of possible outcomes Probability = 4 / 52 Probability = 1 / 13
Therefore, the probability of randomly selecting a red six or a black king from a 52-card deck is 1/13.
For more questions on probability
https://brainly.com/question/251701
#SPJ8
a tissue box is shaped like a right rectangular prism. it has a base area of 16 square inches and a height of 513 inches.what is the volume of the tissue box?
Answers
Therefore, the volume of the tissue box is 8208 cubic inches.
To calculate the volume of the tissue box, we can use the formula:
Volume = Base Area * Height
Given that the base area is 16 square inches and the height is 513 inches, we can substitute these values into the formula:
Volume = 16 * 513
Volume = 8208 cubic inches
To know more about volume,
https://brainly.com/question/15081568
#SPJ11
(a) If you choose a single nucleotide at random from each of the two regions, what is the probability that they are the same nucleotide?
(b)You random sample a three-nucleotide sequence from each of the 2 regions. What is the chance that the triple chosen from the first region will be identical to the triple chosen from the second region? (assume that nucleotides occur independently within each region)
Answers
(a) The probability that a randomly chosen nucleotide from the first region is the same as a randomly chosen nucleotide from the second region is 1/4.
(B) The chance that the triple chosen from the first region will be identical to the triple chosen from the second region is 1/4096.
Nucleotide Probabilities: Single and Triple(a) If we assume that there are four possible nucleotides (A, C, G, T) and that each nucleotide is equally likely to occur in each region, then the probability that a randomly chosen nucleotide from the first region is the same as a randomly chosen nucleotide from the second region is 1/4, since there is a 1 in 4 chance that the nucleotide chosen from the first region will be the same as the nucleotide chosen from the second region.
(b) If we again assume that there are four possible nucleotides (A, C, G, T) and that each nucleotide is equally likely to occur in each region, then the probability of choosing a particular three-nucleotide sequence is (1/4)³ = 1/64.
The probability of choosing the same three-nucleotide sequence from both regions is the product of the probabilities of choosing that sequence from each region, or (1/64) x (1/64) = 1/4096. Therefore, the chance that the triple chosen from the first region will be identical to the triple chosen from the second region is 1/4096.
Learn more about Nucleotide Probabilities: Single and Triple here:
https://brainly.com/question/25161031
#SPJ4
Find the missing number
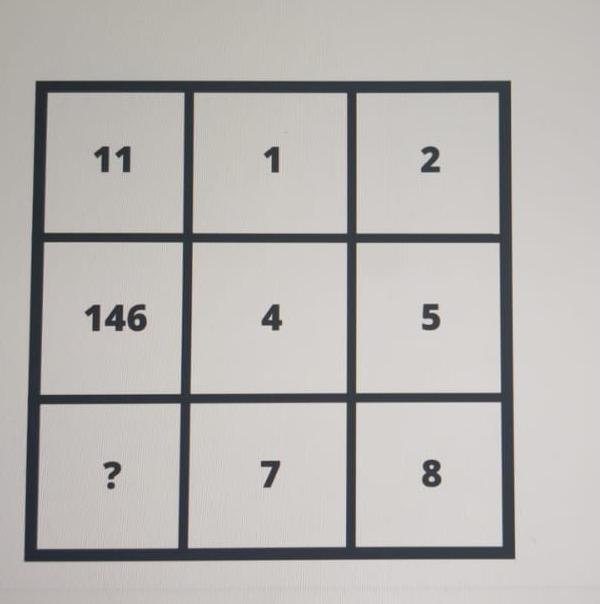
Answers
Pls help!! I can’t figure out how to do thisss

Answers
Answer: 30 and 26
Step-by-step explanation:
Select the correct answer from each drop-down menu.
Gabriel is designing equally sized horse stalls that are each in the shape of a rectangular prism. Each stall must be 9 feet high and have a volume of 1,080 cubic feet. The length of each stall should be 2 feet longer than its width.
The volume of a rectangular prism is found using the formula V = l · w · h, where l is the length, w is the width, and h is the height.
Complete the equation that represents the volume of a stall in terms of its width of x feet.
x2 +
x =
Is it possible for the width of a stall to be 10 feet?
, a2 + b2 = c2, for b, assuming a, b, and c are positive?
Answers
The equation representing the volume of a stall in terms of its width is x^2 + x. It is not possible for the width of a stall to be 10 feet and have a volume of 1,080 cubic feet.
To represent the volume of a stall in terms of its width of x feet, we can use the given information that the length of each stall is 2 feet longer than its width. Let's denote the width as x feet.
Given that the length is 2 feet longer than the width, the length would be (x + 2) feet. The height is fixed at 9 feet.
The volume of the stall can be calculated using the formula V = l · w · h, where V is the volume, l is the length, w is the width, and h is the height.
Plugging in the values, we have:
V = (x + 2) · x · 9
Simplifying this expression, we get:
V = 9x^2 + 18x
Now, we have the equation that represents the volume of a stall in terms of its width.
The equation is: x^2 + x = 1080
To check if it is possible for the width of a stall to be 10 feet, we substitute x = 10 into the equation:
10^2 + 10 = 1080
100 + 10 = 1080
110 = 1080
Since 110 is not equal to 1080, it is not possible for the width of a stall to be 10 feet in order to achieve a volume of 1080 cubic feet.
Note: The complete question is:
Gabriel is designing equally sized horse stalls that are each in the shape of a rectangular prism. Each stall must be 9 feet high and have a volume of 1,080 cubic feet. The length of each stall should be 2 feet longer than its width. The volume of a rectangular prism is found using the formula V = l · w · h, where l is the length, w is the width, and h is the height. Complete the equation that represents the volume of a stall in terms of its width of x feet. x2 + x = Is it possible for the width of a stall to be 10 feet?
For more questions on volume of a stall:
https://brainly.com/question/11253816
#SPJ8
help pls. Only answer if correct and will mark brainliest



Answers
Answer:
180 mm^2
Step-by-step explanation:
For the rectangle, you just multiply the sides together. 15x9 equals to 135. The triangle, is a little harder. For the width, you know that the width of the whole shape is 15mm. And one half of it is 9. So the other half must be 6mm. Now you have the width (6) and the length (15) of the triangle. You find the area of it which is 45mm squared. You add up both numbers to get 180.
in a simple baseline/offset model y = b0 b1*x with a dummy-variable (0 or 1) predictor x, the coefficient b1 may be interpreted as which of the following?
Answers
In a simple baseline/offset model y = b0 + b1*x with a dummy-variable predictor x, the coefficient b1 may be interpreted as the difference in the mean value of y between the two groups represented by the dummy variable.
In a simple baseline/offset model with a dummy-variable predictor x, the coefficient b1 represents the difference in the mean value of the response variable y between the two groups represented by the dummy variable. When the dummy variable takes the value of 0, it represents one group, and when it takes the value of 1, it represents the other group.
The coefficient b1 indicates the average change in y when moving from one group to the other, while holding all other variables constant. Therefore, it provides insights into the effect or impact of the group represented by the dummy variable on the response variable.
To learn more about dummy variable click here: brainly.com/question/30620953
#SPJ11
Sixty percent, or 12, of the students in Alex's class are going to the movies. 2/3, or 14, of the students in Mary's class are going to the movies. Which class has more students? Justify your answer using equations.
Answers
Answer:
Mary's class.
Step-by-step explanation:
One way to do this is to figure out how many students are in Alex's class and how many students are in Mary's class.
60% of Alex's class is 12 students
\(\frac{12*100}{60}\) = \(\frac{1200}{60}\)= 20 students
\(\frac{2}{3}\) or 66.66% (repeating) of Mary's class is 14 students
\(\frac{14*100}{66.66}\) = \(\frac{1400}{66.66}\) = 21 students
(^^66.66 repeating)
Mary's class has more students than Alex's class.