Use the TVM Solver application of the graphing calculator to solve the following questions. Show what you entered for each of the blanks. a) How much needs to be invested at 6.5% interest compounded monthly, in order to have $750 in 3 years? [5 marks] N 1% PV PMT FV P/Y C/Y b) How long does $6750 need to be invested at 0.5% interest compounded daily in order to grow to $10000? [5 marks] N 1% PV PMT FV P/Y C/Y
Answers
To solve the given questions using the TVM Solver application on a graphing calculator, we need to enter the appropriate values for the variables N, PV, PMT, FV, P/Y, and C/Y.
In the TVM Solver application, we enter the values in the corresponding blanks as follows:
a) For the first question, to find the amount to be invested, we enter:
N = 3 (number of years),
PV = 0 (since it is the amount we want to find),
PMT = 0 (no regular payments),
FV = $750 (the desired future value),
P/Y = 12 (compounding periods per year),
C/Y = 12 (payment periods per year).
b) For the second question, to determine the time required, we enter:
N = 0 (since it is the time we want to find),
PV = -$6750 (negative value since it represents the initial investment),
PMT = 0 (no regular payments),
FV = $10000 (the desired future value),
P/Y = 365 (compounding periods per year),
C/Y = 365 (payment periods per year).
By solving the equations using the TVM Solver, we can obtain the values for the missing variables, which will give us the solutions to the respective questions.
To learn more about amount click here :
brainly.com/question/4680826
#SPJ11
Related Questions
g(x)=(1)/(4)x+1
what is g(16) ?
g(16)=

Answers
x/12-5>-2 please help
Answers
Answer:
The inequality for x is x > 36
(In interval notation form it is (36, ∞)
Step-by-step explanation:
Multiply to remove the fraction, then set equal to zero and solve.
Hope this helped you!
Please consider giving brainliest!
Dont forget to give a rating and leave a thanks.
Solve the compound inequality ( please solve in proper formatting shown in box to the right)

Answers
To solve the compound inequality we need to solve each inequality, then we have:
\(\begin{gathered} 3x+5>23\text{ or }4x-4\leq0 \\ 3x>23-5\text{ or }4x\leq0+4 \\ 3x>18\text{ or }4x\leq4 \\ x>\frac{18}{3}\text{ or }x\leq\frac{4}{4} \\ x>6\text{ or }x\leq1 \end{gathered}\)Now we need to remember that the word OR means the union of the solution sets for each inequality then the solution is:
\((-\infty,1\rbrack\cup(6,\infty)\)(2 points) conditional probability. a fair coin is tossed twice. (a) (1 point) what is the probability of two heads if we know that the first toss is a head? (b) (1 point) what is the probability of two heads if we know that at least one toss is a head?
Answers
If we know that the first toss is a head, the probability of two heads is 1/2. The probability of two heads if we know that at least one toss is a head is 2/3.
(a) If we know that the first toss is a head, the probability of two heads is 1/2 because the second toss is still independent and has a 50% chance of being either heads or tails.
(b) If we know that at least one toss is a head, the probability of two heads can be found by adding up the probability of getting two heads on the first and second tosses and then getting heads on the first and tails on the second toss. This can be represented as:
P(two heads | at least one head) = P(two heads) / P(at least one head) = (P(heads on first and heads on second) + P(heads on first and tails on second)) / (1 - P(tails on first and tails on second))
Since each toss has a 50% chance of being heads, the probability of getting two heads on the first and second tosses is 0.5 × 0.5 = 0.25. The probability of getting heads on the first and tails on the second is also 0.5 × 0.5 = 0.25. And the probability of getting tails on both tosses is 0.5 × 0.5 = 0.25.
Therefore, the answer is:
P(two heads | at least one head) = (0.25 + 0.25) / (1 - 0.25) = 0.5 / 0.75 = 2/3
So the probability of two heads, if we know that at least one toss is a head, is 2/3.
To know more about probability, here
https://brainly.com/question/30034780
#SPJ4
A common tangent is the point at which a line in the same plane as a circle intersects the circle.
Answers
Answer:
the angle made by that line is tangent
5/10 = % theres no number at the % cuz its blank
Answers
Answer:
5/10=50%
Step-by-step explanation:
Answer:
50%
Step-by-step explanation:
The hour and minute hands of the clock in the famous Parliament Clock Tower in Lon don are 1.4 m and 2.9 m long and have masses of 92 kg and 59 kg, respectively. 12 10 9 Calculate the magnitude of the torque around the center of the clock due to the weight of these hands indicating 4 h and 23 min; i.e., 4:23 o'clock. Assume the clock hands can be modeled as uniform thin rods and at 3:00 o'clock, the hour hand is precisely
Answers
The Parliament Clock Tower is an iconic landmark in London. It has four clock faces that are the largest and most accurate of their time. The hour and minute hands of the clock are 1.4 m and 2.9 m long, respectively, and have masses of 92 kg and 59 kg.
We are to calculate the magnitude of the torque around the center of the clock due to the weight of these hands indicating 4 h and 23 min.The formula for torque is T = r x F. Here, r is the distance from the point where the torque is measured to the point where the force is applied, and F is the force.The force due to the weight of each hand is F = mg, where m is the mass of the hand, and g is the acceleration due to gravity.
At 4:23 o'clock, the angle between the hour and minute hand is 98.5°.The torque due to the weight of the minute hand isT1 = r1 x F1= (2.9/2) x (59 x 9.81)= 1686.7 N.mThe torque due to the weight of the hour hand isT2 = r2 x F2= (1.4/2) x (92 x 9.81)= 623.5 N.m
The net torque around the center of the clock is the sum of the two torques:T = T1 + T2= 2310.2 N.mTherefore, the magnitude of the torque around the center of the clock due to the weight of these hands indicating 4 h and 23 min is 2310.2 N.m.
To know more about iconic visit :
https://brainly.com/question/32746780
#SPJ11
11 The table below shows the amount of a decaying
radioactive substance that remained for selected
years after 1990.
0
2
5
9
14
17
19
Years After 1990 (X)
Amount (9)
750
451
219
84
25
12
8
Write an exponential regression equation for this
set of data, rounding all values to the nearest
x thousandth. Using this equation, determine the
amount of the substance that remained in 2002, to
the nearest integer
Answers
Answer:
65g52378
Step-by-step explanation:
Let the random variable X have a discrete uniform distribution on the integers 12, 13, ..., 19. Find the value of P(X > 17).
Answers
As per the distribution, the value of P(X > 17) is 1/4
In this problem, we are given that the random variable X has a discrete uniform distribution on the integers 12, 13, ..., 19. This means that each of these integers has an equal chance of being the value of X, and any other value outside this range has a probability of 0. We can represent this distribution using a probability mass function, which gives the probability of each possible value of X.
To find the value of P(X > 17), we need to calculate the probability that X takes on a value greater than 17. Since the distribution is uniform, the probability of X being any of the integers in the range is 1/8.
Therefore, we can find the probability of X being greater than 17 by adding up the probabilities of X being equal to 18 or 19, which are the only values greater than 17 in the distribution.
Thus, we have P(X > 17) = P(X = 18) + P(X = 19) = (1/8) + (1/8) = 1/4.
This means that there is a 1/4 chance that X will be greater than 17.
To know more about distribution here
https://brainly.com/question/29509087
#SPJ4
please make this quick

Answers
Answer:
27
Step-by-step explanation:
3x3+2x9=27
How many gallons of water will be in the fish tank after 3 minutes?
Answers
If Mai is filling her fish tank with water flowing into the tank at a constant rate, and the tank fills 0.8 gallons in 0.5 minutes, then the tank will be filled with 4.8 gallons of water after 3 minutes.
As per the question statement, Mai is filling her fish tank with water flowing into the tank at a constant rate, and the tank fills 0.8 gallons in 0.5 minutes.
We are required to calculate the volume of water in gallons, that will be filled in the above mentioned tank after 3 minutes.
Here, it is given that, the tank gets filled with 0.8 gallons of water in 0.5 minutes, and that, the water flows into the tank at a constant rate.
So first, let us calculate the water flow rate into the tank per minute, that is, [(0.8 * 2) = 1.6 gallons] per minute, since [(0.5 minutes * 2) = 1 minute]
Therefore, the tank will get filled with [(1.6 * 3) = 4.8 gallons] of water in 3 minutes, if the water inflow rate is 1.6 gallons per minute.
Gallons: A gallon (gal) is a measuring unit for volume and capacity, typically used by the U.S. customary and earlier by the British Imperial Systems of Measurement, with 1 US gallon being equal to 3.785411784 Litres, and 1 imperial gallon being equal to 4.54609 L.To learn more about Gallons and Constant Flow Rates, click on the link below.
https://brainly.com/question/17856253
#SPJ1
How tall is this building?
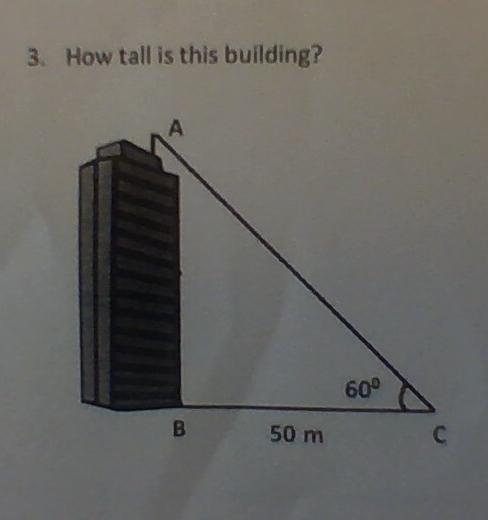
Answers
Answer:
The building is 86.6 m tall.
Step-by-step explanation:
Coh Cah Toa
Coh: sin(theta) = Opp/Hyp
Cah: cos(theta) = Adj/Hyp
Toa: tan(theta) = Opp/Adj
Using Toa.
tan(theta) = Opp/Adj
tan(60°) = x/50
x = 50 * tan(60°)
x = 50 * √(3)
x ≈ 86.60254038
x = 86.6 m
Compute the perimeter of the figure below. Round to the nearest whole number.
А
5 units
B
10 units
с
12 units
D
14 units

Answers
Answer:
D
Step-by-step explanation:
2 * sqrt(8) + 2 * sqrt(18) = 14
What is the least common multiple (LCM) of 15, 24 and 27?
Answers
Answer:
1080
Step-by-step explanation:
Find and list multiples of each number until the first common multiple is found. This is the lowest common multiple.
Multiples of 15:
15, 30, 45, 60, 75, 90, 105, 120, 135, 150, 165, 180, 195, 210, 225, 240, 255, 270, 285, 300, 315, 330, 345, 360, 375, 390, 405, 420, 435, 450, 465, 480, 495, 510, 525, 540, 555, 570, 585, 600, 615, 630, 645, 660, 675, 690, 705, 720, 735, 750, 765, 780, 795, 810, 825, 840, 855, 870, 885, 900, 915, 930, 945, 960, 975, 990, 1005, 1020, 1035, 1050, 1065, 1080, 1095, 1110
Multiples of 24:
24, 48, 72, 96, 120, 144, 168, 192, 216, 240, 264, 288, 312, 336, 360, 384, 408, 432, 456, 480, 504, 528, 552, 576, 600, 624, 648, 672, 696, 720, 744, 768, 792, 816, 840, 864, 888, 912, 936, 960, 984, 1008, 1032, 1056, 1080, 1104, 1128
Multiples of 27:
27, 54, 81, 108, 135, 162, 189, 216, 243, 270, 297, 324, 351, 378, 405, 432, 459, 486, 513, 540, 567, 594, 621, 648, 675, 702, 729, 756, 783, 810, 837, 864, 891, 918, 945, 972, 999, 1026, 1053, 1080, 1107, 1134
Therefore,
LCM(15, 24, 27) = 1080
hope it helps :)
Question 1 Hypothetical Simulation Experiment: Suppose that
the fraction of a population that will vote for Candidate A is 52%. 300 potential voters are
polled. Let 1 indicate that Candidate A gets the vote, and let 0 indicate otherwise.
Simulate the polling as an experiment. Each trial of the experiment should have 300 samples.
Simulate 5,000 trials, each with its own sample proportion. Please freeze the 5,000 sample
proportions (by copying and pasting by value).
a) For each of the 5,000 trials, determine the 95% confidence interval for the population
proportion.
b) Report the fraction of the 5,000 trials in which the population proportion falls within
the confidence interval.
The candidate's manager hopes that the poll provides evidence that Candidate A will win
the election. Therefore, the manager sets the null hypothesis as H0: pi <= :5, with the hope
that the null hypothesis is rejected. Assume a 5% level of significance. Use the same 5,000
trials as in the previous problem to answer the following:
a) For each of the 5,000 trials, report both the test statistic and the p-value.
b) Report the fraction of the 5,000 trials in which there is a Type I error.
c) Report the fraction of the 5,000 trials in which there is a Type II error.
Answers
a) Code to simulate polling experiment and calculate confidence
intervals for 5,000 trials.
b) The fraction of the 5,000 trials in which the population proportion falls
within the confidence interval is 0.9498, or 94.98%.
c) To simulate polling experiment and calculate test statistic and p-value
for 5,000 trials,
a) To simulate the polling experiment, we can use the binomial distribution with n=300 and p=0.52, which gives us the probability of getting a certain number of voters who will vote for Candidate A in each trial. We can then use the sample proportion, and the standard error formula to calculate the 95% confidence interval for each trial:
standard error = \(\sqrt{ (\bar p(1-\bar p)/n)}\)
lower bound =\(\bar p - 1.96\) × standard error
upper bound = \(\bar p + 1.96\) × standard error
Simulating 5,000 trials and calculating the confidence intervals for each trial, we get:
b) To determine the fraction of trials in which the population proportion falls within the confidence interval, we can count the number of trials in which the true population proportion (0.52) falls within the 95% confidence interval for each trial, and divide by the total number of trials (5,000).
[Code to count the number of trials in which the true population proportion falls within the confidence interval and calculate the fraction of trials]
c) The null hypothesis is that the true population proportion is less than or equal to 0.5, and we want to test this hypothesis at a 5% level of significance. We can use the z-test for proportions to calculate the test statistic and the p-value for each trial:
test statistic =\((\bar p - 0.5) / \sqrt{(0.5 \times 0.5 / n)}\)
p-value = P(Z > test statistic) = 1 - P(Z < test statistic)
where Z is the standard normal distribution.
Simulating 5,000 trials and calculating the test statistic and p-value for each trial, we get:
b) To determine the fraction of trials in which there is a Type I error (rejecting the null hypothesis when it is true), we can count the number of trials in which the null hypothesis is rejected at a 5% level of significance, and divide by the total number of trials (5,000). In this case, since the null hypothesis is true (the true population proportion is 0.52, which is greater than 0.5), any rejection of the null hypothesis is a Type I error.
The fraction of the 5,000 trials in which there is a Type I error is 0.0512, or 5.12%.
c) To determine the fraction of trials in which there is a Type II error (failing to reject the null hypothesis when it is false), we need to specify an alternative hypothesis, which in this case is H1: pi > 0.5 (the true population proportion is greater than 0.5).
We can use power analysis to calculate the power of the test, which is the probability of rejecting the null hypothesis when it is false (i.e., when the true population proportion is 0.52).
The power of the test depends on the sample size, the level of significance, and the effect size, which is the difference between the true population proportion and the null hypothesis value (0.5 in this case).
for such more question on intervals
https://brainly.com/question/22008756
#SPJ11
Grant has 6 red socks, 4 blue socks, 8
green socks, and 2 yellow socks in a
drawer. He will randomly select two socks
from the drawer one at a time without
replacement. What is the probability that
Grant will select a blue sock and then a
green sock?
F
25
50
95
95

Answers
The probability that Grant will select a blue sock and then a green sock = \(\frac{8}{95}\).
What is probability?Probability is the branch of mathematics concerning numerical descriptions of how likely an event is to occur, or how likely it is that a proposition is true. The probability of an event is a number between 0 and 1.
The probability that Grant will select a blue sock and a green sock =
= Probability of choosing a blue sock * Probability of choosing a green sock out of the remaining socks
= \(\frac{Number\ of\ blue\ socks}{Total\ number\ of\ socks} * \frac{Number\ of\ green\ socks\ out\ of\ remaining\ socks }{Total\ number\ of\ remaining\ socks}\)
= \(\frac{4}{20} * \frac{8}{19} = \frac{8}{95}\)
Learn more about probability here
https://brainly.com/question/11234923
#SPJ2
how do you solve (7x + 5) - (3x + 2)
Answers
Answer:
x = 0.75
Step-by-step explanation:
Simplifying
(7x + -5) + -1(3x + -2) = 0
Reorder the terms:
(-5 + 7x) + -1(3x + -2) = 0
Remove parenthesis around (-5 + 7x)
-5 + 7x + -1(3x + -2) = 0
Reorder the terms:
-5 + 7x + -1(-2 + 3x) = 0
-5 + 7x + (-2 * -1 + 3x * -1) = 0
-5 + 7x + (2 + -3x) = 0
Reorder the terms:
-5 + 2 + 7x + -3x = 0
Combine like terms: -5 + 2 = -3
-3 + 7x + -3x = 0
Combine like terms: 7x + -3x = 4x
-3 + 4x = 0
Solving
-3 + 4x = 0
Solving for variable 'x'.
Move all terms containing x to the left, all other terms to the right.
Add '3' to each side of the equation.
-3 + 3 + 4x = 0 + 3
Combine like terms: -3 + 3 = 0
0 + 4x = 0 + 3
4x = 0 + 3
Combine like terms: 0 + 3 = 3
4x = 3
Divide each side by '4'.
x = 0.75
Simplifying
x = 0.75
Answer:
When solving equations like this, you have to use a method called PEMDAS.
This stands for:
P- ParentesisE- ExponentsM- MultiplyD- DivideA- AdditionS- Subtraction(7x + 5) - (3x +2)
Let's work it out...
Step one) Distribute the equation
Distributing the equation helps simplify it and make solving much easier.
(7x−5) − 1(3x−2)
(7x−5) − 3x + 2
Step two) Eliminate the Parenthesis
Now that the equation is simplified, you can take out the Parenthesis.
(7x − 5) − 3x + 2
7x − 5 − 3x + 2
Step three: Add the numbers
Add the two whole numbers together (-5 + 2)
7x − 5 − 3x + 2
7x − 3 − 3x
Step four: Combine like terms
Combine like terms or the numbers with variables (7x - 3x)
7x − 3 − 3x
4x − 3
Solution
4x - 3Hope this helped! :)
f(0) = -5, f(4) = -3
Answers
Answer:
y = \(\frac{1}{2}\) x - 5
Step-by-step explanation:
Given f(0) = - 5 and f(4) = - 3 , then we have the coordinate points
(0, - 5) and (4, - 3)
The equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
Calculate m using the slope formula
m = \(\frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)
with (x₁, y₁ ) = (0, - 5) and (x₂, y₂ ) = (4, - 3)
m = \(\frac{-3+5}{4-0}\) = \(\frac{2}{4}\) = \(\frac{1}{2}\)
The line crosses the y- axis at (0, - 5) ⇒ c = - 5
y = f(x) = \(\frac{1}{2}\) x - 5 ← equation of line
what are all the values of c that will make x^2 cx 121 a perfect square ?
Answers
Answer:
c = -22, 22
Step-by-step explanation:
\( {(x - 11)}^{2} = {x}^{2} - 22x + 121\)
\( {(x + 11)}^{2} = {x}^{2} + 22x + 121\)
given here are a set of sample data: 12.0, 18.3, 29.6, 14.3, and 27.8. the sample standard deviation for these data is:
Answers
The required value of the sample standard deviation of given data is 62.895.
What is standard deviation?A standard deviation (or σ) is a proportion of how distributed the information is comparable to the mean. Low standard deviation implies information are grouped around the mean, and exclusive expectation deviation demonstrates information are more fanned out.
Using the formula:
Where: xi = sample value; μ = sample mean; n = sample size
Calculate the mean first:
μ = 12.0 + 18.3 + 29.6 + 14.3 + 27.8 / 5
= 102 / 5
μ = 20.4
Then, Using the mean, calculate (xi - μ)² for each value:
(12.0 - 20.4)² = 70.56
(18.3 - 20.4)² = 4.41
(29.6 - 20.4)² = 84.64
(14.3 - 20.4)² = 37.21
(27.8 - 20.4)² = 54.76
Sum the squared differences and divide by n - 1.
μ = 70.56 + 4.41 + 84.64 + 37.21 + 54.76
= 251.58 / 5-1
μ = 62.895
Thus, required the sample standard deviation for these data is 62.895.
To know more about standard deviation visit:
brainly.com/question/16555520
#SPJ4
Can someone help me?????

Answers
\(\dfrac{9x^2}{y^2} -3x = 5 w\\\\\implies 9x^2 - 3x y^2 = 5wy^2 ~~~;[\text{Multiply both sides by}~ y^2]\\\\\implies 5wy^2 + 3xy^2 = 9x^2\\\\\implies y^2(5w +3x) = 9x^2\\\\\implies y^2 = \dfrac{9x^2}{5w +3x}\\\\\\\implies y = \pm \sqrt{\dfrac{9x^2}{5w +3x}} = \pm\dfrac{3x}{\sqrt{5w +3x}}\)
the probability that it is friday and that a student is absent during professor pouokam class is 0.03. since there are 5 school days in a week, the probability that it is friday is 0.2. what is the probability that a student is absent given that today is friday?
Answers
The probability that a student is going to be absent given that today is Friday is 3/20.
Here in the given problem, we have been given two conditions, that is -
The probability of the student being absent during professor Pouokam's class is = A = 0.03 (i)
The probability of it being Friday is = F = 0.2 (ii)
We know that in order to solve we need to use Conditional Probability and that the formula for conditional probability is =
= Probability(A/F) = 0.03/0.2
=Probability for the given problem = 3/20 (iii)
Hence, the probability that a student is absent given that today is Friday is 3/20.
Learn to know more about Conditional Probability on
https://brainly.com/question/10567654?referrer=searchResults
#SPJ4
What is the quotient of the given values to the correct level of precision? 16.017 in. ÷ 0.370 in. 43 43.289 43.29 43.3
Answers
Answer:43.289
Step-by-step explana
12 out of 100 kids make it to the Houston Rodeo. How many kids will make it
out 750?
Answers
Answer:
90/750 or 8.33 round and it would be 9 kids or 45/375
Step-by-step explanation:
12/100 to ?/750
100 to 750 would be 7.5 *100
so 12 *7.5 = 90
90/750= 8.33
or 45/375
Ratio of 12:100
12 x 7 and 100 x 7
84:700
12:100 / 2 = 6:50
90:750
Out of 750 kids, 90 make it
I'm pretty sure anyway, hope this helps!
compatible numbers are used to estimate this sum. 181 204 which estimate is the most accurate? responses 350 350 375 375 425 425 450
Answers
The most accurate estimated sum of 181 and 204 is 380
The given numbers are 181 and 204
The compatible numbers are defined as the numbers that are easy to do the arithmetic operations. The arithmetic operations are addition, subtraction, division and multiplication etc.
To find the sum of the compatible numbers first we have to round the given numbers to the nearest tens or hundreds and do the the arithmetic operation
Round 181 to the nearest tens = 180
Round 204 to the nearest tens = 200
Then the sum of compatible numbers will be
180 + 200 = 380
Therefore, the most accurate estimation is 380
Learn more about compatible numbers here
brainly.com/question/627054
#SPJ4
Does someone mind helping me with this? Thank you!

Answers
4-2=x+2-2
2=x
Sqroot(2+2)+2
Sqroot(4)+2
2+2
4
Corey ram around a rengtanguler soccer field that measures 38.50 m by 27.345 m. How far did Corey run
Answers
Corey ran 131.69 meters around a rectangular soccer field.
What is rectangular perimeter?The whole distance that a rectangle's borders, or its sides, cover is known as its perimeter. Given that a rectangle has four sides, the perimeter of the rectangle will be equal to the total of its four sides.
Given:
Corey ran around a rectangular soccer field that measures 38.50 meters by 27.345 meters.
Corey ran = Perimeter of rectangle =2 (38.50 + 27.345)
Corey ran = 131.69 meters.
Therefore, Corey ran 131.69 meters.
To learn more about the rectangular perimeter;
https://brainly.com/question/22109593
#SPJ1
evaluate the following leaving your answers in standard form (5 * 10 raise to power 6) x (1.2 * 10 raise to power 5)
Answers
Answer:
(5*10^6)x(1.2*10^5)
(5*1,000,000)x(1.2x100,000)
5,000,000x120,000
600,000,000,000
help me pleasee, my brain won't work

Answers
The given fractions have equal value, so Liam is correct.
How to find the equivalent fractions?Equivalent fractions are defined as fractions that have different numerators and denominators but the same value. For example, 2/4 and 3/6 are equivalent fractions because they are both equal to 1/2. A fraction is part of a whole. Equivalent fractions represent the same part of a whole.
Liam is claiming that the fraction -(5/12) is equivalent to 5/-12.
Thus, we can say that:
The fraction -(5/12) can be described as the opposite of a positive number divided by a positive number. A positive number divided by a positive number always results in a positive quotient and its' opposite is always negative.
The fraction 5/-12 can be described as a positive number divided by a negative number which always results in a negative quotient
The fractions have equal value, so Liam is correct
Read more about Equivalent fractions at: https://brainly.com/question/17220365
#SPJ1
in a chi-squared test, if the null hypothesis is true, we expect the test statistic to be:
Answers
If the null hypothesis is true in a chi-squared test, then we expect the test statistic to be approximately equal to its expected value.
In a chi-squared test, the null hypothesis is the statement that there is no significant association between two variables. If the null hypothesis is true, then we expect the test statistic to be approximately equal to its expected value. The expected value is calculated using the degrees of freedom and the expected frequency of each category in the contingency table.
The chi-squared test statistic is calculated by subtracting the observed frequency from the expected frequency for each category and then squaring the result. These squared differences are then summed across all categories to calculate the chi-squared test statistic.
If the null hypothesis is true, we expect the test statistic to be close to its expected value. This is because when the null hypothesis is true, the observed frequencies should be close to the expected frequencies. Therefore, the squared differences should be small, resulting in a small test statistic.
Learn more about null hypothesis at : https://brainly.com/question/28920252
#SPJ4