Which answer correctly describes the transformation from the preimage to the
image?
a translation 10 units left and 8 units down
a reflection over the x-axis
a translation 10 units right and 8 units up
a rotation of 180°
a reflection over the y-axis

Answers
Translation of 10 units left and 8 units down
================================================
Explanation:
Always start with the preimage. Let's say we focus on point A located at (3,6). Its corresponding point is A' located at (-7,-2). To go from (3,6) to (-7,-2), we move 10 units to the left and 8 units down.
The motion "10 units to the left" means we subtract 10 from the x coordinate. The "8 units down" part means we subtract 8 from the y coordinate.
Overall, the translation rule is \((x,y) \to (x-10,y-8)\)
If we applied that rule to A(3,6), then...
\((x,y) \to (x-10,y-8)\\\\(3,6) \to (3-10,6-8)\\\\(3,6) \to (-7,-2)\\\\\)
which is what the diagram shows going from A to A'
Let's do the same for point B(3,1)
\((x,y) \to (x-10,y-8)\\\\(3,1) \to (3-10,1-8)\\\\(3,1) \to (-7,-7)\\\\\)
This indicates B(3,1) moves to B ' (-7,-7) which is what the diagram shows. I'll let you confirm that C(6,6) moves to C ' (-4,-2) using that translation rule.
Related Questions
Laurie is running in a 5,000-meter race. A kilometer equals about 0.625 mile. About how
many miles long is the race?
Answers
Answer:
3.125 miles
Step-by-step explanation:
5 × 0.625 = 3.125 miles
if the multiplier is 6, then the mpc is group of answer choices A. 0.16.
B. 0.83
C. 0.71.
D 0.86.
Answers
The correct answer choice for the MPC (marginal propensity to consume) when the multiplier is 6 is not provided among the options A. 0.16, B. 0.83, C. 0.71, or D. 0.86.
The MPC is calculated as the ratio of the change in consumption to the change in income. When the multiplier is given, we can derive the MPC using the formula MPC = 1 / (1 + MPC). In this case, the multiplier is stated to be 6.
To find the corresponding MPC, we can solve the equation 1 / (1 + MPC) = 6 for MPC.
Rearranging the equation, we have 1 + MPC = 1 / 6. Subtracting 1 from both sides, we get MPC = 1 / 6 - 1 = -5 / 6.
The result MPC = -5 / 6 implies a negative MPC, which does not align with any of the given answer choices.
Additionally, all the answer choices provided (0.16, 0.83, 0.71, and 0.86) are positive values, further confirming that none of them represents the correct MPC when the multiplier is 6.
Therefore, the correct answer choice for the MPC when the multiplier is 6 is not listed among the options A. 0.16, B. 0.83, C. 0.71, or D. 0.86.
Learn more about MPC (marginal propensity to consume) here:
https://brainly.com/question/32276464
#SPJ11
Conditional Statement: If the object has a remote, then it is a television.
Part A: Write the converse to the conditional statement.
Part B: Write a biconditional statement from the conditional statement.
Part C: Validate the biconditional statement from Part B. Use details to support your answer.
Answers
For given conditional statement 'If the object has a remote, then it is a television.'
Part A: converse to the conditional statement
'If it is a television, then the object has a remote.'
Part B: a biconditional statement
'The object has a remote if and only if it is a television.'
Part C: validation of biconditional statement
The biconditional statement is true because the conditional statement and the converse are true.
In this question, we have been given a conditional statement: 'If the object has a remote, then it is a television.'
Part A:
We need to write the converse to the conditional statement.
The converse of given conditional statement would be:
'If it is a television, then the object has a remote.'
Part B:
We need to write a biconditional statement from the conditional statement.
A biconditional statement of the given conditional statement would be:
'The object has a remote if and only if it is a television.'
Part C:
We need to validate the above biconditional statement.
The biconditional statement is true because the conditional statement and the converse are true.
Therefore, for given conditional statement 'If the object has a remote, then it is a television.'
Part A: converse to the conditional statement
'If it is a television, then the object has a remote.'
Part B: a biconditional statement
'The object has a remote if and only if it is a television.'
Part C: validation of biconditional statement
The biconditional statement is true because the conditional statement and the converse are true.
Learn more about conditional statement here:
https://brainly.com/question/28804708
#SPJ1
johnny is a very picky eater, so he likes to use a lot of condiments. he has ketchup, salt, pepper, and shredded cheese at his disposal. his mother tells him he may only make two additions to his meal (i.e., he can add condiments only twice, regardless of whether or not he already used them). how many different ways can johnny improve his meal?
Answers
Johnny can improve his meal in 6 different ways by choosing two condiments from his four options. Some examples of the different combinations include ketchup and salt, ketchup and pepper, salt and pepper, and so on.
To determine the number of different ways Johnny can improve his meal using condiments, we can use the concept of combinations.
Since Johnny can only make two additions to his meal, we need to find the number of combinations of condiments he can choose from his four options: ketchup, salt, pepper, and shredded cheese.
To calculate the number of combinations, we can use the formula for combinations:
nCr = n! / (r! * (n - r)!)
Where n represents the total number of items and r represents the number of items to be chosen.
In this case, n is 4 (since Johnny has four condiment options) and r is 2 (since Johnny can only make two additions).
Plugging these values into the formula, we get:
4C2 = 4! / (2! * (4 - 2)!)
Simplifying this expression:
4C2 = 4! / (2! * 2!)
The exclamation mark (!) represents the factorial operation, which means multiplying a number by all positive integers less than itself down to 1.
Calculating the factorials:
4! = 4 * 3 * 2 * 1 = 24
2! = 2 * 1 = 2
Substituting these values back into the equation:
4C2 = 24 / (2 * 2)
Simplifying further:
4C2 = 24 / 4
Finally, dividing:
4C2 = 6
Therefore, Johnny can improve his meal in 6 different ways by choosing two condiments from his four options. Some examples of the different combinations include ketchup and salt, ketchup and pepper, salt and pepper, and so on.
To know more about combinations refer here:
https://brainly.com/question/31586670
#SPJ11
please help me
The lengths of a triangle are 50 m, 120 m, and 130 m. Which formula could be used to show that the triangle is a right triangle?
A. 502 + 1302 = 1202
B. 1202 + 502 1302
C. 1302 + 1202 = 502
D. 502 + 502 1202
Answers
Answer:
Step-by-step explanation:
1302 + 1202 = 502
Answer:
1302 + 1202 = 502
Step-by-step explanation:
Cant really explain. 1302 + 1202 = 502
Factor 35v+15
Write your answer as a product with a whole number greater than 1
Answers
Answer:
5(7v+3)
Step-by-step explanation:
Look for what both terms have in common and factor that
35v + 15
Rewrite 35 as 5*7 and 15 as 5*3
5*7v + 5*3
Both terms have in common 5 so will factor it out
5(7v+3)
Find the vector components of multiple vectors and how to verify the sum using components method?
Answers
To find ,we can break down each vector into its horizontal and vertical components. The horizontal component represents the vector's magnitude in the x-axis , and the vertical component the magnitude in the y-axis direction.
To verify the sum of vectors using the components method, we can add the horizontal components together and the vertical components together. If the resultant sum of the horizontal components equals the horizontal component of the resultant vector, and the sum of the vertical components equals the vertical component of the resultant vector, then the components method is verified.
To find the vector components, we typically use trigonometry. Given a vector with magnitude (r) and an angle (θ) measured counterclockwise from the positive x-axis, we can find the horizontal component (x-component) and the vertical component (y-component) using the following equations:
x-component = r * cos(θ)
y-component = r * sin(θ)
To verify the sum of vectors using the components method, we add the horizontal components together and the vertical components together. Let's say we have two vectors A and B. The components of vector A are (A₁, A₂), and the components of vector B are (B₁, B₂). The components of the resultant vector R would be (A₁ + B₁, A₂ + B₂).
To verify the sum, we check if the sum of the horizontal components (A₁ + B₁) equals the horizontal component of the resultant vector R, and the sum of the vertical components (A₂ + B₂) equals the vertical component of the resultant vector R. If these conditions are satisfied, the sum of vectors using the components method is verified.
In summary, vector components can be found by breaking down vectors into their horizontal and vertical components. To verify the sum of vectors using the components method, we add the horizontal and vertical components separately and check if they match the components of the resultant vector.
Learn more about summary here
brainly.com/question/32025150
#SPJ11
Find the distance between the origin and the point (-12,5).
A. 12
B. 6
C. 7
D. 13

Answers
Answer:
D. 13
Step-by-step explanation:
Answer:
I'd pick 13
Step-by-step explanation:
Let M (-12,5) be the given point and O (0,0) be the origin.
What is the distance between 3/4 and 2/5 on the number line?
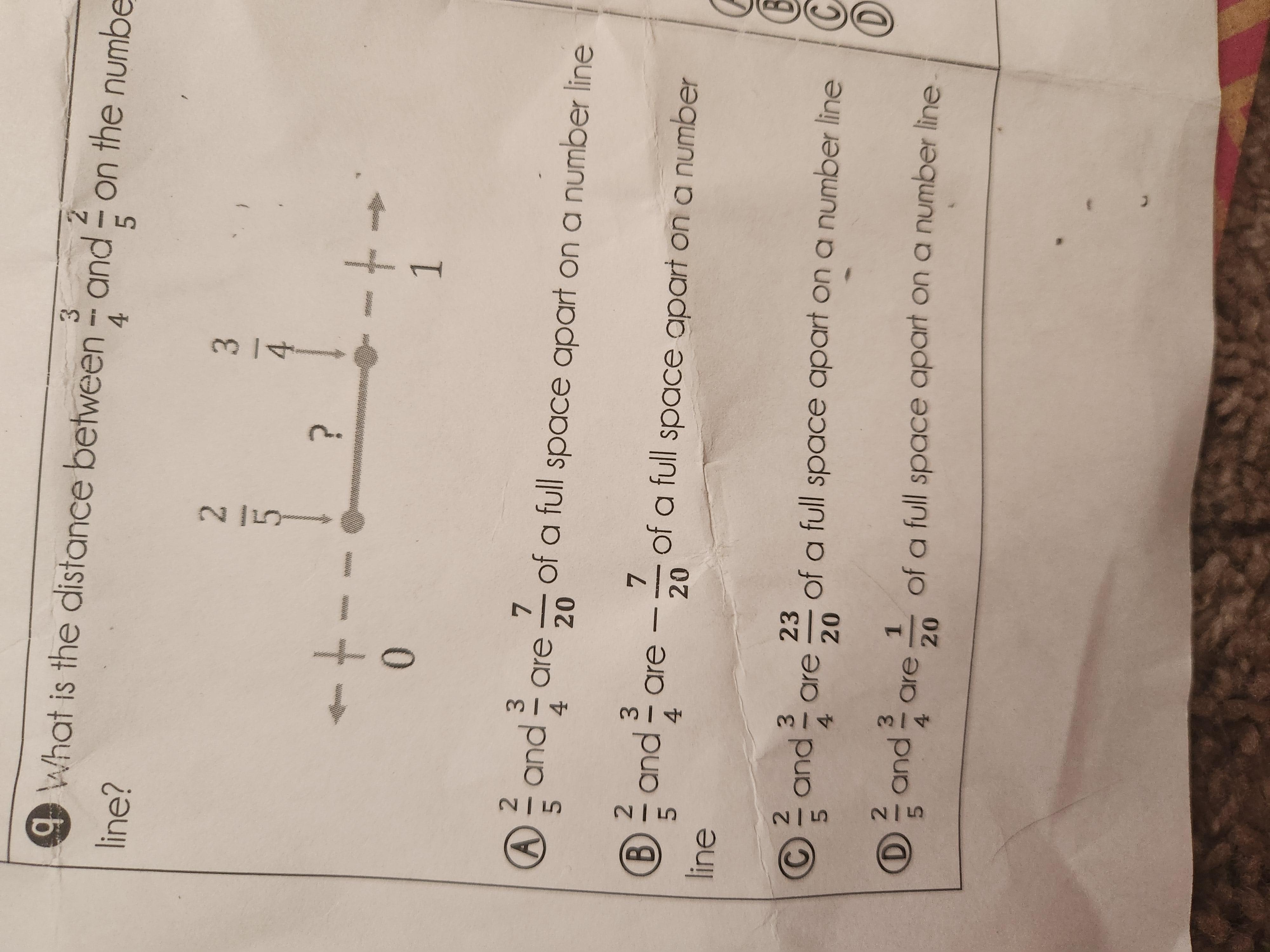
Answers
Answer:
О A) 7/20
Step-by-step explanation:
3/4 = 15/20
2/5 = 8/20
Then:
15/20 - 8/20 = (15-8) / 20
= 7 / 20
...
Select all names that apply to the number. 0
Answers
There is multiple names it has, like for example “Zero” and in British English it is “nought” which is often used as an archaic word for nothing, I hope this help’s.
Given a normal distribution with u = 100 and o= 10, complete parts (a) through (d).
a. What is the probability that X> 85? The probability that X> 85 is_____(Round to four decimal places as needed.) b. What is the probability that X<80? The probability that X < 80 is ____(Round to four decimal places as needed.) c. What is the probability that X<90 or X> 130? The probability that X<90 or X> 130 is ____ (Round to four decimal places as needed.) d. 99% of the values are between what two X-values (symmetrically distributed around the mean)? 99% of the values are greater than __ and less than _(Round to two decimal places as needed.)
Answers
To solve the given problems, we'll use the properties of the normal distribution with mean μ = 100 and standard deviation σ = 10.
a. Probability that X > 85:
To find this probability, we need to calculate the area under the normal curve to the right of 85. We can use the standard normal distribution table or a calculator to find the corresponding z-score and then use the z-table to find the probability.
First, let's calculate the z-score:
z = (X - μ) / σ
z = (85 - 100) / 10
z = -15 / 10
z = -1.5
Using the z-table or a calculator, we find that the probability of Z > -1.5 is approximately 0.9332.
Therefore, the probability that X > 85 is 0.9332 (rounded to four decimal places).
b. Probability that X < 80:
Similarly, we'll calculate the z-score for X = 80:
z = (X - μ) / σ
z = (80 - 100) / 10
z = -20 / 10
z = -2
Using the z-table or a calculator, we find that the probability of Z < -2 is approximately 0.0228.
Therefore, the probability that X < 80 is 0.0228 (rounded to four decimal places).
c. Probability that X < 90 or X > 130:
To calculate this probability, we'll find the individual probabilities of X < 90 and X > 130, and then subtract the probability of their intersection.
For X < 90:
z = (90 - 100) / 10
z = -10 / 10
z = -1
Using the z-table or a calculator, we find that the probability of Z < -1 is approximately 0.1587.
For X > 130:
z = (130 - 100) / 10
z = 30 / 10
z = 3
Using the z-table or a calculator, we find that the probability of Z > 3 is approximately 0.0013.
Since these events are mutually exclusive, we can add their probabilities:
P(X < 90 or X > 130) = P(X < 90) + P(X > 130)
P(X < 90 or X > 130) = 0.1587 + 0.0013
P(X < 90 or X > 130) = 0.1600
Therefore, the probability that X < 90 or X > 130 is 0.1600 (rounded to four decimal places).
d. 99% of the values are between what two X-values (symmetrically distributed around the mean)?
To find the two X-values, we need to find the corresponding z-scores for the cumulative probabilities of 0.005 and 0.995. These probabilities correspond to the tails beyond the 99% range.
For the left tail:
z = invNorm(0.005)
z ≈ -2.576
For the right tail:
z = invNorm(0.995)
z ≈ 2.576
Now we can find the corresponding X-values:
X1 = μ + z1 * σ
X1 = 100 + (-2.576) * 10
X1 = 100 - 25.76
X1 ≈ 74.24
X2 = μ + z2 * σ
X2 = 100 + 2.576 * 10
X2 = 100 + 25.76
X2 ≈ 125.76
Therefore, 99% of the values are greater than 74.24 and less than 125.76 (rounded to two decimal places).
To learn more about probability : brainly.com/question/31828911
#SPJ11
the lengths of human pregnancies (gestation) can be modeled by a bell-shaped distribution with mean 266 days and standard deviation 16 days. a certain pregnancy had a z-score of -2.5 (negative 2.5). how many days did this pregnancy last?
Answers
If a certain pregnancy had a z-score of -2.5 with mean 266 days and standard deviation 16 days , the pregnancy lasted 230 days.
We know that the length of human pregnancies can be modeled by a normal distribution with a mean of 266 days and a standard deviation of 16 days.
We are also given that a certain pregnancy had a z-score of -2.5. The z-score is a measure of how many standard deviations a data point is away from the mean. A negative z-score means that the data point is below the mean.
We can use the formula for converting a z-score to an actual value in a normal distribution:
z = (x - mu) / sigma
where z is the z-score, x is the actual value we want to find, mu is the mean, and sigma is the standard deviation.
Plugging in the given values, we get:
-2.5 = (x - 266) / 16
Solving for x, we get:
x = (-2.5 * 16) + 266 = 230
To learn more about z-score click on,
https://brainly.com/question/19547491
#SPJ4
rockwell hardness of pins of a certain type is known to have a mean value of 60 and standard deviation of 1.8. i) if the distribution is normal, what is the probability that the sample mean hardness for a random sample of 15 pins is at least 61? ii) without assuming population normality, what is the (approximate) probability that the sample mean hardness for a random sample of 50 pins is at least 61?
Answers
Using the normal distribution and the central limit theorem, we have that there is a:
a) 0.95 = 0.95% probability that the sample mean hardness for a random sample of 15 pins is at least 61.
b) 0% approximate probability that the sample mean hardness for a random sample of 50 pins is at least 61.
In a normal distribution with mean and standard deviation , the z-score of a measure X is given by:
Z = x-μ/ σ
It measures the number of standard deviations between the measurements and the mean.
Once the z-score is found, we look at the z-score table and find the p-value associated with that z-score, i.e. the percentile of X.
By the Central Limit Theorem, the sampling distribution of sample means of size n has standard deviation s = σ/√n
In this problem:
Mean of 50, hence μ = 6
Standard deviation of 1.2, hence σ = 1.8
Sample of 9, hence n =9, s = 1.8/√9 = 0.6
(i) Item a:
The probability is 1 subtracted by the p-value of Z when X = 51, hence: Z = x-μ/ σ
By the Central Limit Theorem:
Z = 61-60/0.6
⇒ Z = 1.6
⇒ Z = 1.6 has a p value of 0.05
Therefore,
1 - 0.05 = 0.95
There is a 0.95 = 0.95% probability that the sample mean hardness for a random sample of 15 pins is at least 61.
(ii) Item b:
Even though the distribution is not normal, the sample size is of
n = 61 > 50 , hence the Central Limit Theorem can be applied.
S = 1.8/√40
= 0.28
Based on: Z = x-μ/ σ
Z = 61 - 60/ 0.19
Z = 5.26
Z = 5.26 has a p-value of 1.
Now,
1 - 1 = 0
0% approximate probability that the sample mean hardness for a random sample of 50 pins is at least 61.
Learn more about Sample:
https://brainly.com/question/27860316
#SPJ4
g2 + 23 when g = 6??? help
Answers
If the equation of AC is y=2x-1
Calculate the coordinates of T
Answers
(1/2, 0) are the coordinates of point T
To find the coordinates of point T, we need to determine where the line y = 2x - 1 intersects with the x-axis.
The x-axis is represented by y = 0 since the y-coordinate is zero on the x-axis.
To find the x-coordinate of point T, we substitute y = 0 into the equation y = 2x - 1 and solve for x:
0 = 2x - 1
Adding 1 to both sides, we get:
1 = 2x
Dividing both sides by 2, we find:
x = 1/2
So, the x-coordinate of point T is 1/2.
To find the y-coordinate of point T, we substitute the x-coordinate (1/2) into the equation y = 2x - 1:
y = 2(1/2) - 1
Simplifying, we have:
y = 1 - 1
y = 0
Therefore, the coordinates of point T are (1/2, 0).
Point T is the point of intersection between the line y = 2x - 1 and the x-axis. It represents the x-value where the line crosses the x-axis, and since the y-coordinate is 0, it lies on the x-axis. The x-coordinate of T is 1/2, indicating that it is located halfway between the y-axis and the line y = 2x - 1.
know more about Point of intersection here:
https://brainly.com/question/30548599
#SPJ8
If 1,200 people were interviewed to create the circle graph below how many of the people interviewed said that a fish was their favorite pet?
Answers
To solve the above question
We will follow the steps below:
The total people interview is 1200
23 % of the people interviewed said that a fish was their favorite pet
So we will simply find 23 % of 1200
\(\frac{23}{100}\times\text{ 1200}\)= 27600/100
= 276
Therefore 276 people interviewed said that a fish was their favorite pet
(1 point) a poll is taken in which 302 out of 525 randomly selected voters indicated their preference for a certain candidate. (a) find a 95% confidence interval for p.
Answers
Using the standard z table, a 95% confidence interval for p is (0.5327,0.6173).
In the given question,
A poll is taken in which 302 out of 525 randomly selected voters indicated their preference for a certain candidate.
We have to find a 95% confidence interval for p.
From the question, x=302, n=525
So estimation point,
P=x/n
P=302/525
P=0.575
Now the z value of 95% confidence interval is 1.960 using the standard z table.
Margin of Error (E)=z×√{P(1−P)}/n
Now putting the value
E=1.960×√{0.575(1−0.575)}/525
E=1.960×√(0.575×0.425)/525
E=1.960×√0.244/525
E=1.960×√0.000465
E=1.960×0.0216
E=0.0423
At 95% confidence interval for p is
P−E ≤ p ≤ P+E
Now putting the value
0.575−0.0423≤ p ≤0.575+0.0423
0.5327≤ p ≤0.6173
Hence, a 95% confidence interval for p is (0.5327,0.6173).
To learn more about confidence interval link is here
brainly.com/question/24131141
#SPJ4
Which set of values makes the inequality n > -5 true?
А
{-5, -5.5,-6)
B
{-5, -4.5, -3)
Ć
(-6,0,5)
D
{-6, -7,-8}
Answers
Answer:
the answer is c :)
Step-by-step explanation:
The hotel staff is decorating the lobby by placing one row of string lights along the outer edge of the rectangular ceiling. A member of the staff maps the ceiling on a coordinate grid as shown, where each unit represents 1 meter.
Answers
The length of string used to decorate the ceiling = 40.5 meters.
According to the statement
we have given that the hotel staff is decorating the lobby by placing one row of string lights along the outer edge of the rectangular ceiling.
And the coordinates of rectangular ceiling is A(5,16), B(17,10), C(14,4), D(2,10).
And we have to find the total length for string is used to decorate the rectangular ceiling.
We know that the Total length of string = Area of the rectangular ceiling.
And area of rectangular ceiling = L*B
So, Length of rectangular ceiling = A+B
Length of rectangular ceiling = (17-5) + (16-10)
Length of rectangular ceiling = (12+6)/2
Length of rectangular ceiling = 9.
So,
Breadth of rectangular ceiling = B+C
Breadth of rectangular ceiling = (17-14)+(10-4)
Breadth of rectangular ceiling = (3+6) /2
Breadth of rectangular ceiling = 4.5
So, the length of string used = 4.5*9
the length of string used = 40.5 meters.
The length of string used to decorate the ceiling = 40.5 meters.
Learn more about Length here https://brainly.com/question/1972490
Disclaimer: This question was incomplete. Please find the full content below.
Question:
The hotel staff is decorating the lobby by placing one row of string lights along the outer edge of the rectangular celling. A member of the s⊥aff maps the celling on a coordinate grid as shown, where each unit represents . What is the approximate length of string lights the staff needs to decorate the ceiling?
A 20.25 meter
B. 35 meter
C. 40.5 meter
D. 14 meter
#SPJ4
during a 7 hour snowstorm it snows at a rate of 1 inch per hour for the first 2 hours, at a rate of 2 inches per hour four the next four hours and at rate of 1 inch per hour for the final hour. Write a piece wise function that gives the depth of the snow during the snowstorm.
Answers
Answer:
9 inches of snow fell in the 7-hour snowstorm.
Step-by-step explanation:
To find your answer write an equation to find your answer.
1 inch per hour for two hours 1(2)
2 inches per hour for four hours 4(2)
and 1 inch in the last hour
so add those up
1(2)+4(2)+1
2+8+1
9
Can someone help me on this Proofs problems? It's just number one and two.

Answers
See Explanation
Step-by-step explanation:
1. By interior angle sum Postulate of a triangle.
\( m\angle a+ m\angle y +m\angle z= 180\degree.... (1)\\\\
m\angle a+ m\angle w +m\angle x= 180\degree.... (2)\\\\\)
From equations (1) & (2), we find:
\( m\angle a+ m\angle y +m\angle z= m\angle a+ m\angle w +m\angle x\\\\
m\angle y +m\angle z= m\angle w +m\angle x\\\)
Hence proved
2. In \( \triangle PQS, \: \angle QSR \) is exterior angle.
Therefore, by remote interior angle theorem, we have:
\( m\angle QPS + m\angle PQS= m\angle QSR\\\\
x + m\angle PQS= 2x\\\\
m\angle PQS= 2x-x\\\\
m\angle PQS = x.... (1)\\\\
m\angle QPS = x.... (given).... (2)\\\)
From equations (1) & (2), we find:
\( m\angle PQS = m\angle QPS\\\\
\therefore \angle PQS \cong \angle QPS\\\\
\therefore PS \cong QS\\(sides\: opposite \: to\: congruent \:\angle s) \\\\\)
\( \therefore \triangle PQS \) is an isosceles triangle.
Thus proved.
Find the volume of the composite figure shown

Answers
Answer:
480 inches^3
Step-by-step explanation:
The volume of a Prism is: Bh
B= length of the base
h= height of the lateral face
(8*10)4
320 inches^3
So for the 2nd figure on top you do the same.
This time you divide by 2.
So it's 160 inches^3.
Add up you get a sum of 48- inches^3.
Or 480 cubic inches.
the quality control manager of green bulbs inc. is inspecting a batch of energy saving compact fluorescent light bulbs. when the production process is in control, the mean number of bad bulbs per shift is 6.0. what is the probability that any particular shift being inspected has produced fewer than 5 0 bad bulbs.
Answers
The probability that any particular shift being inspected has produced fewer than 50 bad bulbs is 0.2851.
The mean number of bad bulbs per shift is 6.0.
The probability mass function of Poisson distribution is:
P(X=x)=e^(−λ)λ^x/x!
The Poisson distribution is used to find the given probability.
The probability that any particular shift being inspected has produced fewer than 5 0 bad bulbs is:
P(x<5)=P(x≤4)
=P(x=0)+P(x=1)+P(x=2)+P(x=3)+P(x=4)
=\(e^{-6}\cdot\frac{6^0}{0!}+e^{-6}\cdot\frac{6^1}{1!}+e^{-6}\cdot\frac{6^2}{2!}+e^{-6}\cdot\frac{6^3}{3!}+e^{-6}\cdot\frac{6^4}{4!}\)
=0.0025+0.0149+0.0446+0.0892+0.1339
=0.2851
The required probability is obtained by substituting the values of mean and x in Poisson probability mass function.
The probability that any particular shift being inspected has produced fewer than 50 bad bulbs is 0.2851.
To learn more about probability link is here
brainly.com/question/18882393
#SPJ4
HELP ASP PLEASE (10 POINTS)

Answers
According to the information the area of the figure is 264 in²
How to find the area of the figure?To find the area of the figure we must take into account the dimensions of its sides. In this case we have a 16in by 9in rectangle. We must multiply these two values to find the area of this rectangle:
9 * 16 = 144in²On the other hand, we have a triangle at the top that is 15in high by 16in wide. In this case we multiply the same as to find the area of the rectangle and then divide by two.
16in * 15in = 240in²240in² / 2 = 120in²Finally we add the pair of both elements to find the total area:
120in² + 144in² = 264in²Learn more about area in: https://brainly.com/question/16151549
#SPJ1
An engineer has a 50:1 scale drawing of a bridge. The dimensions of the scaled bridge deck are 24 inches by four and one fifth inches. What is the area of the actual bridge deck in square feet?
120 square feet
875 square feet
1,750 square feet
3,500 square feet
Answers
Thus, the area of the actual bridge deck is found as 1750 square feet.
Explain about the scale factor:A scale factor refers to the amount by which an object is multiplied to produce a second object of different size but with the same appearance. Just a larger or smaller version of the original is created, not an exact copy.
Given dimension-
Length l = 24 inches
width w = 4 1/5 = 21/5 inches
Convert inches to feet:
1 foot = 12 inches.
Scaled length = 24 inches ÷ 12 inches/foot = 2 feet
Scaled width =21/5 inches ÷ 12 inches/foot = 21 / 5*12 = 0.35 feet
Now, scale factor = 50.: 1
Actual length = Scaled length × Scale factor
Actual length = 2 feet × 50 = 100 feet
Actual width = Scaled width × Scale factor
Actual width = 0.35 feet × 50 = 17.5 feet
Area of actual bridge deck:
Area = Actual length × Actual width
Area = 100 feet × 17.5 feet
Area = 1750 square feet
Thus, the area of the actual bridge deck is found as 1750 square feet.
Know more about the scale factor:
https://brainly.com/question/25722260
#SPJ1
I WILL GIVE BRAINLIEST PLS HELP!
Melissa and Jaime are playing a game in geometry class. Melissa draws the shape below on plain white paper and has to give Jaime directions to draw a congruent shape.
I. The location of each vertex on a coordinate plane
II. The shape is a triangle. Its two longest sides measure 6 inches and 3√3 inches.
III. m∠MEL = 90°, m∠ELM = 30°, and LM = 6 inches
Which of the following descriptions of Melissa's triangle is adequate for Jaime to draw a congruent shape?

Answers
Answer:
III. m∠MEL = 90°, m∠ELM = 30°, and LM = 6 inches
Step-by-step explanation:
It´s lll Because to make a congruent shape one angle should be 90 and the longest side should be 6 inches. one angle should equal angle ELM.
So, by AAS criteria it would be congruent.
---------------------------
hope it helps...
have a great day!!
Answer:
III. m∠MEL = 90°, m∠ELM = 30°, and LM = 6 inches
Step-by-step explanation:
hope it helps you...
N students joined the radio control club. Some had boats, some had airplanes, and some had cars. They divided into 3 equal groups according to what model each one had. Ten more students joined the radio-controlled airplane group. There are 15 students in this group now. How many students joined the radio control club in the beginning?
Answers
Answer:
36 people joined the radio control club.
Step-by-step explanation:
15-3= 12
12*3=36
the scale is 1 inch=50 miles. 5 inches is what
Answers
Answer:
Step-by-step explanation:
you multiply 5 by 50 and that would equal 250
5 x 50 = 250
what is the domain of f(x)=5^x?
Answers
Answer:
Domain = {x | x is a real number}
Look at the step-by-step explanation to get a better understanding
Step-by-step explanation:
Domain = {x | x is a real number}
The function shown f(x)=5^x-7 is an exponential function with a vertical shift of -7 units (shift downwards). Nevertheless, it is an exponential function.
Domain is the set of x-values that we can put into the function. Exponential functions of this type have a domain of "all real numbers". If you see the function, you can figure out that we can put any real number and still there will be no "undefined" or "division by zero" cases.
Thus, this function's domain is the set of all real numbers.
Domain = {x | x is a real number}
Hope you fond this helpful! :)
Answer:
all real numbers
Step-by-step explanation:
hi guys can you please help me with this !! i’d appreciate it a lot

Answers
Step-by-step explanation:
I am assuming we are deciding which value is greater:
1. 3/3 < 8/7
2. 4/6 < 3/3
3. 6/9 = 2/3
4. 9/9 < 3/2
5. 2/2 < 5/4
6. 1/2 = 2/4
7. 1/8 < 3/5