A circle has a radius of 6 inches and a central angle of 60°. What is the measure of the arc length associated with this angle?
Answers
Answer:
\(2\pi\)
Step-by-step explanation:
first you take 60°/360°= 1/6 then you take 1/6 and multiple it by \(2\pi\) and that equals \(\frac{\pi }{3}\) finally take \(\frac{\pi }{3}\) times the radius (6) and that should equal \(2\pi\)
PLATO USERS #platolivesmatters
The measure of the arc length associated with the angle is 2π inches OR 6.28 inches
Calculating the length of an arcFrom the question, we are to determine the measure of the arc length
The measure of the arc length can be calculated by using the formula,
\(l = \frac{\theta}{360 ^\circ}\times 2\pi r\)
Where \(l\) is the length of the arc
θ is the central angle
r is the radius of the circle
From the given information,
r = 6 inches
θ = 60°
Putting the parameters into the formula, we get
\(l = \frac{60 ^\circ}{360 ^\circ}\times 2 \pi \times 6\)
\(l = \frac{1}{6}\times 2 \pi \times 6\)
\(l =2\pi\) inches OR 6.28 inches
Hence, the measure of the arc length associated with the angle is 2π inches OR 6.28 inches
Learn more on Calculating the length of an arc here: https://brainly.com/question/12541510
Related Questions
Which statement describes the transformation of figure 2 into figure 3?

Answers
Answer:
2 reflection across the y axis
COULD YALL HELP ME ?!???!

Answers
Answer:
28 degrees
Step-by-step explanation:
We know that (4x + 7) + (2x + 5) add up to a straight angle = 180 degrees, so we have the equation 4x + 7 + 2x + 5 = 180.
By combining like terms, we get 6x + 12 = 180.
Subtract 12 from both sides of the equation to get 6x = 168. Divide both sides by 6 and you get x = 28.
Which of the following side lengths DO NOT make a right triangle?
6-8-10
3-4-5
6-8-9
9-12-15
EXPLAIN HOW YOU FIGURED IT OUT!!! PLEASE
Answers
\(6, 8, 9\) is NOT a right one because \(6^2+8^2\neq9^2\).
Hope this helps.
Pre - Calculus evaluate exponential derivative at a point !

Answers
Answer:
\(\displaystyle\)\(\displaystyle f'(1)=-\frac{9}{e^3}\)
Step-by-step explanation:
Use Quotient Rule to find f'(x)
\(\displaystyle f(x)=\frac{3x^2+2}{e^{3x}}\\\\f'(x)=\frac{e^{3x}(6x)-(3x^2+2)(3e^{3x})}{(e^{3x})^2}\\\\f'(x)=\frac{6xe^{3x}-(9x^2+6)(e^{3x})}{e^{6x}}\\\\f'(x)=\frac{6x-(9x^2+6)}{e^{3x}}\\\\f'(x)=\frac{-9x^2+6x-6}{e^{3x}}\)
Find f'(1) using f'(x)
\(\displaystyle f'(1)=\frac{-9(1)^2+6(1)-6}{e^{3(1)}}\\\\f'(1)=\frac{-9+6-6}{e^3}\\\\f'(1)=\frac{-9}{e^3}\)
Answer:
\(f'(1)=-\dfrac{9}{e^{3}}\)
Step-by-step explanation:
Given rational function:
\(f(x)=\dfrac{3x^2+2}{e^{3x}}\)
To find the value of f'(1), we first need to differentiate the rational function to find f'(x). To do this, we can use the quotient rule.
\(\boxed{\begin{minipage}{5.5 cm}\underline{Quotient Rule for Differentiation}\\\\If $f(x)=\dfrac{g(x)}{h(x)}$ then:\\\\\\$f'(x)=\dfrac{h(x) g'(x)-g(x)h'(x)}{(h(x))^2}$\\\end{minipage}}\)
\(\textsf{Let}\;g(x)=3x^2+2 \implies g'(x)=6x\)
\(\textsf{Let}\;h(x)=e^{3x} \implies h'(x)=3e^{3x}\)
Therefore:
\(f'(x)=\dfrac{e^{3x} \cdot 6x -(3x^2+2) \cdot 3e^{3x}}{\left(e^{3x}\right)^2}\)
\(f'(x)=\dfrac{6x -(3x^2+2) \cdot 3}{e^{3x}}\)
\(f'(x)=\dfrac{6x -9x^2-6}{e^{3x}}\)
To find f'(1), substitute x = 1 into f'(x):
\(f'(1)=\dfrac{6(1) -9(1)^2-6}{e^{3(1)}}\)
\(f'(1)=\dfrac{6 -9-6}{e^{3}}\)
\(f'(1)=-\dfrac{9}{e^{3}}\)
Find the value of each variable. Round to the nearest tenth, if necessary.

Answers
16.7, 6.7 and 22 degrees respectively are the measures of a, c and m<C
Solving trigonometry identityThe given diagram is a triangle with the following sides
Hypotenuse = AC = 18
m<A = 68 Degrees
We need to determine the measure of a, b and m<C
Using the trigonometry identity
sin 68 = a/18
a = 18sin68
a = 16.7
Similarly;
cos68 = c/18
c = 18cos68
c = 6.7
Since the sum of angles in a triangle is 180 degrees, hence:
m<C = 90 - m<A
m<C = 90 - 68
m<C = 22 degrees
Hence the measure of a, c and m<C is 16.7, 6.7 and 22 degrees respectively.
Learn more on triangles here: https://brainly.com/question/1058720
#SPJ1
What is the volume of a cube with
inch sides?
2
1

Answers
Answer/Step-by-step explanation:
The given park of sugar cubes has has 24 cubes of sugar.
The cube of sugar has equal side length of ½ in.
Volume of the park of sugar cubes = 24 * volume of 1 cube of sugar
Volume of 1 cube of sugar = \( \frac{1}{2} * \frac{1}{2} * \frac{1}{2} \)
= \( \frac{1}{8} in^3 \)
Volume of the park of sugar cubes = \( 24 * \frac{1}{8} = \frac{24}{8} = 3 in^3 \)
Two planes, which are 3950 miles apart. fly toward each other. Their speeds differ by 40 mph. If they pass each other in 5 hours, what is the speedof each?Answer How to enter your answer (opens in new window) 3 PointsKeypad

Answers
Given the word problem, we can deduce the following information:
1. The two planes are 3950 miles apart.
2. Their speeds differ by 40 mph.
3. Time =5 hours
To determine the speed of each plane, we first let:
x= speed of the fist plane
x+40 =speed of the second plane
Based on the above information, the combined speed is 2x+40. Our equation would be:
\(2x+40=\frac{3950}{5}\)Next, we find the value of x:
\(\begin{gathered} 2x+40=\frac{3950}{5} \\ \text{Simplify and rearrange} \\ 2x+40=790 \\ 2x=790-40 \\ 2x=750 \\ x=\frac{750}{2} \\ \text{Calculate} \\ x=375 \end{gathered}\)Hence,
x= speed of the fist plane=375 mph
x+40 =speed of the second plane=375+40= 415 mph
May god be with you but help me

Answers
Answer:
150
Step-by-step explanation:
because 30 x 5 =150
Answer:
150°
Step-by-step explanation:
Here,
In linear pair we get the rest of its side angle we get is,
30° + x(unknown value) = 180°
=> x = 150°
Now,
Angle 1 = x. [As corresponding Angles]
So , value of 1 is 150° (Ans)
Find the inverse of the given function. (pictured below)

Answers
Answer:
4
3
0
Step-by-step explanation:
f(x) = y = -1/2 × sqrt(x+3)
2y = -sqrt(x+3)
4y² = x + 3
x = 4y² - 3
now renaming this, so that the normal symbols and names are used for this function definition, so that the input variable is called "x" :
f-1(x) = 4x² - 3
basically, just by itself, this function would be defined for all possible real values of x.
but because it is the inverse of the original function, which generates only values of y<=0, then for the inverse function that same range applies for its input variable x
x<=0
Help please!
S = 180m
How many strides would a runner take during a 1-hour run (60 min)
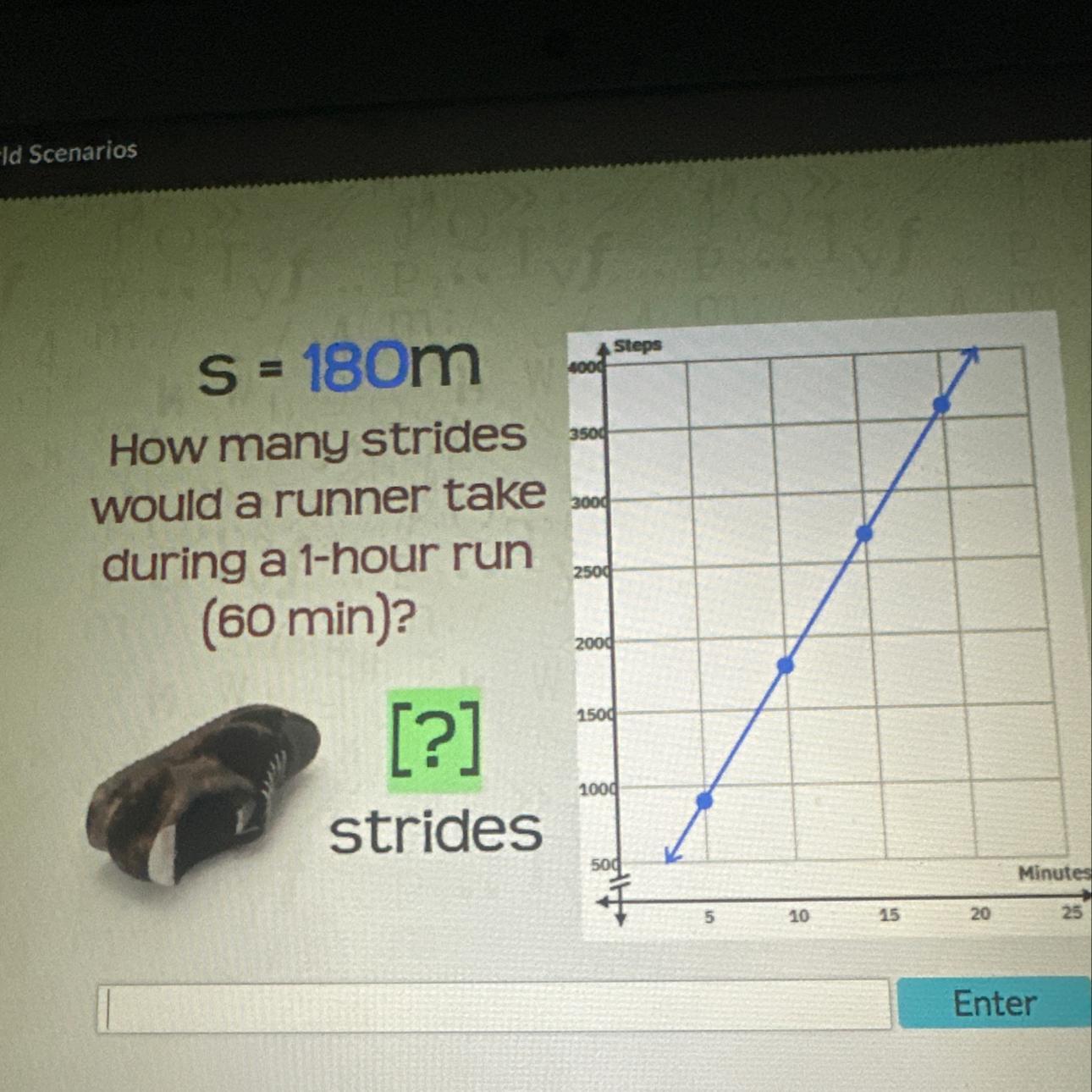
Answers
The number of strides the runner will make during 1 hour run will be =
10,800.
How to calculate the total number of strides made by the runner in a hour?To calculate the distance or number of strides made by the runner in an
hour, the graph given above is considered as follows;
The y-axis which represents the number of strides is plotted against the x-axis which is the number of hours.
From the graph
20 minutes= 3600 strides
60 minutes = X strides
make X the subject of formula;
x= 3600×60/20
= 216000/20
= 10,800
Learn more about distance here:
https://brainly.com/question/30694816
#SPJ1
in baseball Anthony hit 0.63 of the pitches thrown at him what percent of the pitches did Anthony miss
Answers
A cuboid measures 6cm by 3cm by 2cm
Draw a net of the cuboid
Answers
Answer:
See attachment for cube
Step-by-step explanation:
Given
\(Length = 6cm\)
\(Width = 3cm\)
\(Height = 2cm\)
Required
The net of the cuboid
A cuboid has 6 faces.
The dimension of these faces are:
Length and Width; Length and Height;
Length and Width; Length and Height;
Width and Height; Width and Height;
This means that the faces will have the following dimensions:
6cm by 3cm; 6cm by 2cm
6cm by 3cm; 6cm by 2cm
3cm by 2cm; 3cm by 2 cm
Taking into account the above measurements and dimensions, you draw 6 faces, then join similar sides lengths.
See attachment for net of the cuboid

Consider the following equations:
y1 = 2x + 7
y2 = 1.5x + 4
Compare the values of y1 and y2 for x < −7 and for x > −5. Create a table and solve this system of equations.
Answers
The solution of the system of equation is given by the point where the
ordered pair of each equation are equal.
The solution of the equation system is x = -6
Reasons:
y₁ = 2·x + 7
y₂ = 1.5·x + 4
The values of y₁ for x < -7 are;
y₁₍₋₈₎ = 2 × (-8) + 7 = -9
y₁₍₋₉₎ = 2 × (-9) + 7 = -11
y₁₍₋₁₀₎ = 2 × (-10) + 7 = -13
Therefore, we get;
The values of y₁ for x < -7 = -9, -11, -13,...
The values of y₂ for x < -7 are;
y₂₍₋₈₎ = 1.5 × (-8) + 4 = -8
y₂₍₋₉₎ = 1.5 × (-9) + 4 = -9.5
y₂₍₋₁₀₎ = 1.5 × (-10) + 4 = -11
The values of y₂ for x < -7 are; -8, -9.5, -11
The values of y₁ for x > -5 are;
y₁₍₋₄₎ = 2 × (-4) + 7 = -1
y₁₍₋₃₎ = 2 × (-3) + 7 = 1
y₁₍₋₂₎ = 2 × (-2) + 7 = 3
Therefore, we get;
The values of y₁ for x > -5 = -1, 1, 3
The values of y₂ for x > -5 are;
y₂₍₋₄₎ = 1.5 × (-4) + 4 = -2
y₂₍₋₃₎ = 1.5 × (-3) + 4 = -0.5
y₂₍₋₂₎ = 1.5 × (-2) + 4 = 1
The values of y₂ for x > -5 are; -2, -0.5, 1, ...
The table is presented as follows;
\(\begin{tabular}{|c|r|r|}x&y_1&y_2\\-2&3&1\\-3&1&-0.5\\-4&-1&-2\\-5&-3&-3.5\\-6&-5&-5\\-7&-7&-6.5\\-8&-9&-8\end{array}\right]\)
From the above table the solution to the system of equation (the value of x
at which y₁ = y₂) is x = -6.
Lean more here:
https://brainly.com/question/15987573
izza izza izza izza izza izza
Answers
James T-shirt business uses the demand function P = -Q+31 and the supply function P = Q-17. According to these functions, what will the
equilibrium point (P.Q) be for James T-shirt business?
Answers
The equilibrium point for James T-shirt business is (P, Q) = (7, 24).
To find the equilibrium point (P, Q) for James T-shirt business
we need to set the demand function equal to the supply function and solve for the values of P and Q.
Demand function: P = -Q + 31
Supply function: P = Q - 17
Setting them equal:
-Q + 31 = Q - 17
Adding Q to both sides and subtracting 31 from both sides:
2Q = 48
Dividing both sides by 2:
Q = 24
Now, we can substitute this value of Q into either the demand or supply function to find the corresponding value of P.
P = Q - 17
P = 24 - 17
P = 7
Therefore, the equilibrium point for James T-shirt business is (P, Q) = (7, 24).
To learn more on Functions click:
https://brainly.com/question/30721594
#SPJ1
Find the value of each variable

Answers
y is 29
hope that's right
E09 Review
Scores on "The ability to do quantitative thinking" test are normally distributed with a mean of 250 and a standard deviation of
25. Brandi scored at the 81st percentile on this test. What was her test score?
is the test score for Brandi.
Answers
Answer:
272 is the test score for Brandi.
Step-by-step explanation:
Normal Probability Distribution:
Problems of normal distributions can be solved using the z-score formula.
In a set with mean \(\mu\) and standard deviation \(\sigma\), the z-score of a measure X is given by:
\(Z = \frac{X - \mu}{\sigma}\)
The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.
Mean of 250 and standard deviation of 25:
This means that \(\mu = 250, \sigma = 25\)
Brandi scored at the 81st percentile on this test. What was her test score?
The z-score of her score of X has a p-value of 0.81. This means that her score is given by X when Z = 0.88.
\(Z = \frac{X - \mu}{\sigma}\)
\(0.88 = \frac{X - 250}{25}\)
\(X - 250 = 0.88*25\)
\(X = 272\)
272 is the test score for Brandi.
You determined that you can make 72 donuts in 2hours. At rate,how many donuts can you make in 8 hours?
Answers
Answer:
288 donuts
Step-by-step explanation:
Answer:
288 donuts
Step-by-step explanation:
72 divided by 2 is 36 so that means you will make 36 donuts in 1 hour so now we have our unit rate which is 36. so then you multiply 36 by 8 and you get 288. that is your answer
Which expressions describe the end behavior of the graph.

Answers
how do I find range and domain
Answers
DOMAIN: to find the domain set the bottom equal to zero and exclude the X value you find when you solve the equation.
*Please help*
Xavier bought a new boat for $24,000 in the year 2009. He knows that the value of the boat has depreciated linearly. If the value of the boat in 2010 was $18,500, what was the annual rate of change of the boat's value? Round your answer to the nearest hundredth if necessary.
Answers
Answer:
5500 is correct Answer.
Please mark me as brainlist answer.
what are the factor of pair of number?
a.45 and 60
b.45 and 70
c.40 and 80
d.30 and 50
Answers
I need 2 expressions that are equal to 30m+15 when you solve them! Please be quick!
Answers
Answer:
\(30m + 15 = 3(10m + 5)\\\)
\(30m + 15 = 5(6m+3)\)
Step-by-step explanation:
Given
\(30m + 15\)
Required
Equivalent expressions
We have:
\(30m + 15\)
Factor out 3
\(30m + 15 = 3(10m + 5)\)
Also:
\(30m + 15\)
Factor out 3
\(30m + 15 = 5(6m+3)\)
name the transversal
A. Line L
B. Line M
C. Line N
D. There is no transversal

Answers
Which one correct answer??

Answers
What is the question of it ?
lim (cosec 2x - xcosec³x)
x approaches 0
Answers
The limit lim(x approaches 0) (cosec(2x) - x*cosec³(x)) is undefined.
We have,
To evaluate the limit of the expression as x approaches 0, we can simplify the expression first.
The expression is given as lim(x approaches 0) (cosec(2x) - x cosec³(x)).
Using trigonometric identities, we can rewrite cosec(2x) as 1/sin(2x) and cosec³(x) as 1/(sin(x))³.
Substituting these into the expression,
We get lim(x approaches 0) (1/sin(2x) - x (1/(sin(x))³)).
Now, let's evaluate the limit term by term:
lim(x approaches 0) (1/sin(2x)) = 1/sin(0) = 1/0 (which is undefined).
lim(x approaches 0) (x (1/(sin(x))³))
= 0 (1/(sin(0))³)
= 0 x 1/0 (which is also undefined).
Since both terms of the expression are undefined as x approaches 0, we cannot determine the limit of the expression.
Therefore,
The limit lim(x approaches 0) (cosec(2x) - x*cosec³(x)) is undefined.
Learn more about limits here:
https://brainly.com/question/12211820
#SPJ1
Find an equation for the tangent to the curve at the given point. Then sketch the curve and the tangent together. y = 8 , (4,16) y = Choose the correct graph of the curve and the tangent below.
Answers
The equation of tangent to the curve y = 8√x at the point (4,16) is y = 2x + 8 .
We have to find equation of tangent line to the curve y = 8√x at the point (4, 16), we first find the slope ;
So , slope of the tangent line is derivative of function y = 8√x at point (4, 16).
which means : y' = 4\(x^{-\frac{1}{2} }\) ;
At the point (4, 16), the value of x is 4.
So , y' = 4 × \(4^{-\frac{1}{2} }\) = 2 .
By Using the point slope form, the equation of the tangent line is ;
⇒ y - 16 = (2)(x - 4) ;
Simplifying this equation, we get:
⇒ y - 16 = 2x - 8
⇒ y = 2x + (16 - 8)
⇒ y = 2x + 8 .
Therefore, the equation of the tangent line to the curve is y = 2x + 8 .
Learn more about Tangent here
https://brainly.com/question/28994024
#SPJ4
The given question is incomplete , the complete question is
Find an equation for the tangent to the curve y = 8√x at the point (4,16) .
a truck with 34 -inch diameter wheels is traveling at 55 mi/h. round answers to the nearest whole number.
Answers
The angular speed of the wheels in radians per minute is 0.033 and the number of revolutions per minute do the wheels make is 0.0052
In this case, we are dealing with a truck with wheels that have a diameter of 34 inches. The circumference of the wheels is equal to the diameter multiplied by pi, or 34 x pi, which is approximately 106.81 inches. To convert this to miles per hour, we need to divide it by the number of inches in a mile, which is 63,360. Thus, the linear speed of the truck is:
v = (106.81/63360) x 55 = 0.09375 miles per minute
Next, we need to calculate the angular speed of the wheels in radians per minute. The angular speed is defined as the number of radians covered by the wheel in one minute, and it can be calculated using the formula:
ω = v/r
where ω is the angular speed, v is the linear speed, and r is the radius of the wheel. Since we are given the diameter of the wheel, we need to divide it by 2 to get the radius, which is:
r = 34/2 = 17 inches
Substituting the values for v and r into the formula for angular speed, we get:
ω = (0.09375)/(17 x pi/60) ≈ 0.033 radians per minute
Finally, we need to calculate the number of revolutions per minute that the wheels make.
This can be done by dividing the angular speed by 2pi, which is the number of radians in one revolution. Thus, the number of revolutions per minute is:
n = ω/(2pi) ≈ 0.0052 revolutions per minute
To know more about speed here
https://brainly.com/question/4199102
#SPJ4
Complete Question:
A truck with 34 inch diameter wheels is traveling at 55mi/h. Find the angular speed of the wheels in radians per minute? And how many revolutions per minute do the wheels make?
Write the equation of the line passing through the points (-7, 5) and (7, 1)
Answers
Answer:
y = -2/7(x) + 3
Step-by-step explanation:
The equation of a line can be stated as y = mx + b, where m is the slope and b is the y-intercept (the value of the function when x = 0). To find the equation of the line, we'll start by finding the slope.
The slope can be expressed as \(\frac{y_{2} - y_{1}}{x_{2}-{x_1}}\)
Substituting in our coordinates, we have:
\(m = \frac{1 - 5}{7 - (-7)} = \frac{-4}{7 + 7} = \frac{-4}{14}\), which can be simplified to \(-\frac{2}{7}\)
Plugging that back into our equation, we have y = \(-\frac{2}{7}\)x + b
Now, to find b, we substitute in one of our sets of coordinates. Let's use (-7, 5)
x = -7 and y = 5, which gives us:
\(5 = -\frac{2}{7} (-7) + b\)
\(-\frac{2}{7} (-7) = 2\), which gives us:
5 = 2 + b
Subtracting 2 from both sides, we get:
3 = b
Plugging that back into our equation, we have \(y = -\frac{2}{7} x + 3\)
4. Expand and simplify the product of
(x-a)?
Answers
Step-by-step explanation:
x-a