Find the change-of-coordinates matrix from B to the standard basis in R2 B = [ 4 ] [ 1 ]
[-2 ] [ 3 ]
The change of coordinates matrix is ____
Answers
The change-of-coordinates matrix from B to the standard basis in R2 is [ 4 -1 ] [ 1 0 ].
The change of coordinates matrix from B to the standard basis in R2 is [ 4 -1 ] [ 1 0 ].Explanation:A standard basis is the collection of vectors that represent the values of the n-dimensional coordinate axes, with each coordinate axis represented by a unit vector.For an n-dimensional space, the standard basis vectors e1, e2, e3,... en are the column vectors of the identity matrix n × n.In this question, we need to find the change of coordinates matrix from B to the standard basis in R2.B = [ 4 ] [ 1 ]We need to represent B as a linear combination of the standard basis vectors: B = 4e1 + 1e2. So, the matrix that represents B is[ 4 ] [ 1 ] = 4[1 0] + 1[0 1]Next, we write this in matrix form, using the coefficients of the standard basis vectors as columns: [1 0] [0 1] [ 4 1 ]Therefore, the change-of-coordinates matrix from B to the standard basis in R2 is [ 4 -1 ] [ 1 0 ].
Learn more about Change-of-coordinates
brainly.com/question/12661885
#SPJ11
Related Questions
Surface Area of this triangular
prism?

Answers
What is the Value of the expression below when y=4 and z=4?
6y-3z
Answers
Answer: 12
Step-by-step explanation:
6y - 3z
6(4) - 3(4)
24 - 12
12
Solve for x and show the steps

Answers
Answer:
Step-by-step explanation:
All you need do is divide by m and you have the answer.
x*m/m = np/m
x = np/m
PLS SOMEONE HELP ME URGENTLY PLS

Answers
The vector z in the component form is z = < 21 , 24 , -27 >
Given data ,
A vector in component form is typically written as an ordered pair or triplet, where each component represents the magnitude of the vector along a specific coordinate axis.
Now , the vector u = < -1 , 3 , 1 >
v = < 4 , -3 , -1 >
w = < 10 , 5 , -10 >
Now , the value of vector z = < 3w - 2v + u >
z = 3w - 2v + u
z = 3w - 2 * < 4 , -3 , -1 > + < -1 , 3 , 1 >
Using scalar multiplication, we get:
z = < 30 , 15 , -30 > - < 8 , -6 , -2 > + < -1 , 3 , 1 >
Adding vectors, we get:
z = < 30 - 8 - 1 , 15 - (-6) + 3 , -30 + 2 + 1 >
z = < 21 , 24 , -27 >
Hence , the vector z in component form is z = < 21 , 24 , -27 >
To learn more about product of vectors click :
https://brainly.com/question/11044032
#SPJ1
obi's age is twice oba's age 4 years ago obi was three times as old as oba. find their ages
Answers
Answer:
Obi's age is 18 and Oba's age is 9
Step-by-step explanation:
Let Obi's age be x and Oba's age be y
Condition 1:
x = 2y -----------(1)
Condition 2:
(x-4) = 3(y-4)
=> x -4 = 3y-12
=> x - 3y = -9 -----(2)
Putting eq (1) in (2)
2y - 3y = -9
=> -y = -9
=> y = 9
Now,
x = 2y
=> x = 2(9)
=> x= 18
Answer:
\(8\\4\)
Step-by-step explanation:
Obi's age is \(x\).
Oba's age is \(2x\).
4 years ago, Obi's age was \(x-4\).
4 years after, Obi's age is \(2x-4\)
3 times more than Oba's, so \(3(x-4)\)
\(2x - 4 = 3( x - 4)\)
\(2x - 4 = 3x-12\)
\(2x - 3x = -12+4\)
\(-1x=-8\)
\(x=8\)
\(x-4\\(8)-4=4\)
Which polynomial correctly combines the like terms and puts the given polynomial in standard form?.
Answers
The polynomial 8x⁴ - (2/3)x² - (2/3)x correctly combines the like terms and puts the polynomial (1/3)x + 8x⁴ - (2/3)x² - x in the standard form option (C) is correct.
What is polynomial?
Polynomial is the combination of variables and constants systematically with "n" number of power in ascending or descending order.
The question is incomplete.
The complete question is in the picture, please refer to the attached picture.
It is given that:
The polynomial:
(1/3)x + 8x⁴ - (2/3)x² - x
Adding combine like terms:
= 8x⁴ - (2/3)x² + (1/3)x - x
= 8x⁴ - (2/3)x² - (2/3)x
Thus, the polynomial 8x⁴ - (2/3)x² - (2/3)x correctly combines the like terms and puts the polynomial (1/3)x + 8x⁴ - (2/3)x² - x in the standard form option (C) is correct.
To learn more about Polynomial visit : brainly.com/question/20121808
#SPJ4

dy cos Deinz What is the general solution to the differential equation da --- ? COS Y A y = arcsin (esin x) + + B 2 y = arcsin (esin æ $C) с y=sin x + arcsin(C) D y = arcsin(sin xe cosa +C)
Answers
The general solution to the given differential equation dy/dx = (cos y)/(a cos x) can be expressed as y = arcsin(e sin x) + C, where C is an arbitrary constant.
The general solution to the given differential equation dy/dx = (cos y)/(a cos x) is y = arcsin(e sin x) + C, where C is an arbitrary constant. This solution is obtained by integrating both sides of the differential equation with respect to x and solving for y.
To solve the differential equation dy/dx = (cos y)/(a cos x), we first observe that the equation involves the trigonometric function cosine (cos) of y and x. By rearranging the equation, we can separate the variables y and x on opposite sides of the equation. Then, we can integrate both sides with respect to x, treating y as a constant, to obtain the equation y = arcsin(e sin x) + C, where C represents the constant of integration. This equation represents the general solution to the given differential equation, as it satisfies the original equation for all values of x and corresponding values of y. The arbitrary constant C allows for different possible solutions within the family of curves defined by the equation.
To learn more about general solution : brainly.com/question/32062078
#SPJ11
One year, the mean age of an inmate on death row was 38.8 years. A sociologist wondered whether the mean age of a death-row inmate has changed since then. She randomly selects 32 death-row inmates and finds that their mean age is 37.5, with a standard deviation of 9.2. Construct a 95% confidence interval about the mean age. What does the interval imply
Answers
A 95% confidence interval about the mean age is (34.31, 40.69)
We know that, the confidence interval is:
\((\bar{x}-z\frac{\sigma}{\sqrt{n} },~ \bar{x}+z\frac{\sigma}{\sqrt{n} } )\)
where,
\(\bar{x}\) is the sample mean.
z is the critical value.
n is the sample size.
σ (OR s) is the standard deviation for the population.
For given question,
A sociologist randomly selects 32 death-row inmates and finds that their mean age is 37.5, with a standard deviation of 9.2
we need to calculate the 95% confidence interval about the mean age.
For 95%, the critical value is z = 1.96
We have been given x¯ = 37.5, σ = 9.2, and n = 32
So, the confidence interval would be,
\((37.5-1.96\frac{9.2}{\sqrt{32} },~ 37.5+1.96\frac{9.2}{\sqrt{32} } )\)
= (37.5 - 1.96(1.63), 37.5+ 1.96(1.63))
= (37.5 - 3.19, 37.5 + 3.19)
= (34.31, 40.69)
A 95% confidence interval for each sample, then approximately 95 of the 100 confidence intervals will contain the true mean value.
Therefore, a 95% confidence interval about the mean age is (34.31, 40.69)
Learn more about the confidence interval here:
brainly.com/question/28064687
#SPJ4
Find the measures of each numbered angles
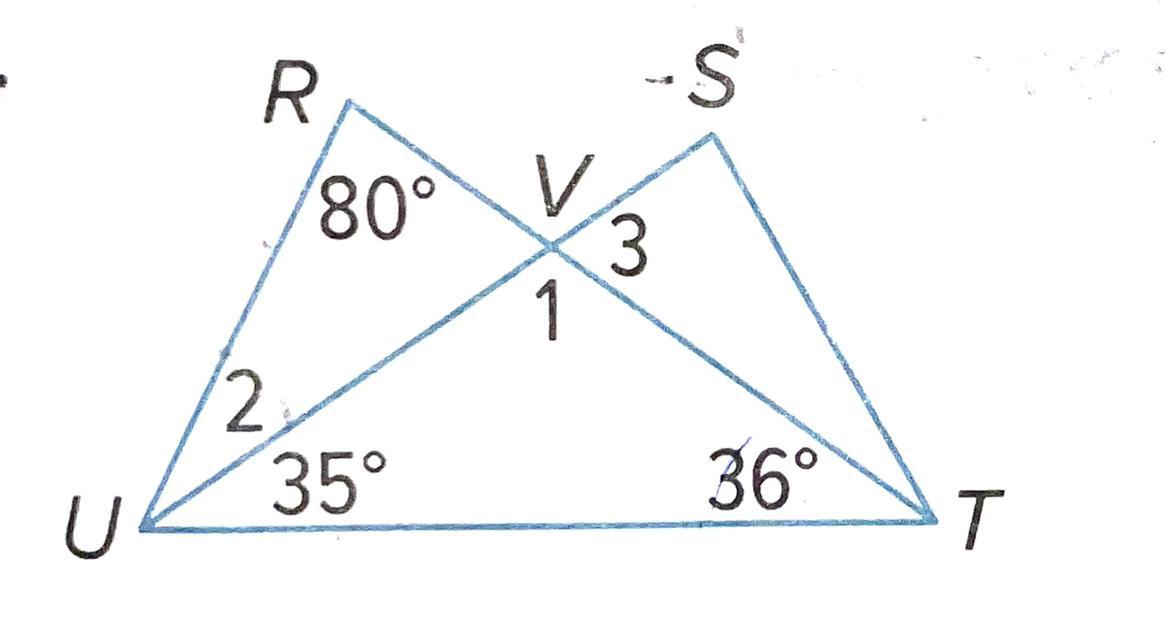
Answers
Answer:
∠ 1 = 109° , ∠ 2 = 29° , ∠ 3 = 71°
Step-by-step explanation:
The sum of the 3 angles in a triangle = 180° , then
∠ 1 + 35° + 36° = 180°
∠ 1 + 71° = 180° ( subtract 71° from both sides )
∠ 1 = 109°
-------------------
∠ RVU and ∠ 1 are adjacent angles on a straight line and sum to 180° , then
∠ RVU + ∠ 1 = 180°
∠ RVU + 109° = 180° ( subtract 109° from both sides )
∠ RVU = 71°
In Δ RVU
∠ 2 + 80° + 71° = 180° ( sum of angles in triangle )
∠ 2 + 151° = 180° ( subtract 151° from both sides )
∠ 2 = 29°
-------------------------
∠ 3 and ∠ RVU are vertically opposite angles and are congruent , then
∠ 3 = 71°
Δ UVT has: ∠V₁ = 180⁰ - ∠TUV - ∠VTU = 180⁰ - 35⁰ - 36⁰ = 109⁰
We have: ∠RVU = ∠V₃ = 180⁰ - ∠V₁ = 180⁰ - 109⁰ = 71⁰
ΔRVU has: ∠U₂ = 180⁰ - ∠URV - ∠ RVU = 180⁰ - 80⁰ - 71⁰ = 29⁰
Answer:
∠V₁ = 109⁰∠U₂ = 29⁰∠V₃ = 71⁰Ok done. Thank to me :>
I need help I’ll give Brainliest

Answers
The perimeter of the triangle DEF is half the perimeter of triangle ABC.
We are given a triangle ABC with mid-points D,E, and F of AB, BC and CA respectively.
DEF forms a triangle.
We have to find the relationship between the perimeter of triangle ABC and triangle DEF.
Let us consider sides AB,BC, and CA as 2x, 2y and 2z.
We know that triangle ABC is divided into four equal triangles of sides which are half of the sides of triangle ABC.
Hence,
DE = x,
EF = y, and
DF = z
Hence,
Perimeter of triangle ABC = 2x + 2y + 2z = 2(x + y + z)
Perimeter of triangle DEF = x + y + z
Hence, we can say that the perimeter of triangle DEF is half the perimeter of triangle ABC.
To learn more about perimeter, here:-
https://brainly.com/question/6465134
#SPJ1
Wile E. Coyote is pursuing the Road Runner across Great Britain toward Scotland. The Road Runner chooses his route randomly, such that there is a probability of 0.8 that he'll take the high road and 0.2 that he'll take the low road. If he takes the high road, the probability that Wile E. Catches him is 0.01. If he takes the low road, the probability he gets caught is 0.05.
Answers
Answer:
0.444
Step-by-step explanation:
The objective is to draw the tree diagram and find the probability that the roadrunner took the high road, given that he was caught.
The tree diagram is attached in the image below:
The required probability can be computed as:
\(P(high\ road \ | \ caught) = \dfrac{P(high \ road \ and \ caught)} {P(caught)} \\ \\ P(high \ road \ | \ caught) = \dfrac{P(high \ road \ and \ caught)}{P(high \ road \ and \ caught)+P(low \ road \ and \ caught)}\)
\(P(high \ road \ | \ caught) =\dfrac{ 0.8*0.01 }{ 0.80*0.01 + 0.20*0.05} \\ \\ P(high \ road \ | \ caught) = \dfrac{0.008 }{ 0.008 + 0.01} \\ \\\)
\(P(high \ road \ | \ caught) = \dfrac{0.008 }{ 0.018}\)
\(\mathbf{P(high \ road \ | \ caught) = 0.444}\)

The probability that he took the high road, given that he was caught is 0.444 and this can be determined by using the properties of probability.
Given :
The Road Runner chooses his route randomly, such that there is a probability of 0.8 that he'll take the high road and 0.2 that he'll take the low road. If he takes the high road, the probability that Wile E. Catches him is 0.01.If he takes the low road, the probability he gets caught is 0.05.The required probability is given by:
\(\rm P = \dfrac{P(High\;road\;and\;caught)}{P(Caught)}\)
\(\rm P = \dfrac{P(High\;road\;and\;caught)}{P(High \;road \;and \;caught)+P(Low\; road\; and \;caught)}\)
\(\rm P=\dfrac{0.8\times 0.01}{0.8\times 0.01 + 0.2\times 0.05}\)
\(\rm P=\dfrac{0.008}{0.018}\)
P = 0.444
The probability that he took the high road, given that he was caught is 0.444.
For more information, refer to the link given below:
https://brainly.com/question/23044118
for which value of c will the equation 2x - 5 = 2x - c have an infinite number of solutions? select all that appy
A 3
b 4
C 5
D 6
Answers
Answer:
d po
Step-by-step explanation:
i hope its help
carry on learning
Solve each system of the new equations by adding or subtracting

Answers
Given the equations
x + y = 5----------------------(1)
x - 3y = 3-----------------------(2)
Subtract equation (2) from (1)
x - x -3y - y = 3 - 5
-4y = -2
Divide both -4
\(\begin{gathered} \frac{-4y}{-4}\text{ = }\frac{-2}{-4} \\ y\text{ = }\frac{1}{2}\text{ = 0.5} \\ \end{gathered}\)Substitute y = 1/2 into equation (1)
x + y = 5
\(\begin{gathered} x\text{ + }\frac{1}{2}\text{ =5} \\ x\text{ = 5 -}\frac{1}{2} \\ x\text{ = }\frac{10-1}{2} \\ x=\frac{9}{2}\text{ = 4.5} \\ \end{gathered}\)Hence, the solution to the equations is
\(\begin{gathered} x\text{ = }4.5,\text{ y = 0.5} \\ Or\text{ in coordinate form, (4.5, 0.5)} \end{gathered}\)Find x. Please help with this problem!!!!!
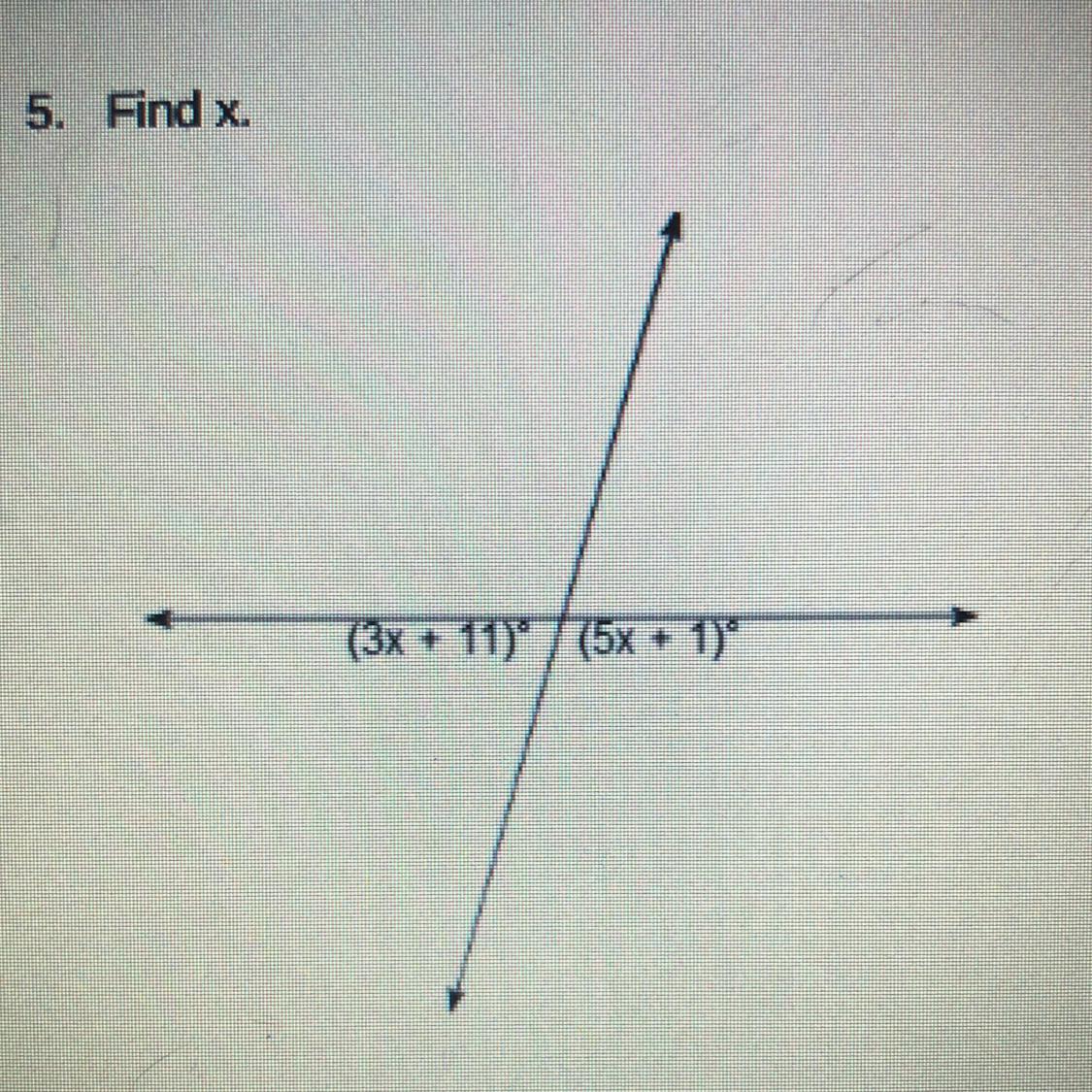
Answers
8x+12=180
8x=168
x=21
What are the year-2 CPI and the rate of inflation from year 1 to year 2 for a basket of goods that costs $25.00 in year 1 and 25.50 in year 2?
Answers
The year-2 CPI is 102, and the rate of inflation from year 1 to year 2 is 2%.
To calculate the rate of inflation and the Consumer Price Index (CPI) change from year 1 to year 2, we need to follow these steps:
Step 1: Calculate the inflation rate:
Inflation Rate = (Year 2 CPI - Year 1 CPI) / Year 1 CPI
Step 2: Calculate the Year 2 CPI:
Year 2 CPI = (Year 2 Basket Price / Year 1 Basket Price) * 100
Let's calculate the values:
Year 1 Basket Price = $25.00
Year 2 Basket Price = $25.50
Step 1: Calculate the inflation rate:
Inflation Rate = ($25.50 - $25.00) / $25.00
Inflation Rate = $0.50 / $25.00
Inflation Rate = 0.02 or 2%
Step 2: Calculate the Year 2 CPI:
Year 2 CPI = ($25.50 / $25.00) * 100
Year 2 CPI = 1.02 * 100
Year 2 CPI = 102
Therefore, the year-2 CPI is 102, and the rate of inflation from year 1 to year 2 is 2%.
For such more questions on CPI and Inflation Calculation
https://brainly.com/question/31995570
#SPJ8
1
The coefficient of x2 in the expansion of 2x +1 by 3 whole square
Answers
Answer:
1 +6 x + 12 x 2 + 8x 3
Explanation:
We must use our knowledge of the binomial expansion:
Method 1:
We can use:
(
x
+
1
)
n
=
1
+
n
x
+
n
(
n
−
1
)
2
!
x
2
+
n
(
n
−
1
)
(
n
−
2
)
3
!
x
3
+
...
Substituting
n
=
3
and
x
for
2
x
⇒
(
2
x
+
1
)
3
=
1
+
(
3
⋅
2
x
)
+
3
⋅
2
2
!
⋅
(
2
x
)
2
+
3
⋅
2
⋅
1
3
!
⋅
(
2
x
)
3
=
1
+
6
x
+
12
x
2
+
8
x
3
Method 2:
We can use:
(
A
+
B
)
n
=
A
n
+
(
n
1
)
A
n
−
1
B
1
+
(
n
2
)
A
n
−
2
B
2
+
...
Letting
A
=
2
x
and
B
=
1
for this circumstance:
(
2
x
+
1
)
3
=
(
2
x
)
3
+
(
3
1
)
(
2
x
)
2
(
1
)
+
(
3
2
)
(
2
x
)
1
(
1
)
2
+
(
3
3
)
(
2
x
)
0
(
1
)
3
=
8
x
3
+
12
x
2
+
6
x
+
1
Step-by-step explanation:
Ali and ben divide £35 in the ratio of 2:5 how much do they both get
Answers
Ali will receive £10, and Ben will receive £25 when they divide £35 in the ratio of 2:5.
How to find he ratio of 2:5 how much do they both getTo divide £35 in the ratio of 2:5, we first need to calculate the total parts of the ratio:
Total parts = 2 + 5 = 7
Next, we divide the total amount (£35) into 7 equal parts:
£35 / 7 = £5
Now, we can allocate the amounts to Ali and Ben based on the ratio:
Ali's share = 2 parts * £5 = £10
Ben's share = 5 parts * £5 = £25
Therefore, Ali will receive £10, and Ben will receive £25 when they divide £35 in the ratio of 2:5.
Learn more about ratio at https://brainly.com/question/12024093
#SPJ4
Find mZWZY.
W
x
(8x - 1)º
(5x + 11)°
Z
Answers
Answer:
08.262=)))55668(4738dfrj
please help me on this will give you brainliest

Answers
Answer:
Step-by-step explanation:
1
-
8 exponent 9
suppose we have a prime factorization of p - 1 show why the following algorithm produces a generator
Answers
The algorithm ensures that g is a generator, satisfying the necessary conditions.
To show that the algorithm produces a generator, we need to verify two conditions:
The algorithm selects a number, g, that is coprime to p-1.
The algorithm checks if g raised to the power of (p-1)/q is not congruent to 1 modulo p for each prime factor, q, of p-1.
Let's go through the steps of the algorithm to demonstrate these conditions:
Start with the prime factorization of p-1: p-1 = q1^a1 * q2^a2 * ... * qn^an, where q1, q2, ..., qn are distinct prime factors.
For each prime factor, q, calculate g = h^((p-1)/q) modulo p, where h is a randomly selected number between 2 and p-1.
Check if g is congruent to 1 modulo p. If it is, go back to step 2 and select a different h.
Repeat steps 2 and 3 until g is not congruent to 1 modulo p for each prime factor, q.
Now let's analyze these conditions:
By raising h to the power of (p-1)/q for each prime factor, the resulting g is guaranteed to be coprime to p-1. This is because g is not divisible by any prime factor of p-1.
For each prime factor, q, g^(p-1)/q is not congruent to 1 modulo p. This is because g^(p-1)/q is congruent to h^((p-1)/q * (p-1)/q) modulo p, and since h^((p-1)/q) is coprime to p, raising it to the power of (p-1)/q * (p-1)/q will not result in 1 modulo p.
Know more about algorithm here:
https://brainly.com/question/31936515
#SPJ11
Choose the best graph to fit the inequality. x < 32y2
Answers
The graph of the solution set for the inequality can be seen on the image at the end.
Which is the graph of the inequality?Here we have the following inequality:
x < 32*y^2
So x is smaller than the parabola.
First, because we have the symbol "<", we whill graph the parabola:
x = 32*y^2
With a dashed line, which means that the points on the parabola are not solutions.
Once we have that parabola graphed, we need to shade all the region to the left of the parabola, that shaded region is the solution set for the inequality.
The graph can be seen below.
Learn more about inequalities:
https://brainly.com/question/24372553
#SPJ1

The equation 2x^2 + x - 1 = 0 has two solutions. Find an equation of the form ax^2 + bx + c = 0, which solutions....
a) are by 5 larger
b) 3 time as large
Can someone please help me with this task? Thank you for your answers!
Answers
Answer:
Step-by-step explanation:
Let the solution to
2x^2 + x -1 =0
x^2+ (1/2)x -(1/2)
are a and b
Hence a + b = -(1/2) ( minus the coefficient of x )
ab = -1/2 (the constant)
A. We want to have an equation where the roots are a +5 and b+5.
Therefore the sum of the roots is (a+5) + (b+5) = a+ b +10 =(-1/2) + 10 =19/2.
The product is (a+5)(b+5) =ab + 5(a+b) + 25 = (-1/2) + 5(-1/2) + 25 = 22.
So the equation is
x^2-(19/2)x + 22 =0
2x^2-19x + 44 =0
B. We want the roots to be 3a and 3b.
Hence (3a) + (3b) = 3(a+b) = 3(-1/2) =-3/2 and
(3a)(3b) = 9(ab) =9(-1/2)=-9/2.
So the equation is
x^2 +(3/2) x -9/2 = 0
2x^2 + 3x -9 =0.
A group of boys went on a camping trip. They spent the night in 5 tents and 4 cabins. There were 9 boys in each tent and 7 boys in each cabin. How many boys went on the trip?
Answers
Answer:
Step-by-step explanation:
In a tent, there are nine boys.
There were five tents.
5 tents containing 9 boys can equal 45 boys.
(5 x 9 = 45)
In a cabin, there are seven boys.
There were four cabins.
4 cabins containing 7 boys can equal 28 boys.
(4 x 7 = 28)
Now, to find all the boys, add the numbers together.
28 boys with 45 boys will equal 73 boys.
Please check my answer for no one, not even me is right.
Good luck :)
The series ∑_(n=3)^[infinity]▒(In (1+1/n))/((In n)In (1+n)) is
convergent and sum its 1/In 3
convergent and its sum is 1/In 2
convergent and its sum is In 3
convergent and its sum is In 3/In 2
Answers
The series ∑(n=3)∞ (ln(1+1/n))/(ln(n)ln(1+n)) is convergent, and its sum is 1/ln(3).
To determine the convergence of the series, we can use the limit comparison test. Let's consider the general term of the series, aₙ = (ln(1+1/n))/(ln(n)ln(1+n)). We can compare it to a known convergent series, bₙ = 1/(nln(n)).
Taking the limit as n approaches infinity of aₙ/bₙ, we have:
lim (n→∞) (ln(1+1/n))/(ln(n)ln(1+n))/(1/(nln(n))) = lim (n→∞) [(ln(1+1/n))(nln(n))]/[(ln(n)ln(1+n))]
Using limit properties and simplifying the expression, we find:
lim (n→∞) (ln(1+1/n))/(ln(n)ln(1+n)) = 1/ln(3)
Since the limit is a finite non-zero value, both series have the same convergence behavior. Thus, the series ∑(n=3)∞ (ln(1+1/n))/(ln(n)ln(1+n)) is convergent, and its sum is 1/ln(3).
To know more about convergent click here: brainly.com/question/31756849
#SPJ11
Federal income tax rates are??

Answers
Answer:
Progressive
Step-by-step explanation:
Progressive tax rates increase as a person's income increases
is - 3/10 rational or irrational?
Answers
Answer:
rational
Step-by-step explanation:
Answer:
The fraction 3/10 is a rational number. All fractions are rational numbers.
A mountain bike originally priced at $921.00 is up for sale at a discount of 11%. What is the price of the mountain bike after the discount?
Answers
Answer:
Step-by-step explanation:
Original Cost Price (CP)= $921
Discount Rate (%)= 11 %
Discounted Money= Discount Rate* Cost Price=11%*$921=0.11*921= $ 101.31
Hence, Price after Discount= Original Cost Price- Discounted Money
= $921 - $101.31
= $ 819.69
Hence, The price of the mountain bike after the discount is $819.69.
How is each measurement represented using scientific notation? 48,000,000,000 g = 4.8 × 1010 g = 48 × 109 g = 48 × 10-10
Answers
The number 48,000,000,000 can be written in scientific notation as:
4.8 × 1010 or 48 × 109
Scientific notation is a way of expressing numbers in a concise form. It is typically used when dealing with very large or very small numbers. In scientific notation, a number is written as the product of two factors: a number between 1 and 10, and a power of 10.
For example, to express the number 48,000,000,000 in scientific notation we must first determine the two factors.
First factor: This is the number between 1 and 10. To determine this factor, we must count the number of places to the right of the decimal point (in this case, 0 places) and then move the decimal point that many places to the left.
48,000,000,000 → 4.8
Second factor: This is the power of 10. To determine this factor, we must count the number of places we moved the decimal point and then express this number as a power of 10.
In this example, we moved the decimal point 10 places to the left. Therefore, our power of 10 is 10.
Therefore, the number 48,000,000,000 can be written in scientific notation as:
4.8 × 1010 or 48 × 109
Learn more about Scientific notation here:
https://brainly.com/question/4953486
#SPJ4
Answer: A, A
Explanation:
48,000,000,000 g = 4.8 × 1010 g
0.000000655 mL = 6.55 × 10-7 mL
15.
Josh buys a new pair of shoes at Kohl's. The tax rate is an exorbitant 11%. He put the total bill of
$66.59 on his Visa card (the balance of which he will pay in full when it's due to avoid extra finance
charge!) What is the cost of the shoes before tax?
Answers
Answer:
$59.99
Step-by-step explanation:
11% of $59.99 is $6.60. $59.99+$6.60=66.59
Over a ten-year period, the average price of a gallon of milk increased from $1.13 per gallon to $1.63 per gallon. To the
nearest percent, how much did the price of a gallon of milk increase?
14%
B 31%
C) 44%
D 69%
Answers
Answer:
c
Step-by-step explanation: