Answers
Answer:
D) Reflection across a horizontal line
Answer:
d
Step-by-step explanation:
Related Questions
Question is in the pic

Answers
Answer:
y=-3x-1
Step-by-step explanation:
Give the equation of the circle that has a diameter with endpoints G(-6, — 3) and
R(-6,-8).
Answers
Generally, the equation of a circle is organized like this: \((x-h)^2+(y-k)^2=r^2\), where (h,k) is the centre and r is the radius.
Solving the QuestionWe're given the endpoints of the diameter: \((-6,-3)\) and \((-6,-8)\).
First, we can find the centre of the circle by finding the midpoint of these two endpoints.
\(midpoint=(\dfrac{x_1+x_2}{2},\dfrac{y_1+y_2}{2})\)
Plug in the points:
\(midpoint=(\dfrac{-6+-6}{2},\dfrac{-3+-8}{2})\\\\midpoint=(-6,\dfrac{-11}{2})\)
Therefore, the centre of the circle is \((-6,\dfrac{-11}{2})\). Plug this into the general equation:
\((x-h)^2+(y-k)^2=r^2\\\\(x-(-6))^2+(y-(-\dfrac{11}{2}))^2=r^2\\\\(x+6)^2+(y+\dfrac{11}{2})^2=r^2\)
To find the radius of the circle, we can calculate the distance between the centre and one of the given endpoints:
\(d=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2\)
Plug in the centre and one of the endpoints:
\(d=\sqrt{(-6-x_1)^2+(\dfrac{-11}{2}-y_1)^2}\\d=\sqrt{(-6-(-6))^2+(\dfrac{-11}{2}-(-3))^2}\\d=\sqrt{(-6+6)^2+(\dfrac{-11}{2}+3)^2}\\\\d=\sqrt{(0)^2+(-5.5+3)^2}\\\\d=\sqrt{(0)^2+(-2.5)^2}\\\\d=\sqrt{(0)^2+(-\dfrac{5}{2})^2}\\\\d=\sqrt{0+\dfrac{25}{4}}\\d=\sqrt{\dfrac{25}{4}}\\\\d=\dfrac{5}{2}\)
Therefore, the radius of the circle is \(\dfrac{5}{2}\). Plug this into the general equation:
\((x+6)^2+(y+\dfrac{11}{2})^2=r^2\\\\(x+6)^2+(y+\dfrac{11}{2})^2=(\dfrac{5}{2})^2\\\\(x+6)^2+(y+\dfrac{11}{2})^2=\dfrac{25}{4}\)
Answer\((x+6)^2+(y+\dfrac{11}{2})^2=\dfrac{25}{4}\)
Worth 25 points!
Simplify these equations with steps please.
√-48
√-63
√-72
√-24
√-84
√-99

Answers
Answer:
1) \(\sqrt{-48}=4\sqrt{3} \ i\)
2) \(\sqrt{-63}=3\sqrt{7} \ i\)
3) \(\sqrt{-72}=6\sqrt{2} \ i\)
4) \(\sqrt{-24}=4\sqrt{6} \ i\)
5) \(\sqrt{-84}=2\sqrt{21} \ i\)
6) \(\sqrt{-99}=3\sqrt{11} \ i\)
Step-by-step explanation:
We need to simply the following :
Note: For all questions given we know that \(\sqrt{-1}=i\) using it in all the given questions.
1) \(\sqrt{-48}\)
Factors of 48 are: 2x2x2x2x3
\(\sqrt{-48}\\=\sqrt{48}\sqrt{-1} \\=\sqrt{2\times2\times2\times2\times3}\ i\\= \sqrt{2^2\times2^2\times3}\ i\\=2\times2\sqrt{3} \ i \\=4\sqrt{3} \ i\)
2) \(\sqrt{-63}\)
Factors of 63 are: 3x3x7
\(\sqrt{-63}\\=\sqrt{63}\sqrt{-1} \\=\sqrt{3\times3\times7}\ i\\= \sqrt{3^2\times7}\ i\\=3\sqrt{7} \ i\)
3) \(\sqrt{-72}\)
Factors of 72 are: 2x2x2x3x3
\(\sqrt{-72}\\=\sqrt{72}\sqrt{-1} \\=\sqrt{2\times2\times2\times3\times3}\ i\\= \sqrt{2^2\times3^2\times2}\ i\\=2\times3\sqrt{2} \ i \\=6\sqrt{2} \ i\)
4) \(\sqrt{-24}\)
Factors of 24 are: 2x2x2x3
\(\sqrt{-24}\\=\sqrt{24}\sqrt{-1} \\=\sqrt{2\times2\times2\times3}\ i\\= \sqrt{2^2\times2\times3}\ i\\=2\sqrt{2\times3} \ i \\=2\sqrt{6} \ i\)
5) \(\sqrt{-84}\)
Factors of 84 are: 2x2x3x7
\(\sqrt{-84}\\=\sqrt{84}\sqrt{-1} \\=\sqrt{2\times2\times3\times7}\ i\\= \sqrt{2^2\times3\times7}\ i\\=2\sqrt{21} \ i\)
6) \(\sqrt{-99}\\\)
Prime factors of 99 are: 3x3x11
\(\sqrt{-99}\\=\sqrt{99}\sqrt{-1} \\=\sqrt{3\times3\times11}\ i\\= \sqrt{3^2\times11}\ i\\=3\sqrt{11} \ i \\=3\sqrt{11} \ i\)
Is there a way to solve this equation without Calculus concepts? I am enrolled in Geometry and this is extra credit and I'm having a hard time understanding this. Please mind how stupid I may sound if I said something wrong.

Answers
Step-by-step explanation:
ω = ω₀ + αt
θ = ω₀ t + ½ αt²
We need to eliminate t from the system of equations. Solve for t in the first equation, then substitute it into the second equation.
t = (ω − ω₀) / α
θ = ω₀ (ω − ω₀) / α + ½ α ((ω − ω₀) / α)²
θ = ω₀ (ω − ω₀) / α + ½ (ω − ω₀)² / α
αθ = ω₀ (ω − ω₀) + ½ (ω − ω₀)²
αθ = ω₀ω − ω₀² + ½ (ω² − 2ω₀ω + ω₀²)
αθ = ω₀ω − ω₀² + ½ ω² − ω₀ω + ½ ω₀²
αθ = ½ ω² − ½ ω₀²
2αθ = ω² − ω₀²
Which is an equation of a direct proportion?

Answers
Answer:
y=6x-6
Step-by-step explanation:
i think not sure tho
Answer:
y = 6x
Step-by-step explanation:
i just got it right on TTM
Which of these values for x make the value of the expression (x-5)(x+7) the greatest?
Answers
Which of these values for x make the value of the expression (x-5)(x+7) the greatest?
This is a quadratic equation (vertical parabola) open upward
the vertex is a minimum
the greatest value of this expression is for the greater value of x in absolute value
we have
that -9 is the greater value in absolute value
therefore
the answer is -9
Please help
Find the indicated side of the triangle
What is a= ?

Answers
Answer:
28\(\sqrt{2}\)
Step-by-step explanation:
The given is a special right triangle with angle measures : 45-45-90
The side lengths in this special triangle is follows as x\(\sqrt{2}\)-x\(\sqrt{2}\)-x
x represent the side length that sees the angle measure with 90 and it is given as 28 here so x\(\sqrt{2}\) or a in this case is equal to
28\(\sqrt{2}\)
Please help me solve the word problem!

Answers
Answer:
Step-by-step explanation:
Use the Pythagorean Theorem!
Ferris to Dunlap would be 35 mi
however, we do not know Ferris to Buttle
use the Pythagorean theorem
We get a squared + b squared is equal to c squared
-> 225 + 400 = 625
625 is equal to 25 * 25
so Ferris to Buttle would be 25.
and it is 15 mi shorter!
k
-4 -3 -2 -1
3
L
-1
-2
-3
-4
What is the slope of the line?
1 2
3
4
> X

Answers
Answer:
\(\frac{8}{5}\)
Step-by-step explanation:
Find two points on the line (-4,-4) and (1,4) If you start at (-4,-4) and go up vertically 8 spaces and then go right horizontally 5 spaces. You will end up at the point (1,4). This is our slope. The rise over the run. \(\frac{8}{5}\)
in an informal survey of students, 20 have dogs, 15 have cats, and five have both. one has a pet squirrel. how many people have a pet dog or a pet cat?
Answers
The people have a pet dog or a pet cat would be 30.
What is Union and Intersection of a numbers?
The set of elements that are contained in either set X, set Y, or both sets X and Y is referred to as the union of two sets X and Y. The set of items that are a part of both sets X and Y is known as the intersection of two sets X and Y.
Given that:
In survey of students,
20 have dogs, 15 have cats, and 5 have both. 1 has a pet squirrel.
n(A)=Student who has dogs.
n(B)=Student who has cats.
and n(AUB)= Student who having both dogs and cats.
So,
n(A)=20
n(B)=15
n(AUB)=5
According to formula,
n(AUB)=n(A)+n(B)-n(A∩B)
i.e. n(A∩B)=n(A)+n(B)-n(AUB)
n(A∩B)=20+15-5
n(A∩B)=30
Hence, The people have a pet dog or a pet cat would be 30.
To know more about Intersection visit,
https://brainly.com/question/29129216
#SPJ4
The record low temp in Fargo, ND is -37*F. The record high is 109*F. What is the difference in the record high and the record low temperatures?
Answers
Answer:
109 - -37 = 146
Step-by-step explanation:
since you are subtracting a negative, the negative number and the sign cancel each other out. So all you have to do is add.
In which of the following would you multiply both sides of the equation by n?
Solve m/n=p for m.
Solve m - n = p for m.
Solve mn = p for m.
Solve m + n = p for m.
Answers
Answer:
first question : solve for m: multiply both sides by n
m/n=p
m*(n)/n=p*n
m= p*n
Step-by-step explanation:
to solve for m in the first part, you have to get rid of the extras next to m. in this case because m is divided by n, if we multiply both sides by n, both n on the m side will cancel out n/n=1 . this will leave m*1= n*p
because we can ignore the coefficient 1, the solution of ot will give you m=n*p
this also answers the question that's asked in the first place. in the first question, you have to multiply both sides by n in order to get the answer.
Please fill in all of the blanks
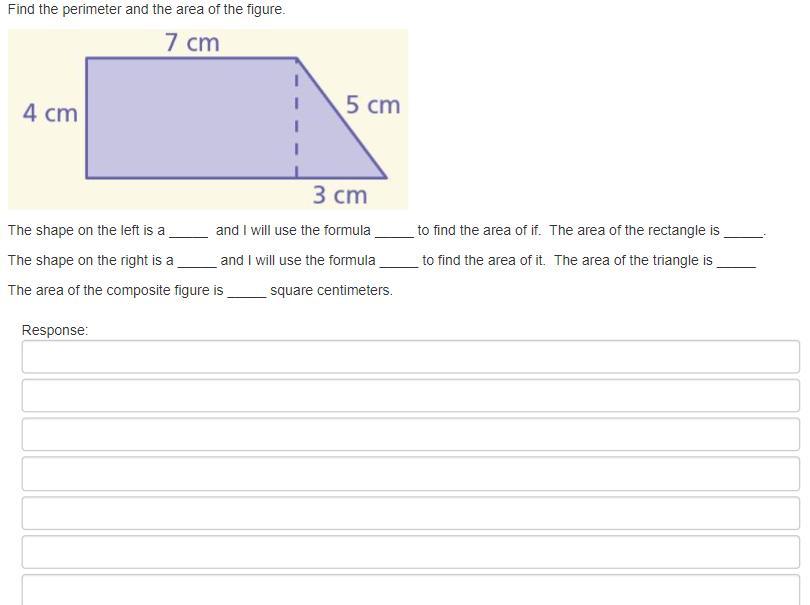
Answers
Answer:
The perimeter of this trapezoid is
7 + 5 + 3 + 7 + 4 = 26 cm
rectangle, A = lw, 4 × 7 = 28 square cm
triangle, A = (1/2)bh, (1/2) × 3 × 4 =
6 square cm
(1/2)(4)(7 + 10) = (1/2)(4)(17) = 34 square cm = 28 square cm + 6 square cm
question jasmine has 84 flowers available to make bouquets for the farmer's market. to make the bouquets more appealing to the eye, she would like to have an odd number of flowers in each. she wants to use all of the available flowers. to meet jasmines' requirements, how many flowers could she put in each bouquet? responses a 66 b 99 c 44 d 77
Answers
Answer:
b if she is allowed to use extra flowers, d if not, not sure im sorta guessing rn
a box with a square base and open top must have a volume of . we wish to find the dimensions of the box that minimize the amount of material used. first, find a formula for the surface area of the box in terms of only , the length of one side of the square base. simplify your formula as much as possible. next, find the derivative, .
Answers
The formula for the surface area of the box, in terms of the length of one side of the square base (s), is A = s^2 + 4s^2 = 5s^2.
The derivative of the surface area function with respect to s, denoted as dA/ds, gives us the rate of change of the surface area with respect to the length of one side of the base.
1. The surface area of the box consists of the area of the square base and the four equal sides. The area of the square base is s^2, and each side has a length of s. Therefore, the total surface area is given by A = s^2 + 4s^2 = 5s^2.
2. To find the derivative of the surface area function, we differentiate 5s^2 with respect to s using the power rule of differentiation. The power rule states that if we have a function f(x) = cx^n, then the derivative is f'(x) = cnx^(n-1).
Applying the power rule, we have dA/ds = d(5s^2)/ds = 10s.
3. The derivative, dA/ds = 10s, represents the rate of change of the surface area with respect to the length of one side of the square base. This means that for every unit increase in s, the surface area increases by 10s units.
The derivative does not provide information about minimizing the amount of material used. To find the dimensions of the box that minimize the amount of material used, we need to set up an optimization problem and solve for the critical points. This involves setting the derivative equal to zero and finding the values of s that satisfy this equation. However, since the problem statement does not provide a specific volume constraint or objective function, we cannot proceed with the optimization process.
To learn more about derivative, click here: brainly.com/question/23819325
#SPJ11
Write the interval as an inequality
[-5,5]
Answers
Answer:
\(-5\leq x\leq 5\)
Step-by-step explanation:
-5<x<5
both of the < need lines under them
Please Help! No Silly Answers Please! I would appreciate correct answers. Thank you all! Please try and include Scratch Work, Thank you.

Answers
Answer:
y in (7,y) = 14
Step-by-step explanation:
A General Linear function is as the following:
y = mx + c, Where: m: slope of the line, c: y-intercept
Given that:
Point 1 (P1) = (1,5)
Point 2 (P2) = (3, 11)
Point 3 (P3) = (7,y)
By substituting in the linear function with P1:
5 = m + c ---> (1)
By substituting in the linear function with P2:
11 = 3m + c ---> (2)
By multiplying equation (1) times 3 and subtracting eq. (2) from it:
(15 = 3m + 3c) - (11 = 3m + c) =
4 = 2c
Then, c = 2
By substituting with "c" in eq. (1):
5 = m + 2
Then, m = 3
Hence,
the function of this line is:
y = 3x + 2
So,
to get "y" in P3, we are going to substitute with 7:
y = 3(7) + 2
then "y" in P3 = 23
Another Solution:
by getting the slope of the line:
\(Slope = \frac{\triangle y}{\triangle x}\) Where: Δy: change in y points, Δx: change in x points
by utilizing P1 & P2 in slope formula:
\(slope = \frac{11 - 5}{3 - 1} = \frac{6}{2} = 3\)
By substituting with P1 in the linear function:
5 = 3 + c
Then, c = 2
the function of the line is:
y = 3x + 2
So,
to get "y" in P3, we are going to substitute with 7:
y = 3(7) + 2
then "y" in P3 = 23
hope you find this easy to understand....
Have any questions? Write in the comments.
give me brainliest if you found this answer useful
If L | | m and m<8 = 109°, what is m<5

Answers
Answer:
m∡5 = 71°
Step-by-step explanation:
m∡8 = m∡6
m∡6 + m∡5 = 180
180 - m∡6 = m∡5
180 - 109 = 71
Answer:
the measurement of angle 5 is 71
Step-by-step explanation:
m∡8 = m∡6
M∡6 + m∡5 = 180
180 - m∡6 = m∡5
180 - 109 = 71
Hope this helps :)
If if 1. 504 ÷ 4. 7 equals 0. 32 what is the value of 320 *
0. 7
Answers
The given information is that 1.504 ÷ 4.7 equals 0.32. We can use this information to solve for the value of 320 * 0.7.
Let's start by rearranging the given equation:
1.504 ÷ 4.7 = 0.32
Next, we can multiply both sides of the equation by 4.7 to isolate 1.504:
1.504 = 0.32 * 4.7
Simplifying the right side of the equation gives us:
1.504 = 1.504
This shows that the equation is balanced, meaning the values are equal. Therefore, we can conclude that the value of 320 * 0.7 is also 1.504.
Based on the given equation 1.504 ÷ 4.7 = 0.32, we can deduce that the value of 320 * 0.7 is 1.504.
To learn more about equation : brainly.com/question/29657983
#SPJ11
Im really struggling with C

Answers
The rate in meters per seconds at which the distance between John and Sam is changing is given as 33.33
How to solve for the rateFor C we are to find the rate at which the distance that exist between Jon and Sam is changing when the t time is given as 3 seconds
Given that Xs = 300 meters
Xs = 200 meters
t = 3 Seconds
We would have
The rate of change as Xs - Xt / t
= 300 - 200 / 3
= 100 / 3
= 33.33
d. The acceleration would be a positive value for Sam at t = 2. The implication that this would have is that she is speeding up.
Read more on rate here:https://brainly.com/question/119866
#SPJ1
15 divided by 0.3?
I know it’s easy but my Brains not working so
Answers
Hope this helps
Have a great day/night
Simplify the rational expression, if possible.
9y/y^2 − 81
I don't know what to do ;-;
Answers
Answer:
\(- \: \cfrac{ 81 {y}^{2} + 9 {}^{} }{y {}^{} } \)Step-by-step explanation:
I'm here for your assistance! :)
9y/y^2 − 81
\( \cfrac{ - 81 {y}^{2} + 9y {}^{} }{y {}^{2} } \)\( - \: \cfrac{ 81 {y}^{2} + 9 {}^{} }{y {}^{} } \)Hope I helped! :)
For the function f(x)=x4-2x2+3: ((a)) Determine the relative maximum point(s) of f. Answer: (XmYm )= (b)) Determine the relative minimum point(s) off.
Answers
The relative maximum point is (0, 3) and the relative minimum points are (-1, 2) and (1, 2).
To find the relative maximum and minimum points of the function f(x) = x^4 - 2x^2 + 3, we need to find the values of x where f'(x) = 0.
f'(x) = 4x^3 - 4x = 4x(x^2 - 1)
Setting f'(x) = 0, we get x = 0, ±1 as critical points.
To determine the nature of these critical points, we need to use the second derivative test.
f''(x) = 12x^2 - 4
At x = 0, f''(0) = -4 < 0, so this critical point is a relative maximum.
At x = 1, f''(1) = 8 > 0, so this critical point is a relative minimum.
At x = -1, f''(-1) = 8 > 0, so this critical point is also a relative minimum.
Therefore, the relative maximum point is (0, 3) and the relative minimum points are (-1, 2) and (1, 2).
To learn more about critical points, refer below:
https://brainly.com/question/29144288
#SPJ11
Construct a truth table for each of these compound propositions
a) p → ⇁p
b) p ↔ ⇁p
c) p ⊕ (p V q) d) (p ∧ q) → (p V q) e) (p → ⇁p) ↔ (p ↔ q) f) (p ↔ q) ⊕ (p ↔ ⇁q)
Answers
After considering the given data we conclude that there truth table is possible and is placed in the given figures concerning every sub question.
A truth table is a overview that projects the truth-value of one or more compound propositions for each possible combination of truth-values of the propositions starting up the compound ones.
Every row of the table represents a possible combination of truth-values for the component propositions of the compound, and the count of rows is described by the range of possible combinations.
For instance, if the compound has just two component propositions, it comprises four possibilities and then four rows to the table. The truth-value of the compound is projected on each row comprising the truth functional operator.
To learn more about truth table
https://brainly.com/question/28605215
#SPJ4



If y = 4 find slope, X-intercept and y-intercept.
Answers
Answer:
An equation in the form y = mx + b is in the 'slope y-intercept' form where m is the slope and b is the y-intercept. We can rewrite our equation, y = 4, in slope y-intercept form as follows: y = 0x + 4. Here, it is clear that the slope, or m, is zero. Therefore, the slope of the horizontal line y = 4 is zero
its all on the picture giving brainiest and 100 points its also math

Answers
#1
(1,2)(2,4)Slope=
\(\\ \rm\Rrightarrow m=4-2/2-1=2\)
Equation in point slope form
\(\\ \rm\Rrightarrow y-2=2(x-1)\)
\(\\ \rm\Rrightarrow y-2=2x-2\)
\(\\ \rm\Rrightarrow y=2x\)
Graph attached
#2
(1,3)(2,6)Same like before
Equation of the line:-
\(\\ \rm\Rrightarrow y=3x\)
Graph attached


Monica makes tomato sauce with the plants she grows in her garden. She uses 3 basil leaves in her sauce for every 8 tomatoes. She is making a big batch of sauce with 32 tomatoes from her garden.
How many basil leaves should Monica use in her sauce?
Answers
Answer:
12
Step-by-step explanation: has to do with ratios and proportionalities. 3/8 is going to equal b/32. B = to number of basil leaves. 32/8= 4. 4x3=12.
Answer:
Twelve.
Step-by-step explanation:
This scenario can be interpreted by the ratio 3:8, with 3 being the amount of basil leaves and 8 being the amount of tomatoes. If there were 32 tomatoes in the sauce, then there would have been 12 basil leaves.
3:8
6:16
9:24
12:32
All of these ratios can simplify back down to 3:8.
The admission for the train is $7 for adults and $4 for children. There are 4 children in Jan's family who are 2, 3, 7, and 9, and 3 adults. How much will it cost to ride the train?
Answers
Answer:
250 $3 tickets and 100 $2 tickets were sold.
37
Step-by-step explanation:
they're are three adults so multiply 3 by 7 which is 21
and 4 kids so multiply 4 by 4 to get 16
to get the total add 16 and 21 to get 26
Find the value of x and y from the following figure.

Answers
Answer:
X=70
Step-by-step explanation:
aw we can for a triangle, where the 2 angles are complementary from the outer ones, so the 2 angles are 50, and 60
so the third one will be 70
if the true percentages for the two treatments were 25% and 30%, respectively, what sample sizes (m
Answers
a. The test at the 5% significance level indicates no significant difference in the incidence rate of GI problems between those who consume olestra chips and the TG control treatment. b. To detect a difference between the true percentages of 15% and 20% with a probability of 0.90, a sample size of 29 individuals is necessary for each treatment group (m = n).
How to carry out hypothesis test?
To carry out the hypothesis test, we can use a two-sample proportion test. Let p₁ represent the proportion of individuals experiencing adverse GI events in the TG control group, and let p₂ represent the proportion in the olestra treatment group.
Null hypothesis (H₀): p₁ = p₂
Alternative hypothesis (H₁): p₁ ≠ p₂ (indicating a difference)
Given the data, we have:
n₁ = 529 (sample size of TG control group)
n₂ = 563 (sample size of olestra treatment group)
x₁ = 0.176 x 529 ≈ 93.304 (number of adverse events in TG control group)
x₂ = 0.158 x 563 ≈ 89.054 (number of adverse events in olestra treatment group)
The test statistic is calculated as:
z = (p₁ - p₂) / √((\(\hat{p}\)(1-\(\hat{p}\)) / n₁) + (\(\hat{p}\)(1-\(\hat{p}\)) / n₂))
where \(\hat{p}\) = (x₁ + x₂) / (n₁ + n₂)
b. We want to determine the sample size (m = n) necessary to detect a difference between the true percentages of 15% and 20% with a probability of 0.90.
Step 1: Define the given values:
p₁ = 0.15 (true proportion for the TG control treatment)
p₂ = 0.20 (true proportion for the olestra treatment)
Z₁-β = 1.28 (critical value corresponding to a power of 0.90)
Z₁-α/₂ = 1.96 (critical value corresponding to a significance level of 0.05)
Step 2: Substitute the values into the formula for sample size:
n = (Z₁-β + Z₁-α/₂)² * ((p₁ * (1 - p₁) / m) + (p₂ * (1 - p₂) / n)) / (p₁ - p₂)²
Step 3: Simplify the formula since m = n:
n = (Z₁-β + Z₁-α/₂)² * ((p₁ * (1 - p₁) + p₂ * (1 - p₂)) / n) / (p₁ - p₂)²
Step 4: Substitute the given values into the formula:
n = (1.28 + 1.96)² * ((0.15 * 0.85 + 0.20 * 0.80) / n) / (0.15 - 0.20)²
Step 5: Simplify the equation:
n = 3.24² * (0.1275 / n) / 0.0025
Step 6: Multiply and divide to isolate n:
n² = 3.24² * 0.1275 / 0.0025
Step 7: Solve for n by taking the square root:
n = √((3.24² * 0.1275) / 0.0025)
Step 8: Calculate the value of n using a calculator or by hand:
n ≈ √829.584
Step 9: Round the value of n to the nearest whole number since sample sizes must be integers:
n ≈ 28.8 ≈ 29
The complete question is:
Olestra is a fat substitute approved by the FDA for use in snack foods. Because there have been anecdotal reports of gastrointestinal problems associated with olestra consumption, a randomized, double-blind, placebo-controlled experiment was carried out to compare olestra potato chips to regular potato chips with respect to GI symptoms. Among 529 individuals in the TG control group, 17.6% experienced an adverse GI event, whereas among the 563 individuals in the olestra treatment group, 15.8% experienced such an event.
a. Carry out a test of hypotheses at the 5% significance level to decide whether the incidence rate of GI problems for those who consume olestra chips according to the experimental regimen differs from the incidence rate for the TG control treatment.
b. If the true percentages for the two treatments were 15% and 20% respectively, what sample sizes (m = n) would be necessary to detect such a difference with probability 0.90?
Learn more about hypothesis test on:
https://brainly.com/question/17099835
#SPJ4
